Schmidt decomposition - How do I find the matrix related to the state?

[Arquimedes]

TL;DR Summary

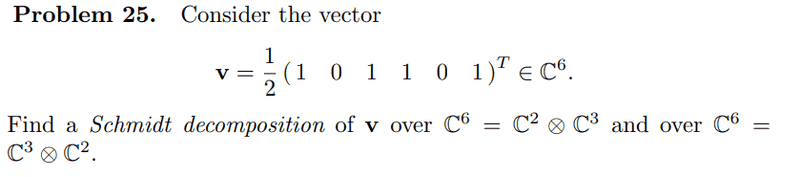
How to writte the matrix associated to the state, so I can apply the Singular Value Decomposition to that matrix and get the Schmidt coefficients.

Hello, I am currently studying the Schmidt decomposition and how to use it to determine if a state is entangled or not and I can't understand how to write the state as a matrix so I can apply the Singular Value Decomposition and find the Schmidt coefficients. The exercise I am trying to complete is this one:;

 

[Paul Colby]

A general mapping from $\mathbb{C}^2\otimes\mathbb{C}^3$ to $\mathbb{C}^6$ may be written,

$v_i = \sum_{k=1}^2 \sum_{m=1}^3 \alpha_{i k m} u_k w_m$

there are 36 unspecified constants, $\alpha_{i k m}$. So there are many possibilities. I think the problem statement is incomplete?