Series & Parallel capacitors in a circuit

[mitleid]

Four capacitors are connected as shown in the figure below (C = 16 mF)

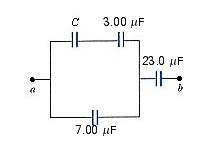
(a) Find the equivalent capacitance between points a and b.
(b) If V(ab) = 16.5 V, calculate the charge on the the 23.0 mF capacitor.

This is repeated for each capacitor in the circuit.

For the sake of simplicity, C = 16mF, C1 = 3 mF, C2 = 7 mF and C3 = 23 mF

I've correctly answered the first two parts of the problem. First C and C1 are considered in series and so the equivalent capacitance is found as:
1/C' = 1/C + 1/C1

C' and C2 are in parallel, so their equivalent capacitance C'' = C' + C2

Finally, C'' and C3 are in series, and the same equation as above is applied to get 6.74 mF.

To get the charge on C3, the value for total C (6.74) is used in the equation Q = CV to get 111 mC.

Solving for the other three capacitors has proved difficult... most likely I am making a similar mistake for both parts (since the charge for C and C1 are identical I only have two charges left to find).

To find the charge on C2 (7 mF), it seems I should be able to just multiply its capacitance by the voltage since it is in parallel (and so Q is directly proportional to each C).

Any advice?

 

[dynamicsolo]

Here's what you can look for when dealing with these capacitive networks. You know that plates joined by wire are equipotentials (since we assume this is magical "physics problem" wiring). It would also be the case that, before the voltage was applied across ab, those wires were electrically neutral. So the total charge on all plates joined by wire must add to zero.

This will tell you that Q = Q1 and that Q1 + Q2 = Q3. You can combine this with what you know about the potential "drops" along the parallel branches and across C3 (given that the total "drop" must be 16.5 V) to solve for all of the charges. (It takes a little work, but...)

I confirm your result for Q3.

 

[mitleid]

So adjacent plates must have complimentary charges? I can see that Q1 + Q2 = Q3, so likewise Q + Q2 = Q3... since these are parallel, correct? I am still running into some trouble with the calculation, though.

In general, Q = CV, but if I simply apply that I'm not getting the correct charges. My book says this applies only in parallel circuits where Q corresponds directly to C for each capacitor... *sigh* I'm not really getting this conceptually yet. I'll have to come back later, lab to finish!

 

[dynamicsolo]

So adjacent plates must have complimentary charges? I can see that Q1 + Q2 = Q3, so likewise Q + Q2 = Q3... since these are parallel, correct?

I'm not sure which ones you mean by "these are parallel". What I'm saying is that two plates connected by wire which started out as neutral will end up with equal and opposite charges after the voltage is applied. By the same token, multiple capacitors (such as C1, C2, and C3 here) sharing a common wiring will also divide charges among them. Since C1 and C2 are on parallel branches and their negative plates are both wiring to the positive plate of C3 (I'm taking point a to have the higher potential), then C1 and C2 will share the total charge found on C3.

Your approach to finding the charge on C3 is correct: the "total" charge, calculated by dividing the full "voltage drop" by the equivalent capacitance of the network, will be found on any capacitor on the "main line" connecting the points of the applied potential. So Q3 = (16.5 V)·(6.74 microF) = 111.2 microC .

From this, you can now find the voltage drop across C3, which will also tell you the voltage drop across the parallel branches. You can carry on from here and find all the remaining charges.

 

[mitleid]

... the "total" charge, calculated by dividing the full "voltage drop" by the equivalent capacitance of the network, will be found on any capacitor on the "main line" connecting the points of the applied potential. So Q3 = (16.5 V)·(6.74 microF) = 111.2 microC .

From this, you can now find the voltage drop across C3, which will also tell you the voltage drop across the parallel branches. You can carry on from here and find all the remaining charges.

This seems contradictory, unless I'm missing something. I thought charge is equal to C * V, not V/C.

Also, does each capacitor have a 'partial' potential drop across it? If so this would explain why something like... Q1 = 3 mF * 16.5 V doesn't work out.

I'm not certain how voltage drops work across parallel branches - i can't find anything in my notes.

At this point I've already solved the problem, but I'm still not comfortable with the it. The charge on C2 = 81.7, C1 = 29.3 and C = 29.3. C1 + C2 = 81.7 + 29.3 = 111 = C3. Using these numbers I solved for the "voltage drops" across each resister. For C2 I got 11.67 and for C1 I got 9.76 - but where do these numbers come from? Apparently I don't know anything about voltage drops through parallel branches... could use the last bit of help on this one.

 

[mitleid]

really all I need to know is how the voltage across C3 helps me find the other partial voltages... man this is probably painfully obvious.

 

[dynamicsolo]

This seems contradictory, unless I'm missing something. I thought charge is equal to C * V, not V/C.

Yes, Q = CV; is that not what you're seeing? (I edited the post because of something I mistyped and I sometimes wonder if other readers are seeing what I had originally, or what I revised it to.)

Also, does each capacitor have a 'partial' potential drop across it? If so this would explain why something like... Q1 = 3 mF * 16.5 V doesn't work out.

Yes: see below.

I'm not certain how voltage drops work across parallel branches - i can't find anything in my notes.

Points joined by wire only are considered to all be at the same voltage. If b is the "negative" end (lower potential), then the positive plate of C3 is at the same potential as the negative plates of C1 and C2; the positive plates of C and C2 are at the same potential. The "drop" across the C and C1 branch is the same as the "drop" across the C2 branch.

Have you discussed in class methods like setting the lower potential end (b) to zero, which would put the higher end (a) to 16.5 V, and solving for the other intermediate voltages relative to zero? (We would still need to find the voltage between C and C1 and on the wire connecting C1, C2, and C3.)

At this point I've already solved the problem, but I'm still not comfortable with the it. The charge on C2 = 81.7, C1 = 29.3 and C = 29.3. C1 + C2 = 81.7 + 29.3 = 111 = C3. Using these numbers I solved for the "voltage drops" across each resister. For C2 I got 11.67 and for C1 I got 9.76 - but where do these numbers come from? Apparently I don't know anything about voltage drops through parallel branches... could use the last bit of help on this one.

That looks good. Here's the various voltages then:

We'll set the potential at b to zero, making the potential at a +16.5 V.

The potential drop across C3 is then
delta_V = Q3/C3 = (111.2 microC)/(23 microF) = 4.83 V.
So the potential at the positive plate of C3 and the negative plates of C1 and C2 is +4.83 V.

This means that the potential drop across the parallel branches is
+16.5 - (+4.83) V = 11.67 V.
This either jibes with or is how you find Q2:
Q2 = C3 · V3 = (7 microF)·(11.67 V) = 81.66 microC .

The charge on both C and C1 must be the same, since they are on the same parallel branch and thus the negative plate of C is joined to the positive plate of C1. The charge must be Q = Q1 = Q3 - Q2 = 111.2 - 81.66 microC = 29.54 microC .

We now have all the charges we were looking for, but just to confirm everything, we'll also find the potential drops across each capacitor. For C, this is
delta_V = Q / C = (29.54 microC)/(16 microF) = 1.85 V .
For C1, delta_V = Q1/C1 = (29.54 microC)/(3 microF) = 9.85 V.

The potential at a point between C and C1 will be either 1.85 V down from b or +16.5 - 1.85 V = +14.65 V , or 9.85 V up from the negative plate of C1, which is +4.83 + 9.85 = +14.68 V (close enough for jazz... I didn't round numbers the same way each time, I guess).

There are enough independent checks of the voltages at various points in the circuit to have reasonable confidence in our answers.

 

[mitleid]

Thanks very much - this certainly tied things together !

I was browsing through other posts on capacitance, and this one shows some of the differences in calculating the charge based on whether the capacitors are in "series" or in "parallel". I understand the differences.. it seems a little complicated though, the way they are doing it in comparison to how you spelled it out. This is much more straight-forward and is also how I solved some of the other problems on my assignment.

https://www.physicsforums.com/showthread.php?t=189245

Actually my professor has described that, but in terms of two arbitrary forms connected by a wire with induced delta_V between them. He described how one form has charge +Q, the other -Q after all the energy has been transferred. Starting at 0, the first infinitesimal point charge dQ (or just q) is transferred from one to the other, which induces a -q on the other form. This process continues until +Q and -Q is achieved.

So he did describe this, but not in terms of the circuit (or from one point to another). He's a weird guy, likes to build up the concepts from a fairly abstract point of view. It takes me a little while to really get a hold of the subjects in this class, but it's cool. I got an A on my first exam!

Honestly, I have physicsforums to thank for that, you guys are awesome. Thanks very much for the help.

 

[jhess12]

thanks mitleid and dynamicsolo, this helped me out a lot

 

[dynamicsolo]

Thanks very much - this certainly tied things together !

I was browsing through other posts on capacitance, and this one shows some of the differences in calculating the charge based on whether the capacitors are in "series" or in "parallel". I understand the differences.. it seems a little complicated though, the way they are doing it in comparison to how you spelled it out. This is much more straight-forward and is also how I solved some of the other problems on my assignment.

https://www.physicsforums.com/showthread.php?t=189245

I should mention that electrical engineers have developed a variety of methods for doing circuit analysis which are very efficient but not always straightforward (it takes a bit of derivation to understand why many of their techniques work and give correct results.) There are several ways that circuit analysis is discussed in physics texts also, but I find some of them a bit obscure. (I prefer to look at what the charge carriers, currents and fields are doing, as it makes it clearer what the formulas mean.)

Actually my professor has described that, but in terms of two arbitrary forms connected by a wire with induced delta_V between them. He described how one form has charge +Q, the other -Q after all the energy has been transferred. Starting at 0, the first infinitesimal point charge dQ (or just q) is transferred from one to the other, which induces a -q on the other form. This process continues until +Q and -Q is achieved.

So he did describe this, but not in terms of the circuit (or from one point to another). He's a weird guy, likes to build up the concepts from a fairly abstract point of view. It takes me a little while to really get a hold of the subjects in this class, but it's cool.

I think what he's setting up is the method for how you calculate the equilibrium electric field and electric potential energy of the charged capacitor. The electrical and electronic devices have to be analyzed differentially because their present behaviors are functions of their present levels of charge.

I got an A on my first exam!

Congratulations! On to the next one...

 

[dynamicsolo]

thanks mitleid and dynamicsolo, this helped me out a lot

I'm glad to see that students are browsing the problem-solving threads -- not every one is a success, but you can pick up a lot of thoughts about the topics and ideas for approaching problems by looking around here...