Shear flow in thin-walled members -- Hibbeler confusion

[arestes]

TL;DR Summary

Hibbeler computes moment of inertia of thin-walled member with exact dimensions. In more recent edition, it uses "centerline" dimensions. Results are close to each other but which one is "more" right?

Hello:
I was reading about thin-walled members under shear force, specifically example 7-7 of Hibbeler's Engineering Mechanics, Mechanics of Materials.
First, the fourth Edition:

As you can see above. He starts by computing the moment of inertia on the first equation by subtracting a rectangle to another rectangle (the hole). This is clear.

However, the most recent edition I have (Ninth edition) computes the moment of inertia (again, same problem, same data) on the first equation:

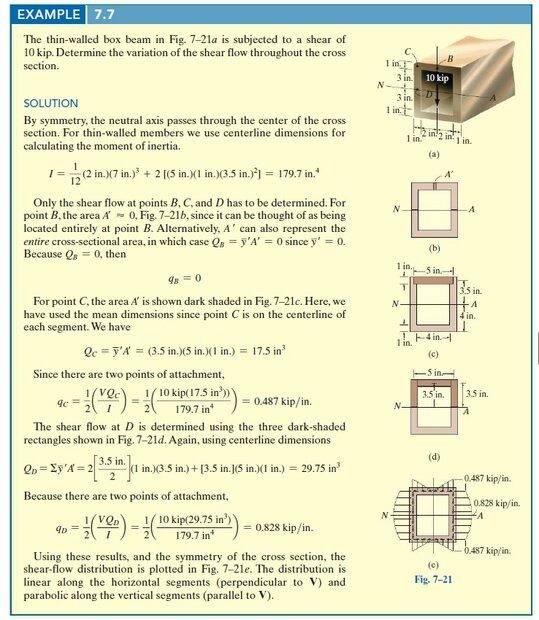
Here Hibbeler uses "centerline" dimensions (which, I guess it's just an approximation). I understand that both computations are close. I could argue that one is just a convenient approximation. However, I read some parts of the theory about shear flow. For example, shear stress due to torsion needs centerline area (see the chapter on torsion on this same book (thin-walled tubes having closed cross sections) and the concept of "mean area" appears. This makes me believe there's something going on with mean or centerline dimensions. After all, isn't all this based on approximations of continuum mechanics theories?

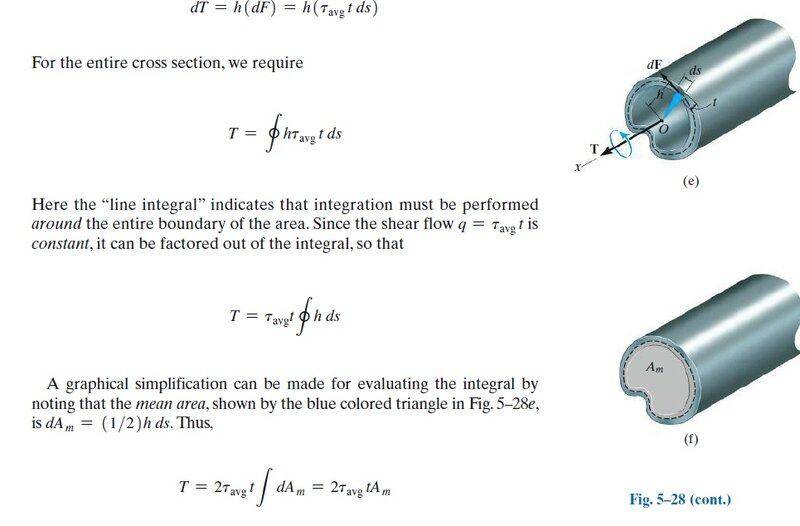
If the only difference in taking centerline dimensions and exact moment of inertia is just a matter of convenience, I'm puzzled as to why Hibbeler decided to change his first exact calculation to an approximate one, given that the exact computation was fairly straightforward.
I'm interested in getting more info about this kind of calculations. Any comments and insights would be appreciated. Thanks

 

[onatirec]

I'm puzzled as to why Hibbeler decided to change his first exact calculation to an approximate one, given that the exact computation was fairly straightforward.

The first calculation is also an approximation from continuum mechanics. One of the larger assumptions being that the shear stress is constant over the width of a member. So, the "regular" shear stress formula is an average value assuming uniform stress distribution. This assumption quickly falls apart as the width/height ratio of a member increases, e.g. in thin-walled members.

The thin-walled approach explicitly ignores thickness terms above 2nd order in it's derivation/simplification. It also ignores shear flow perpendicular to the thin-walled members.

It would be interesting to compare both approaches to the theory of elasticity, but I don't have much insight into the magnitudes of deviations from deeper theory.. Maybe a continuum mechanics expert could chime in.