SHM r/R Ratio Question: Solving for Simple Harmonic Motion in a Fixed Trough"

[ehild]

Yes... you are right...the equation i have written says the same
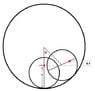
distance moved by CM =(R-r)θ
distance moved by point on the hoop =αr

I am assuming the hoop rolls without slipping ...

Yes, it is rolling without slipping.

ehild

 

[Saitama]

Yes :)

ehild

Continuing from my previous equation:

$$U=2mgR(1-\cos \theta)+2mgr\cos \theta+mgr\cos((\frac{R}{r}-1)\theta)$$
If I put θ=0, I get U=3mgr which verifies that the equation is correct.

What should be my next step?

 

[ehild]

What happens if theta changes?

What is the characteristics of the potential function in case of SHM?

ehild

 

[Saitama]

What is the characteristics of the potential function in case of SHM?

Minimum at the mean position.

 

[ehild]

How do you know if a function has minimum?

ehild

 

[Saitama]

How do you know if a function has minimum?

ehild

By differentiating the given function.

 

[ehild]

And?

It is U(theta). Differentiate.

ehild

 

[Saitama]

And?

And set the derivative equal to zero.

$$\frac{dU}{d\theta}=2mgR\sin(\theta)-2mgr\sin(\theta)-mgr\sin((\frac{R}{r}-1)\theta)(\frac{R}{r}-1)=0$$
Simplifying, I end up with this:
$$2\sin(\theta)=\sin((\frac{R}{r}-1)\theta)$$

How should I proceed from here?

 

[ehild]

dU/dθ =0 at θ=0?

ehild

 

[Saitama]

dU/dθ =0 at θ=0?

ehild

Yes, its zero at θ=0.

 

[ehild]

Is is maximum, minimum, saddle?

ehild

 

[Saitama]

Is is maximum, minimum, saddle?

ehild

I guess I have reached the answer. It should be minimum so that SHM takes place. For this, the second derivative is greater than zero at θ=0.
$$\frac{d^2U}{d\theta^2}=2mgR \cos(\theta)-2mgr \cos(\theta)-mgr \cos((\frac{R}{r}-1})\theta)(\frac{R}{r}-1})^2$$
(Why the above code is not shown properly? )
At θ=0
$$\frac{d^2U}{d\theta^2}=2mgR-2mgr-mg\frac{(R-r)^2}{r}>0$$
$$mg(R-r)\frac{(3r-R)}{r}>0$$
Hence,
$$\frac{r}{R}>\frac{1}{3}$$
The least r/R ratio is thus 1/3.

 

[ehild]

I guess I have reached the answer. It should be minimum so that SHM takes place. For this, the second derivative is greater than zero at θ=0.
$$\frac{d^2U}{d\theta^2}=2mgR \cos(\theta)-2mgr \cos(\theta)-mgr \cos((\frac{R}{r}-1)\theta)(\frac{R}{r}-1)^2$$
(Why the above code is not shown properly? )
At θ=0
$$\frac{d^2U}{d\theta^2}=2mgR-2mgr-mg\frac{(R-r)^2}{r}>0$$
$$mg(R-r)\frac{(3r-R)}{r}>0$$
Hence,
$$\frac{r}{R}>\frac{1}{3}$$
The least r/R ratio is thus 1/3.

Well done!

ehild

 

[Saitama]

Well done!

ehild

Thanks ehild for the help!

Btw, is there any trick to do this question? This question is from a test paper and the solution says that for stable equilibrium, the condition is
$$\frac{1}{h}>\frac{1}{r}-\frac{1}{R}$$
How did they arrived at this relation?

 

[ehild]

What is h?

ehild

 

[Saitama]

What is h?

ehild

The solution says that h is the height of centre of mass of ring particle system.

 

[ehild]

The position is stable if the CM gets higher when the system is displaced. I do not see at the moment how that relation comes out.

ehild

 

[TSny]

Btw, is there any trick to do this question? This question is from a test paper and the solution says that for stable equilibrium, the condition is
$$\frac{1}{h}>\frac{1}{r}-\frac{1}{R}$$
How did they arrived at this relation?

Use the same type of geometry you have already used to show that the change in height of the CM may be expressed as

$\Delta h = (R-r)(1-cos\theta) - (h-r)[1-cos(\frac{R-r}{r}\theta)]$

Use small angle approximation $cos\beta = 1-\beta^2/2$ to simplify the condition $\Delta h >0$ for small displacement of the hoop.

 

[Saitama]

Use the same type of geometry you have already used to show that the change in height of the CM may be expressed as

$\Delta h = (R-r)(1-cos\theta) - (h-r)[1-cos(\frac{R-r}{r}\theta)]$

Use small angle approximation $cos\beta = 1-\beta^2/2$ to simplify the condition $\Delta h >0$ for small displacement of the hoop.

Thanks TSny, I did reach the relation now but how can I find the least ration using the relation?

 

[ehild]

What is h in terms of r?

ehild

 

[Saitama]

What is h in terms of r?

ehild

Oops, totally missed it, I was so much involved in deriving that equation of TSny that I forgot to use the value of h.

Thanks once again both of you.