chwala
Gold Member
- 2,827
- 415
- Homework Statement
- Pack A consists of ##10## cards numbered ##0,0,1,1,1,1,1,3,3,3##. Pack B consists of ##6## cards numbered ##0,0,2,2,2,2##. One card is chosen at random from each pack. The random variable ##X##is defined as the sum on the two cards.
Show that ##P(X=2)=\dfrac{2}{15}##
- Relevant Equations
- Probability
My approach;
##P(X=2)=\dfrac{8}{60}=\dfrac{4}{30}=\dfrac{2}{15}##
Mark scheme solution;
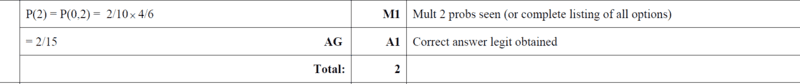
just sharing...cheers! Any insight is welcome.
| 0 | 0 | 1 | 1 | 1 | 1 | 1 | 3 | 3 | 3 | |
| 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 3 | 3 | 3 |
| 0 | 0 | 0 | 1 | 1 | 1 | 1 | 1 | 3 | 3 | 3 |
| 2 | 2 | 2 | 3 | 3 | 3 | 3 | 3 | 5 | 5 | 5 |
| 2 | 2 | 2 | 3 | 3 | 3 | 3 | 3 | 5 | 5 | 5 |
| 2 | 2 | 2 | 3 | 3 | 3 | 3 | 3 | 5 | 5 | 5 |
| 2 | 2 | 2 | 3 | 3 | 3 | 3 | 3 | 5 | 5 | 5 |
##P(X=2)=\dfrac{8}{60}=\dfrac{4}{30}=\dfrac{2}{15}##
Mark scheme solution;
just sharing...cheers! Any insight is welcome.