MHB Show that X satisfies the equation

[mathlearn]

View attachment 5923

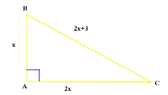
Solve that x satisfies the equation $x^2-12x-9$=$0$

Solve the above equation ($\sqrt{5}=2.24$)

Any Ideas on how to begin ? (Crying)

 

[I like Serena]

Solve that x satisfies the equation $x^2-12x-9$=$0$

Solve the above equation ($\sqrt{5}=2.24$)

Any Ideas on how to begin ? (Crying)

Hey mathlearn! ;)

Let's start with the Pythagorean theorem.
In a right triangle, we have:
$$a^2+b^2=c^2$$

What would it look like if we replace $a,b,c$ by the respective formulas? (Wondering)

 

[mathlearn]

Hey mathlearn! ;)

Let's start with the Pythagorean theorem.
In a right triangle, we have:
$$a^2+b^2=c^2$$

What would it look like if we replace $a,b,c$ by the respective formulas? (Wondering)

OK ,Using the pythagorean theorem It would look like this (Happy)
$2x^2+x^2 = (2x+3)^2$
$4x^2+x^2 = (4x^2+9)$

 

[I like Serena]

OK ,Using the pythagorean theorem It would look like this (Happy)
$2x^2+x^2 = (2x+3)^2$
$4x^2+x^2 = (4x^2+9)$

Let's put a couple of parentheses into make sure we evaluate everything in the correct order:
$$(2x)^2+(x)^2 = (2x+3)^2$$

Now if we want to evaluate something like $(a+b)^2$, it works out like this:
$$(a+b)(a+b) = a(a+b) + b(a+b) = a^2 + ab + ba + b^2 = a^2 + 2ab + b^2$$
Or for short:
$$(a+b)^2 = a^2 + 2ab + b^2$$

That means:
$$(2x+3)^2 = (2x)^2 + 2(2x)(3) + (3)^2 = 4x^2 + 12x + 9$$

 

[mathlearn]

(Nod) True!

Solve that x satisfies the equation $x^2-12x-9$=$0$

Solve the above equation ($\sqrt{5}=2.24$)

Any Ideas on how to begin ? (Crying)

That means:
$$(2x+3)^2 = (2x)^2 + 2(2x)(3) + (3)^2 = 4x^2 + 12x + 9$$

$$(2x+3)^2 = 4x^2 + 12x + 9 $$

Now to satisfy $x^2-12x-9$=$0$. (Happy)

 

[HallsofIvy]

Have you forgotten what the original problem was?

Previously, using the Pythagorean theorem, you correctly said that
$x^2+ (2x)^2= (2x+ 3)^2$ but incorrectly expanded the right side.

Now, knowing that $(2x+ 3)^2= 4x^2+ 12x+ 9$, your equation becomes
$x^2+ 4x^2= 4x^2+ 12x+ 9$. Simplify that.

 

[I like Serena]

(Nod) True!

$$(2x+3)^2 = 4x^2 + 12x + 9 $$

Now to satisfy $x^2-12x-9$=$0$. (Happy)

We have:
\begin{align}(2x)^2 + (x)^2 = (2x+3)^2
&\quad\Rightarrow\quad 4x^2 + x^2 = 4x^2 + 12x + 9 \\
&\quad\Rightarrow\quad 4x^2 + x^2 - 4x^2 - 12x - 9 = 0 \\
&\quad\Rightarrow\quad x^2 - 12x - 9 = 0
\end{align}

 

[mathlearn]

(Nod) $ x^2 - 12x - 9 = 0 $

Now $x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}$.

$x=\frac{12\pm\sqrt{180}}{2}$.

$x=\frac{12\pm6\sqrt{5}}{2}$.

$x=\frac{25.44}{2}$.

$x=12.72$., Correct ?(Thinking)

 

[HallsofIvy]

Yes, but that has nothing at all to do with the original problem! You were not asked to solve the equation.

 

[mathlearn]

(Yes)(Wink)(Smile) Thank you very much both of you. I Like Serena & hallsofivy

 

[I like Serena]

Yes, but that has nothing at all to do with the original problem! You were not asked to solve the equation.

Erm... the OP says:

Solve the above equation ($\sqrt{5}=2.24$)