Show the proof by induction in the given problem

[chwala]

Homework Statement

See attached

Relevant Equations

Induction

My interest is solely on the highlighted part in red...hmmmmmmm taken a bit of my time to figure that out...but i got it. Looking for any other way of looking at it;

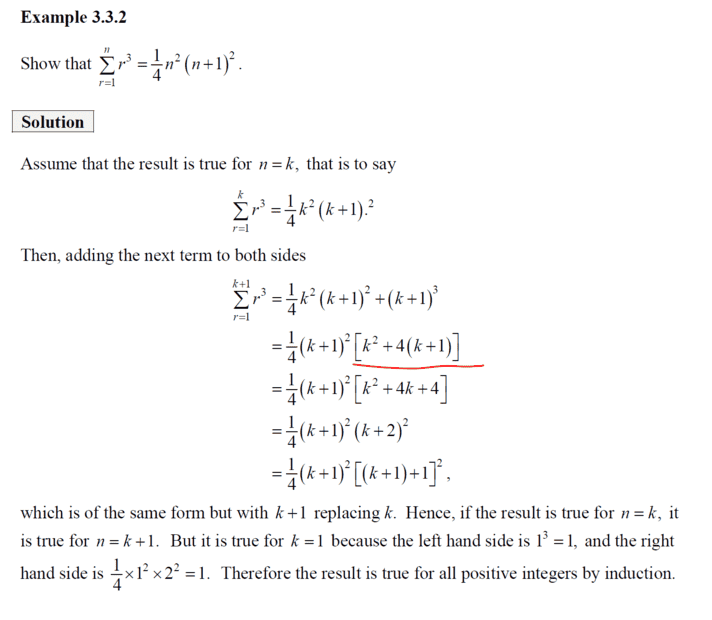
I just realised that the next term would be given by;

$\dfrac{1}{4}(k+1)^2(k+2)^2-\dfrac{1}{4}k^2(k+1)^2$

$=\dfrac{1}{4}(k+1)^2\left[(k+2)^2-k^2\right]$

$=\dfrac{1}{4}(k+1)^2\left[k^2+4k+4-k^2\right]$

$=\dfrac{1}{4}(k+1)^2\left[4k+4\right]$

Therefore adding the $k$ to $k+1$ yields;

$\dfrac{1}{4}k^2(k+1)^2+ \dfrac{1}{4}(k+1)^2\left[4k+4\right]$

$=\dfrac{1}{4}(k+1)^2\left[k^2+4k+4\right]$

Then the rest of the step will follow...this was the only challenging part...any other way of looking at it would be nice. Cheers

Question
If i may ask, how did they get the $(k+1)^3$ on the first line?

 

[Office_Shredder]

The line in red is just factoring $\frac{1}{4}(k+1)^2$. The left term leaves a $k^2$, the right term leaves a $4(k+1)$ which is less visually obvious because factoring fractions is tricky. You can avoid needing to notice clever manipulations. You can just totally expand the expression into a polynomial, and then take your target expression and expand it into a polynomial, and confirm the coefficients line up.

 

[malawi_glenn]

Question
If i may ask, how did they get the $(k+1)^3$ on the first line?

Where on the first line?

 

[chwala]

Where on the first line?

On this line; $\sum_{r=1}^{k+1} r^3= \dfrac{1}{4}(k+1)^2 +(k+1)^3$

 

[malawi_glenn]

On this line; $\sum_{r=1}^{k+1} r^3= \dfrac{1}{4}(k+1)^2 +(k+1)^3$

Hint, what can $\displaystyle \sum_{r=1}^{k} r^3 + (k+1)^3$ be written as?

what do you think "adding the next term" mean?

 

[chwala]

Hint, what can $\displaystyle \sum_{r=1}^{k} r^3 + (k+1)^3$ be written as?
View attachment 319251
what do you think "adding the next term" mean?

Seen it...you can check post $1$ and see that i know what 'the next term is'...therefore i will just go ahead and add it to previous term as follows;

$\dfrac{1}{4}k^2(k+1)^2+\dfrac{1}{4}(k+1)^2\left[4k+4\right]$

$\dfrac{1}{4}k^2(k+1)^2+4\left[\dfrac{1}{4}(k+1)^2(k+1)\right]$

$=\dfrac{1}{4}k^2(k+1)^2+(k+1)^2 (k+1)$

$\dfrac{1}{4}k^2(k+1)^2+(k+1)^3$

Cheers man!

 

[malawi_glenn]

Cheers man!

It means this
$\displaystyle \sum_{r=1}^{k} r^3 + (k+1)^3 = 1 + 2^3 + 3^3 + \cdots + (k-1)^3 + k^3 + (k+1)^3 = \sum_{r=1}^{k+1} r^3$.
Thus, we can write
$\displaystyle \sum_{r=1}^{k+1} r^3 = \sum_{r=1}^{k } r^3 + (k+1)^3 = \dfrac{1}{4}k^2(k+1)^2 + (k+1)^3$
where we used the induction hypothesis in the last step.