Showing piece-wise function continuous

Click For Summary

Homework Help Overview

The discussion revolves around the continuity of a piecewise function, specifically examining the function defined as \( f(x) = \cos x \) on the interval \([\frac{\pi}{4}, \infty)\) and its behavior around the point \(\frac{\pi}{4}\).

Discussion Character

  • Conceptual clarification, Assumption checking

Approaches and Questions Raised

  • Participants explore whether certain details, such as explicitly stating the function on specific intervals, are necessary for clarity. There is also a discussion about the importance of including or omitting certain points when discussing continuity.

Discussion Status

The conversation is ongoing, with participants providing feedback on the clarity of statements made in the context of the function's continuity. Some guidance has been offered regarding the inclusion of specific points in the discussion.

Contextual Notes

There is a focus on the continuity of the function across specified intervals, with particular attention to the implications of including or excluding the point \(\pi/4\) in the analysis.

member 731016
Homework Statement
Please see below
Relevant Equations
Please see below
For this,
1680662250322.png
,
The solution is,
1680662269391.png

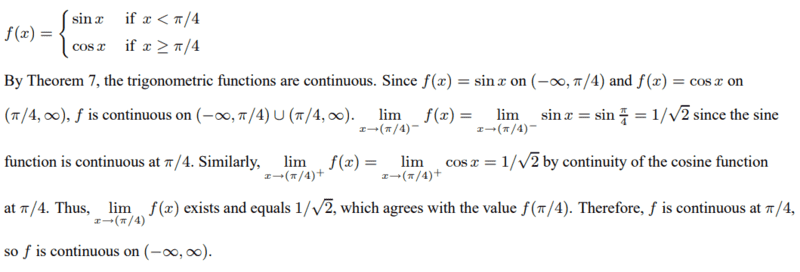
However, should they not write ##f(x) = \cos x## on ##[\frac{pi}{4}, \infty)##

Many thanks!
 
Last edited by a moderator:
Physics news on Phys.org
Do you mean right after the "Similarly,"? It wouldn't hurt, but I think that it is easy enough to follow the logic without saying that. Initially, you should be in the habit of stating everything. After a while, that becomes tedious and both you and the reader will be happy if you skip obvious things. You must be careful though, what you skip.
 
Reply
  • Like
Likes   Reactions: member 731016
FactChecker said:
Do you mean right after the "Similarly,"? It wouldn't hurt, but I think that it is easy enough to follow the logic without saying that. Initially, you should be in the habit of stating everything. After a while, that becomes tedious and both you and the reader will be happy if you skip obvious things. You must be careful though, what you skip.
Thank you for you reply @FactChecker!

No sorry I meant right after the "Since f(x) = Sinx on ..."

Many thanks!
 
ChiralSuperfields said:
Thank you for you reply @FactChecker!

No sorry I meant right after the "Since f(x) = Sinx on ..."

Many thanks!
Oh. The reason for not including the point ##\pi/4## is that the rest of the sentence is only about continuity on ##(-\infty, \pi/4)\cup(\pi/4, \infty)##. So there was no need to include ##\pi/4##. It wouldn't have hurt to include it.
 
Reply
  • Like
Likes   Reactions: member 731016

Similar threads

Uniform Continuity of functions
  • · Replies 40 ·
2
Replies
40
Views
5K
Show function series involving arctan is not differentiable at x=0
  • · Replies 26 ·
Replies
26
Views
2K
Converting a Piecewise function to a Heaviside function
  • · Replies 10 ·
Replies
10
Views
3K
How to calculate primitive functions on maximal intervals for periodic functions?
  • · Replies 4 ·
Replies
4
Views
2K
Can this function still be a constant function?
  • · Replies 11 ·
Replies
11
Views
2K
How should I show the following by using the signum function?
  • · Replies 14 ·
Replies
14
Views
2K
Finding A Constant To A Piece-Wise Function
  • · Replies 4 ·
Replies
4
Views
2K
MHB How Can We Approximate a Continuous Function with a Piece-Wise Linear Function?
  • · Replies 7 ·
Replies
7
Views
2K
Proving piecewise function is k-differentiable
  • · Replies 5 ·
Replies
5
Views
2K
Continuity With Piece Wise Functions
  • · Replies 1 ·
Replies
1
Views
1K