Jason-Li
- 119
- 14
- Homework Statement
- (a) Determine the voltage required on the input of the amplifier to give
the maximum output of 85 dBμV.
(b) If the signal level from the aerial is 5 dBmV and the input noise level
is 20 dBμV, calculate the signal-to-noise ratio on the output of the
amplifier.
Values:
Bandwidth 40–862 MHz
Gain 20 dB
Noise Figure 6 dB
Max. Output 85 dBV
Input Impedance 75Ω
Output Impedance 75Ω
- Relevant Equations
- SNR=20Log(Vs/Vn)
SNR=10Log(Ps/Pn)
So pretty confident I understand part (a) however for part (b) I'm not sure if I have carried it out correctly if someone could give me a pointer?
(b)
5dBmV Input as a voltage:
5=20Log(V/1mV)
V=105/10
V=1.77827941mV
Then the noise level is 20dbμV so changing to a voltage:
20=20*Log(V/1μV)
V=1020/20
V=10μV
Then I can find the SNR of the input using:
SNRIn=20*Log(Vp/Vn)
SNRIn=20*Log((1.77827941*10-3)/(10*10-6))
SNRIn=45dB
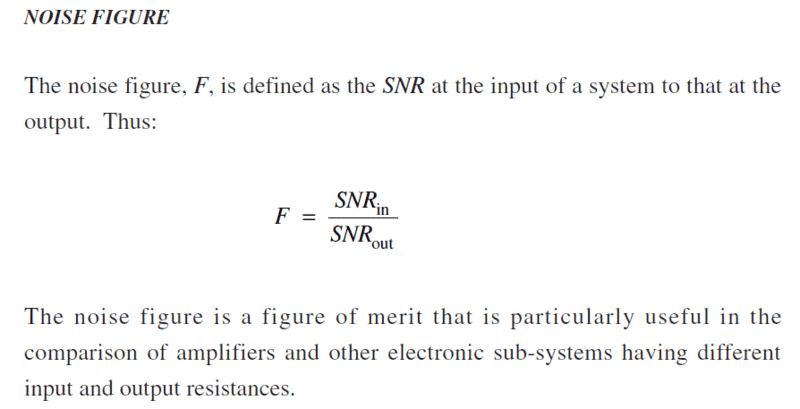
Then as I have the Noise figure from the data as F=6dB I can do the following:
F=SNRIn/SNROut
SNROut=SNRIn/F
SNROut=45*6
SNROut=270dB
This seems far too high? I have found websites that say the formula is actually F = SNRIn-SNROut however the learning materials say:
(b)
5dBmV Input as a voltage:
5=20Log(V/1mV)
V=105/10
V=1.77827941mV
Then the noise level is 20dbμV so changing to a voltage:
20=20*Log(V/1μV)
V=1020/20
V=10μV
Then I can find the SNR of the input using:
SNRIn=20*Log(Vp/Vn)
SNRIn=20*Log((1.77827941*10-3)/(10*10-6))
SNRIn=45dB
Then as I have the Noise figure from the data as F=6dB I can do the following:
F=SNRIn/SNROut
SNROut=SNRIn/F
SNROut=45*6
SNROut=270dB
This seems far too high? I have found websites that say the formula is actually F = SNRIn-SNROut however the learning materials say: