- #1
paralleltransport
- 131
- 97
Hello.
I wanted to construct a simple and clear explanation for the relation between the beta function and the critical exponents (divergence of correlation length) close to a critical point.
I wanted to check if this reasoning is valid. This is my own rewording of complicated arguments I see in standard QFT texts.
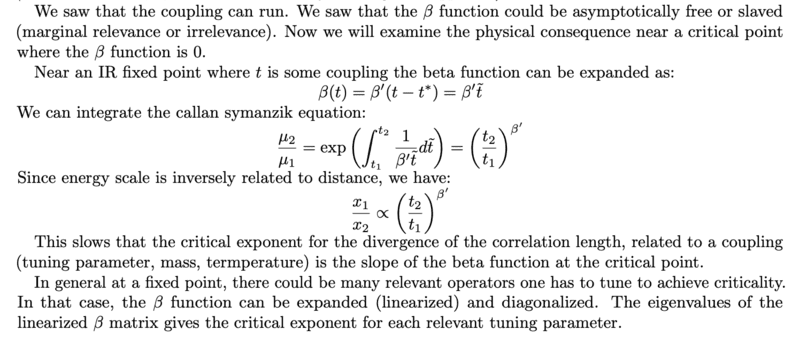
I wanted to construct a simple and clear explanation for the relation between the beta function and the critical exponents (divergence of correlation length) close to a critical point.
I wanted to check if this reasoning is valid. This is my own rewording of complicated arguments I see in standard QFT texts.