Sinusoidal sequences with random phases

[ashah99]

Homework Statement

Please see below from problem statement

Relevant Equations

Ergodicity: ensamble mean = time average mean

Hello all, I have a random sequences question and I am mostly struggling with the last part (e) with deriving the marginal pdf. Any help would be greatly appreciated.
My attempt for the other parts a - d is also below, and it would nice if I can get the answers checked to ensure I'm understanding things properly or if I’m off track.

Problem

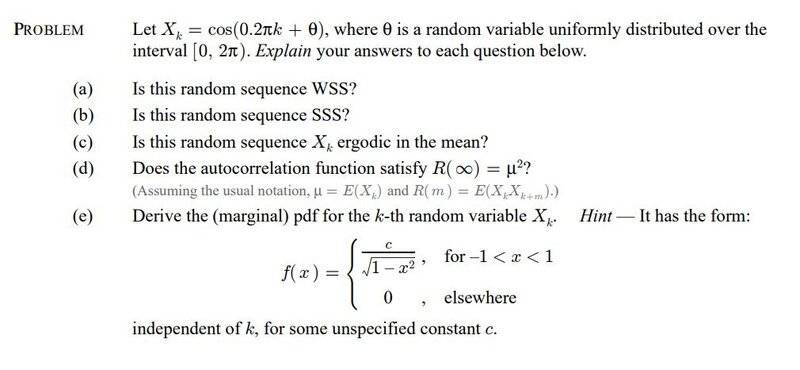
Attempt
For part (a) I got yes, because the mean is 0 (constant) and the autocorrelation function is independent of time k. I got Rx(m) = 0.5*cos(0.2*pi*m)
For (b) I said yes because all statistics are not dependent on time k.
For (c) both the ensemble and time averages would be 0, and since these are equal it seams yes, Xk is ergodic in the mean.
For (d), I believe it is no, because the autocorrelation function is a periodic sinusoid and goes on infinitely, so the limit as Rx(m) goes to infinity does not exist, i.e. it is not constant and not equal to mu ^2

 

[The Bill]

What work have you done on part (e)? Have you set up the problem on paper?

Often, just writing down the problem statement, the given facts, and the relevant definitions gives you a good idea of where to start.