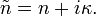Nono713
Gold Member
MHB
- 615
- 4
OK, I've looked around the net but there just doesn't seem to be a straightforward answer that doesn't require you to be an electromagnetics guru, so I'm hoping someone on here can help me out.
I have a light wave incident to a planar interface, coming from a medium with complex refractive index $n_1 + i k_1$, the other side of the interface is a medium with complex refractive index $n_2 + i k_2$. The light wave is incident with the interface normal at an angle $\theta_\text{i}$. You can assume optical wavelengths.
1. What is the (real) angle of refraction $\theta_\text{t}$? Snell's Law here gives a complex angle, which doesn't really make much sense to me. What direction does the refracted wave go in?
2. Is there a phase change upon reflection or transmission? I know about the $\pi$ phase change upon reflection from a medium with higher refractive index, but what does "higher" mean when the indices are complex? Higher real part, or modulus, or something else? And is there any other phase change?
Basically, I'm confused big time about reflection/refraction from absorbing media.
For question 1, I found the following expression in "Descartes-Snell law of refraction with absorption":
$$\frac{\sin \theta_\text{i}}{\sin \theta_\text{t}} = \frac{n_2 \left ( 1 - \frac{k_2^2}{n_2^2} \right )}{n_1}$$
Which looked promising, but it does not work at all when $k_2 \geq n_2$, and for instance for copper, $k = 2.558$ and $n = 1.133$ for 500nm wavelength, so either I am failing to understand the equation, or my data is wrong.
I'm not looking for a "physics student" answer, but actual equations for $\sin \theta_\text{t}$, I'd just like to figure this out so I can move on to the rest of my project, since I need to model absorbing media. Thanks for your insight!
I have a light wave incident to a planar interface, coming from a medium with complex refractive index $n_1 + i k_1$, the other side of the interface is a medium with complex refractive index $n_2 + i k_2$. The light wave is incident with the interface normal at an angle $\theta_\text{i}$. You can assume optical wavelengths.
1. What is the (real) angle of refraction $\theta_\text{t}$? Snell's Law here gives a complex angle, which doesn't really make much sense to me. What direction does the refracted wave go in?
2. Is there a phase change upon reflection or transmission? I know about the $\pi$ phase change upon reflection from a medium with higher refractive index, but what does "higher" mean when the indices are complex? Higher real part, or modulus, or something else? And is there any other phase change?
Basically, I'm confused big time about reflection/refraction from absorbing media.
For question 1, I found the following expression in "Descartes-Snell law of refraction with absorption":
$$\frac{\sin \theta_\text{i}}{\sin \theta_\text{t}} = \frac{n_2 \left ( 1 - \frac{k_2^2}{n_2^2} \right )}{n_1}$$
Which looked promising, but it does not work at all when $k_2 \geq n_2$, and for instance for copper, $k = 2.558$ and $n = 1.133$ for 500nm wavelength, so either I am failing to understand the equation, or my data is wrong.
I'm not looking for a "physics student" answer, but actual equations for $\sin \theta_\text{t}$, I'd just like to figure this out so I can move on to the rest of my project, since I need to model absorbing media. Thanks for your insight!