Solution to Problem 624: Moment Diagrams by Parts

[foo9008]

Homework Statement

http://www.mathalino.com/reviewer/m...ution-to-problem-624-moment-diagrams-by-parts
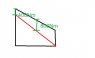
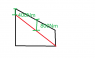
First , 1st solution, the author taking moment about 2m from A?
secondly, the 800Nm is clockwise moment, so it is positive? why the 2400Nm on the right(anticlockwise moment) is also positive?

Homework Equations

The Attempt at a Solution

i think the 2400Nm anticlockwise moment should be negative, which is located under the x-axis of graph , am i right?

 

[foo9008]

in the second solution here, why the 400Nm is drawn from A to 2m away from A? why not the entire length of beam?
The 400nm act along the entire length of beam? Is it true?

 

[foo9008]

i suspect the author is wrong ... ?

 

[pongo38]

"First , 1st solution, the author taking moment about 2m from A?"

The author is taking moments about EVERY point in the beam and then plotting the result as a graph. That is what a bending moment diagram is.

"secondly, the 800Nm is clockwise moment, so it is positive? why the 2400Nm on the right(anticlockwise moment) is also positive?"
The sign is nothing to do with clockwise or anticlockwise (if you went round to the other side of the beam, would the moment change its sign?.
The sign of the bending moment is determined by whether the effect is hogging or sagging. At the point of application of the 400Nm moment, the effects of the two reactions at A and at B are both sagging; that's why they have the same sign. That is just as well, because the definition of bending moment at a section being 'the algebraic sum of the moments on one side (or the other) of the section' must produce the same value whether we take moments to the left, or to the right.

 

[foo9008]

"First , 1st solution, the author taking moment about 2m from A?"

The author is taking moments about EVERY point in the beam and then plotting the result as a graph. That is what a bending moment diagram is.

"secondly, the 800Nm is clockwise moment, so it is positive? why the 2400Nm on the right(anticlockwise moment) is also positive?"
The sign is nothing to do with clockwise or anticlockwise (if you went round to the other side of the beam, would the moment change its sign?.
The sign of the bending moment is determined by whether the effect is hogging or sagging. At the point of application of the 400Nm moment, the effects of the two reactions at A and at B are both sagging; that's why they have the same sign. That is just as well, because the definition of bending moment at a section being 'the algebraic sum of the moments on one side (or the other) of the section' must produce the same value whether we take moments to the left, or to the right.

what do you mean by the author is taking moments about EVERY point in the beam ?
if the author is taking moments about EVERY point in the beam , then how to get the 800Nm , 800Nm is a result of 400N (R1) taking moment about 2m from A , the point which the 400Nm act

 

[foo9008]

why the 400Nmis drawn from A to 2m away from A?why not the 400m drawn throughout the entire length of beam?

 

[foo9008]

"First , 1st solution, the author taking moment about 2m from A?"

The author is taking moments about EVERY point in the beam and then plotting the result as a graph. That is what a bending moment diagram is.

"secondly, the 800Nm is clockwise moment, so it is positive? why the 2400Nm on the right(anticlockwise moment) is also positive?"
The sign is nothing to do with clockwise or anticlockwise (if you went round to the other side of the beam, would the moment change its sign?.
The sign of the bending moment is determined by whether the effect is hogging or sagging. At the point of application of the 400Nm moment, the effects of the two reactions at A and at B are both sagging; that's why they have the same sign. That is just as well, because the definition of bending moment at a section being 'the algebraic sum of the moments on one side (or the other) of the section' must produce the same value whether we take moments to the left, or to the right.

Bump

 

[foo9008]

bump

 

[foo9008]

bump

 

[foo9008]

bump

 

[foo9008]

"First , 1st solution, the author taking moment about 2m from A?"

The author is taking moments about EVERY point in the beam and then plotting the result as a graph. That is what a bending moment diagram is.

"secondly, the 800Nm is clockwise moment, so it is positive? why the 2400Nm on the right(anticlockwise moment) is also positive?"
The sign is nothing to do with clockwise or anticlockwise (if you went round to the other side of the beam, would the moment change its sign?.
The sign of the bending moment is determined by whether the effect is hogging or sagging. At the point of application of the 400Nm moment, the effects of the two reactions at A and at B are both sagging; that's why they have the same sign. That is just as well, because the definition of bending moment at a section being 'the algebraic sum of the moments on one side (or the other) of the section' must produce the same value whether we take moments to the left, or to the right.

why didint the author draw the straight line 400Nm from the point where the 400Nm act to B ?
But , from A to the point where the 400Nm act only ?

 

[foo9008]

ok , now my problem is in the 1st solution, why the author didnt indicate the 400Nm moment in the moment area diagram ? (red circled part)

 

[pongo38]

The so-called 'missing' 400 Nm is the difference between 2400 and -2000. This is a graph preparing for gaphical summation. If you like, you can imagine folding the lower triangle about the horizontal axis, to cancel a good bit of the right hand upper triangle. The zero line would then, in that middle third part of the beam, be a sloping line. it would be instructive for you to do the graphical addition and then reduce everything to a zero horizontal axis throughout. The missing 400 would then be clearer...

 

[foo9008]

The so-called 'missing' 400 Nm is the difference between 2400 and -2000. This is a graph preparing for gaphical summation. If you like, you can imagine folding the lower triangle about the horizontal axis, to cancel a good bit of the right hand upper triangle. The zero line would then, in that middle third part of the beam, be a sloping line. it would be instructive for you to do the graphical addition and then reduce everything to a zero horizontal axis throughout. The missing 400 would then be clearer...

what do u mean? i still have no idea what you are trying to say...
sloping line? how does it relate to 400Nm ?

 

[pongo38]

Unfortunately the M diagram you gave us is not drawn to scale. I suggest you redraw it to scale on graph paper. Let's call C the point of application of the 400 Nm moment, and point D the point of application of the 1000 N load. If you redraw the 2000 Nm triangle above the horizontal axis, as if it were positive, you will get leading ordinates on the graph at point C of: 2400, 2000, and 800 Nm. Draw a line from the +2000 point thus obtained to point D on the horizontal axis; that is the sloping line I am describing. Because much of the 2400 tringle is canceled out by the -2000 triangle, the sloping line becomes a zero axis, everything above it being (to scale) the graphical summation of the two diagrams, and (in this case) everything below it being zero. Lo and behold, the missing 400 appears!

 

[foo9008]

Unfortunately the M diagram you gave us is not drawn to scale. I suggest you redraw it to scale on graph paper. Let's call C the point of application of the 400 Nm moment, and point D the point of application of the 1000 N load. If you redraw the 2000 Nm triangle above the horizontal axis, as if it were positive, you will get leading ordinates on the graph at point C of: 2400, 2000, and 800 Nm. Draw a line from the +2000 point thus obtained to point D on the horizontal axis; that is the sloping line I am describing. Because much of the 2400 tringle is canceled out by the -2000 triangle, the sloping line becomes a zero axis, everything above it being (to scale) the graphical summation of the two diagrams, and (in this case) everything below it being zero. Lo and behold, the missing 400 appears!

Do you mean draw all the moment diagram above x axis? So, we are moving the - 2000Nm diagram which is negative side to positive side?
How could that be? The 2000Nm has different orientation from the moment 2400Nm and 800Nm...

 

[pongo38]

You are right to recognise that the 2400 has a different orientation to the -2000. But, just as you can add a positive number (say, +6) to a negative number (say, -2) to give a result of +4, so you can add areas on a graph. At a point just to the right of C, the result is +2400 -2000= +400 (the same 400 you were looking for). At a point (call it E) mid-way between C and D, 3 m from A, you would be adding 3/4 of 2400 = 1800 to 1/2 of -2000 = -1000, to give a result of +800. Doing that for every point in the region CD gives various results that can be plotted as a graph; most easily, by "folding" the lower triangle about the horizontal axis to then lie on top of the upper triangle, cancelling most of it, and leaving a positive triangle with 400 at C and 1200 at D. that graphical addition seems a bit peculiar because the zero axis has become a sloping line; however, you can redraw it, if you wish, so that the horizontal axis is consistently the zero axis. Please try it on graph paper, and you may find that the penny drops as you do it.

 

[foo9008]

You are right to recognise that the 2400 has a different orientation to the -2000. But, just as you can add a positive number (say, +6) to a negative number (say, -2) to give a result of +4, so you can add areas on a graph. At a point just to the right of C, the result is +2400 -2000= +400 (the same 400 you were looking for). At a point (call it E) mid-way between C and D, 3 m from A, you would be adding 3/4 of 2400 = 1800 to 1/2 of -2000 = -1000, to give a result of +800. Doing that for every point in the region CD gives various results that can be plotted as a graph; most easily, by "folding" the lower triangle about the horizontal axis to then lie on top of the upper triangle, cancelling most of it, and leaving a positive triangle with 400 at C and 1200 at D. that graphical addition seems a bit peculiar because the zero axis has become a sloping line; however, you can redraw it, if you wish, so that the horizontal axis is consistently the zero axis. Please try it on graph paper, and you may find that the penny drops as you do it.

do you mean here is 400Nm (at C ) While the 800Nm is the diffrence in length of the triangle ? refer to the diagram attached .

 

[pongo38]

The diagram is quite a good representation of what I mean, although I am struggling to understand your language: "800Nm is the diffrence in length of the triangle"