Solve Kinematics Problem Homework: Part B

[Precursor]

Homework Statement

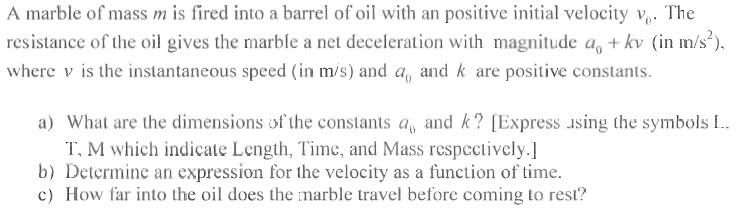The attempt at a solution

I have already solved for part a. It's part b that I'm having trouble with right now.

Here is what I have for part b:

\frac{\mathrm{d} v}{\mathrm{d} t} = a_{0} + kv

dv = a_{0}dt + kvdt

dv = a_{0}dt + adt

\int dv = \int a_{0}dt + adt

And my final answer is:

v = a_{0}t + at

Is this correct?

 

[Delphi51]

I don't understand the 3rd step where you replace kv with a.
Are you assuming that the acceleration is kv ?

Also in the final step, you assume that "a" is a constant.
Acceleration can't be constant; it is proportional to the velocity and the velocity is decreasing.

I have forgotten how to solve differential equations, but you might try a substitution to make it look easier. If you let v = u + b where b is a constant, then your dv/dt = ao + kv will change to
du/dt = ao +kb + ku
and you can choose b = -ao/k so
du/dt = ku.
This is the equation for radioactive decay, and it can easily be integrated to get u as an exponential function. Change variables back to v to get an exponential function for v.

 

[Precursor]

I made kv = a because the dimension of k is 1/T, where T is time, and v is simply velocity. Therefore, the product of these two variables will be acceleration.

 

[Delphi51]

But kv is not a constant, so your "a" is not a constant and its integral over time is not at.

 

[Precursor]

Does this mean that the integral of adt is equal to v.

 

[Delphi51]

Your "a" is really "k*v" so its integral over time would be k*distance + constant, where distance is a function of time. I don't think this does any good. Better to leave the kv in the differential equation and make the substitution I suggested in the 2nd post.

 

[RTW69]

I don't think your integration is correct. Go to Wolframalpha and use Mathematica's DSolve command for v'[t]=a+k*v[t] I think the solution is v[t]=-a/k + e^(k*t) C1 but not 100% sure since I'm not a regular user of Mathematica