- #1
Solve ln(a/b+1) Using Identity ln(a+b)=ln b + ln(a/b+1)
- I
- Thread starter Gurasees
- Start date
-
- Tags
- Logarithm
In summary, the conversation is discussing how to solve the expression "ln(a/b+1)" after applying the identity "ln(a+b)=ln b + ln(a/b+1)". It is mentioned that this expression depends on the variable T and numerical approximations may be needed to find a specific value. The conversation also includes a request for helpful links and resources for solving this type of expression. Finally, a link to the power series expansion of logarithmic functions is provided, with a caution about the range in which it can be applied.
- #2
- 19,686
- 25,658
What do you mean by "solve"?
- #3
Gurasees
- 50
- 1
The identity keeps repeating itself in the solution,fresh_42 said:
I want to take ln of
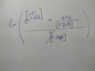
- {e^(-6.7/(T-292)) + 0.56/(T-292)} / {3/(T-292)}
- #4
- #5
- 19,686
- 25,658
So you have ##\log \left( (T-292)\exp\left( \dfrac{-6.7}{T-292} \right) + 0.56 \right) - \log(3)\,.##
I think you can only solve this numerically, resp. expand the exponential function and the logarithm into power series, in which case you must consider the interval of convergence.
I think you can only solve this numerically, resp. expand the exponential function and the logarithm into power series, in which case you must consider the interval of convergence.
- #6
Gurasees
- 50
- 1
I don't know how to do that. Can you send me some links which might be helpful for the solution?fresh_42 said:
- #7
- 19,686
- 25,658
Solution of what? You cannot simplify this expression further. To solve something, an equation would be helpful. You can find the power series on Wikipedia if you look for the exponential, resp. logarithmic function. But look out for the range of ##T-292\,.##Gurasees said:
- #8
mfb
Mentor
- 37,262
- 14,098
I still don't see an equation you could solve. Can you "solve a+b"?
We have an expression that depends on T. Should that be equal to something specific? Do you want to find a T such that the expression will obtain a given value? That will need numerical approximations.
We have an expression that depends on T. Should that be equal to something specific? Do you want to find a T such that the expression will obtain a given value? That will need numerical approximations.
- #9
Gurasees
- 50
- 1
fresh_42 said:
Thanks for your help.fresh_42 said:
- #10
Gurasees
- 50
- 1
I can't find the expansion of ln(x-a). Can you help?fresh_42 said:
- #11
- 19,686
- 25,658
https://en.wikipedia.org/wiki/Logarithm#Power_seriesGurasees said:
but be cautious with the range in which they can be applied. You have to have some knowledge about the quantity ##T-292##.
In case you have an actual equation, I would let do wolframalpha the job: https://www.wolframalpha.com/
FAQ: Solve ln(a/b+1) Using Identity ln(a+b)=ln b + ln(a/b+1)
What is the identity used to solve ln(a/b+1)?
The identity used to solve ln(a/b+1) is ln(a+b)=ln b + ln(a/b+1).
How do you apply the identity to solve ln(a/b+1)?
To apply the identity, you first need to identify the values of a and b in the given equation. Then, you can substitute those values into the identity and simplify the equation to solve for ln(a/b+1).
Can the identity be used for any value of a and b?
Yes, the identity can be used for any value of a and b as long as they are both positive numbers.
What is the purpose of using the identity to solve ln(a/b+1)?
The purpose of using the identity is to simplify the given equation and make it easier to solve for ln(a/b+1).
Are there any other identities that can be used to solve ln(a/b+1)?
Yes, there are other identities that can be used to solve ln(a/b+1), such as ln(x/y)=ln x - ln y and ln(a/b)=ln a - ln b. However, the identity ln(a+b)=ln b + ln(a/b+1) is the most commonly used for this specific equation.