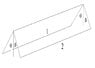
- #1
doodijh
- 10
- 0
Homework Statement
Two wires have a current going through them and each wire is suspended by a string from the ceiling.
Wire 2 makes an angle of β with the vertical and Wire 1 makes and angle of θ with the vertical.
(see the attached picture for further clarification)
L 1(length of wire) = ?
I 1(current) = ?
L 2(length of wire) = ?
I 2(current) = ?
B (magnetic field) =?
D (distance in between the two wires) =?
M1 (mass of wire 1) = ?
M2 = ?
Fm = ?
a) Find the direction of current in the two wires?
b) State the magnitude of the magnetic force between the two wires (1&2)?
c) Find the magnitude of the magnetic force in each wire?
Homework Equations
Current carrying wire in a magnetic field
Magnetic field due to current carrying wire
Magnetic force between two current carrying wires
[tex]\mi_{o}\ =\ 4\pi\ \times\ 10^{-7}[/tex]
The Attempt at a Solution
a) the two wires seem to be attracting each other because if they were repulsive, then their angles from the vertical must be the same. According to the right hand rule, the two wires have to point in the same direction in order to have an attractive force with each other.
b&c) I had no idea of how to do them. I just wrote the appropriate equation beneath the letters. This question was the last question from my test in magnetism (by the way I am a grade 12 university student)
If I get the test back, then I will post the solution to this problem, but I would like to see how u people would solve this problem.
Attachments
Last edited: