MHB Solve Parameters of Displacement Function - Raja's Question

[MarkFL]

Here is the question:

How do you solve the following question?


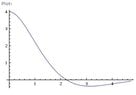
A particle is moving in a straight line. The displacement x, from an origin O on the line, is given at time t by the equation x=e^(-3/4 t) (a sin t + b cos t).

Initially t=0, x=4, dx/dt = 0. Find the constants a and b. Determine also (i) the time elapsing from the start before the particle first reaches O, (ii) the time taken from O to attain the greatest displacement on the negative side of the origin.

I have posted a link there to this topic so the OP can see my work.

 

[MarkFL]

Hello raja,

We are given the displacement function:

$$x(t)=e^{-\frac{3}{4}t}\left(a\sin(t)+b\cos(t) \right)$$

and we are told:

$$x(0)=4$$

And so this implies:

$$x(0)=e^{-\frac{3}{4}\cdot0}\left(a\sin(0)+b\cos(0) \right)=b=4$$

We may differentitate the displacement function with respect to time $t$ to obtain the velocity function:

$$v(t)=\frac{dx}{dt}=e^{-\frac{3}{4}t}\left(a\cos(t)-b\sin(t) \right)-\frac{3}{4}e^{-\frac{3}{4}t}\left(a\sin(t)+b\cos(t) \right)$$

We are given:

$$v(0)=0$$

Hence:

$$v(0)=e^{-\frac{3}{4}\cdot0}\left(a\cos(0)-b\sin(0) \right)-\frac{3}{4}e^{-\frac{3}{4}\cdot0}\left(a\sin(0)+b\cos(0) \right)=a-\frac{3}{4}b=a-3=0\,\therefore\,a=3$$

And so we have found:

$$a=3,\,b=4$$

Thus:

$$x(t)=e^{-\frac{3}{4}t}\left(3\sin(t)+4\cos(t) \right)$$

Using a linear combination identity, we may write this as:

$$x(t)=5e^{-\frac{3}{4}t}\sin\left(t+\tan^{-1}\left(\frac{4}{3} \right) \right)$$

i) Because the exponential factor has no real roots, to find the time it takes for the particle to first reach the origin, we simply need to equate the argument of the sine function to $\pi$:

$$t+\tan^{-1}\left(\frac{4}{3} \right)=\pi$$

$$t=\left(\pi-\tan^{-1}\left(\frac{4}{3} \right) \right)\text{ s}\approx2.21429743558818\text{ s}$$

ii) We may differentiate the displacement function to obtain the velocity function:

$$v(t)=5\left(e^{-\frac{3}{4}t}\cos\left(t+\tan^{-1}\left(\frac{4}{3} \right) \right)-\frac{3}{4}e^{-\frac{3}{4}t}\sin\left(t+\tan^{-1}\left(\frac{4}{3} \right) \right) \right)$$

$$v(t)=\frac{5}{4}e^{-\frac{3}{4}t}\left(4\cos\left(t+\tan^{-1}\left(\frac{4}{3} \right) \right)-3\sin\left(t+\tan^{-1}\left(\frac{4}{3} \right) \right) \right)$$

Using a linear combination identity, we may write:

$$v(t)=\frac{25}{4}e^{-\frac{3}{4}t}\sin\left(t+\tan^{-1}\left(\frac{4}{3} \right)+\tan^{-1}\left(-\frac{4}{3} \right)+\pi \right)$$

$$v(t)=-\frac{25}{4}e^{-\frac{3}{4}t}\sin(t)$$

Thus, we see by the first derivative test that the particle has a relative minimum at:

$$t=\pi\text{ s}\approx3.14159265358979\text{ s}$$

And because of the decaying amplitude, we know that for $0<t$, this is the global minimum.

Here is a plot of the displacement function:

View attachment 1431