MHB Solve Right Triangle Problem Without Knowing Bottom Line

Click For Summary
To solve a right triangle problem without knowing the base length, one can use trigonometric relationships involving angles. The angle θ can be utilized to find the hypotenuse (d) with the formula d = 16/cos(θ), where 16 meters is the adjacent side. The discussion raises skepticism about the possibility of determining a numerical value for d with only the angle θ provided. Clarification is sought on how one could arrive at a solution without additional information. Understanding these trigonometric principles is essential for solving similar problems effectively.
Jacob123
Messages
1
Reaction score
0
How would I have to calculate this question for an answer, a friend of mine told me he could get the answer without knowing that the bottom line was 16 meters, I can't seem to find a way that would work, I am not sure if I am missing something or he is lying.

Screenshot_90.png
 
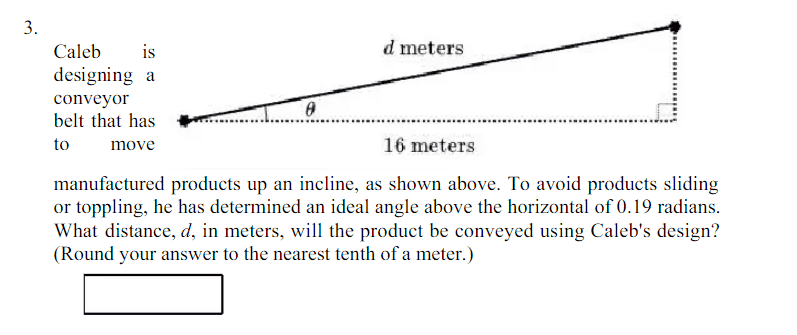
Last edited by a moderator:
Mathematics news on Phys.org
I'd also like to know how your friend could determine a numerical value for $d$ just knowing the angle $\theta = 0.19$ with no other information.
If he let's you know how, post back and enlighten me.

Using the angle, $\theta$, value and the 16 meters ...

$\cos{\theta} = \dfrac{16}{d} \implies d = \dfrac{16}{\cos{\theta}}$
 

Similar threads

I Geometry problem of interest with a 3-4-5 triangle
  • · Replies 59 ·
2
Replies
59
Views
2K
MHB Can an Ellipse Help Solve the Scalene Triangle Problem?
  • · Replies 1 ·
Replies
1
Views
1K
MHB Length of sides of right triangle with 1 angle and 1 side
  • · Replies 2 ·
Replies
2
Views
2K
MHB Find unknown angles of a triangle
  • · Replies 6 ·
Replies
6
Views
3K
MHB Are the coordinates of the vertices of this triangle all integers?
  • · Replies 3 ·
Replies
3
Views
2K
MHB Find the Sides of Triangle DEF: A Challenge!
  • · Replies 4 ·
Replies
4
Views
3K
Finding area of a non right angled triangle
  • · Replies 9 ·
Replies
9
Views
3K
MHB Find the height and base of a trapezium
  • · Replies 1 ·
Replies
1
Views
2K
Right Triangle Maximization Problem
  • · Replies 5 ·
Replies
5
Views
3K
MHB *find eq of perpendicular line so that the area of the triangle is 8
  • · Replies 3 ·
Replies
3
Views
2K