Solve the given inequality

[chwala]

Homework Statement

see attached

Relevant Equations

inequalities

I am interested in an algebraic approach.

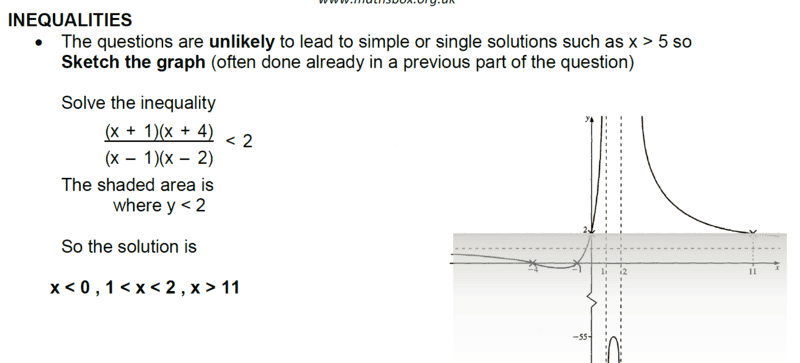
My lines are as follows;

$\dfrac{(x+1)(x+4)}{(x-1)(x-2)} -2<0$

$\dfrac{(x^2+5x+4) - 2(x-1)(x-2)}{(x-1)(x-2)} <0$

The denominator will give us the vertical asymptotes $x=1$ and $x=2$

The numerator gives us,

$x^2+5x+4-2x^2+6x-4 <0$

$-x^2+11x<0$

$x(-x+11)<0$

$x=0$ is critical value $⇒x<0$ and the other critical value is $x=11$ $⇒x>11$. On checking together with the asymptotes, we end up with
$x<0, 1<x<2$ and $x>11$.
Any insight or positive criticism is welcome.

 

[Office_Shredder]

You said "the numerator gives us" but the things that follows is only true if the denominator is positive, which you haven't explicitly handled i think?

 

[Hill]

You have converted the original inequality into
$$\dfrac{x(-x+11)}{(x-1)(x-2)} <0$$
This means that either
$$x(-x+11)<0, (x-1)(x-2)>0$$ or $$x(-x+11)>0, (x-1)(x-2)<0$$
These two options further split:
$$x<0, (-x+11)>0, (x-1)>0,(x-2)>0$$ $$x>0, (-x+11)<0, (x-1)>0,(x-2)>0$$ $$x<0, (-x+11)>0, (x-1)<0,(x-2)<0$$ $$x>0, (-x+11)<0, (x-1)<0,(x-2)<0$$ $$x<0, (-x+11)<0, (x-1)<0,(x-2)>0$$ $$x>0, (-x+11)>0, (x-1)<0,(x-2)>0$$ $$x<0, (-x+11)<0, (x-1)<0,(x-2)>0$$ $$x>0, (-x+11)>0, (x-1)<0,(x-2)>0$$
The first inequality gives $$x<0,x<11,x>1,x>2$$ which is empty.
Etc.

 

[pasmith]

We require $$f(x) = \frac{x(11-x)}{(x-1)(x - 2)} < 0.$$ The numerator and denominator have no common linear factors, so $f$ changes sign whenever a linear factor of either the numerator or the denominator changes sign. Thus we have sign changes at $x = 0, 1, 2, 11$.

Starting at $x = -\infty$, we see that $f(x) \sim -1$, so $f(x) < 0$ for $x < 0$ and $1 < x < 2$ and $x > 11$.

 

[chwala]

We require $$f(x) = \frac{x(11-x)}{(x-1)(x - 2)} < 0.$$ The numerator and denominator have no common linear factors, so $f$ changes sign whenever a linear factor of either the numerator or the denominator changes sign. Thus we have sign changes at $x = 0, 1, 2, 11$.

Starting at $x = -\infty$, we see that $f(x) \sim -1$, so $f(x) < 0$ for $x < 0$ and $1 < x < 2$ and $x > 11$.

For one to use sign change one has to take the derivative of $f(x)$ with respect to $x$?

 

[Mark44]

For one to use sign change one has to take the derivative of $f(x)$ with respect to $x$?

No, you don't need to take the derivative. For example, looking at the x - 1 factor in the denominator, it will change sign for x on either side of 1, but close to 1. The factors in the numerator, x and 11 - x don't change sign. The other factor in the denominator, x - 2, also doesn't change sign.

Since no other factors change sign, the y value changes sign for values near 1, but on either side of 1. This can be seen by looking at the graph you attached in the OP.