Solve the given trigonometry equation

[chwala]

Homework Statement

see attached

Relevant Equations

Trigonometry

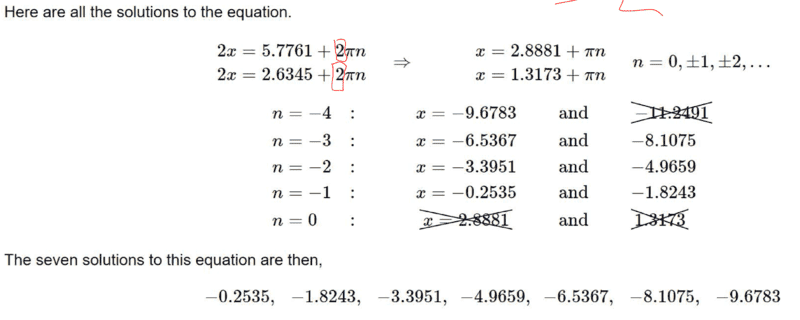
This is the problem. The question is simple i just need some clarification as indicated on the part highlighted below in red.

Now from my understanding tangent repeats on a cycle of $π$ radians...why do we have 2 the part circled in red below i.e $2$? This is the part that i need clarity. Why consider cycles for sine and cosine here?
It's straightforward and less stressful to just use $π$radians in my opinion.

The other form given below is the one that i know and am very much conversant with;

 

[chwala]

We know that $\tan^{-1}$$\left[-\dfrac{5}{9}\right]=-0.5071$.

Tangent is negative in the second and fourth quadrant respectively. This will realize the given values: $-0.5071+π$ and $2π-0.5071$ respectively... giving us the desired $2.6345$ and $5.7761$
i.e
$2x=-0.5071+nπ⇒x=-0.25355+\dfrac{1}{2}nπ$

$2x=2.6345+nπ⇒x=1.31725+\dfrac{1}{2}nπ$

$2x=5.7761+nπ⇒x=2.88805+\dfrac{1}{2}nπ$

where $n=0, ±1, ±2...$ and given that the solution lies on $-10≤x≤0$ then we shall get the answers as shown on the text.

Cheers.