chwala
Gold Member
- 2,827
- 415
- TL;DR Summary
- See attached.
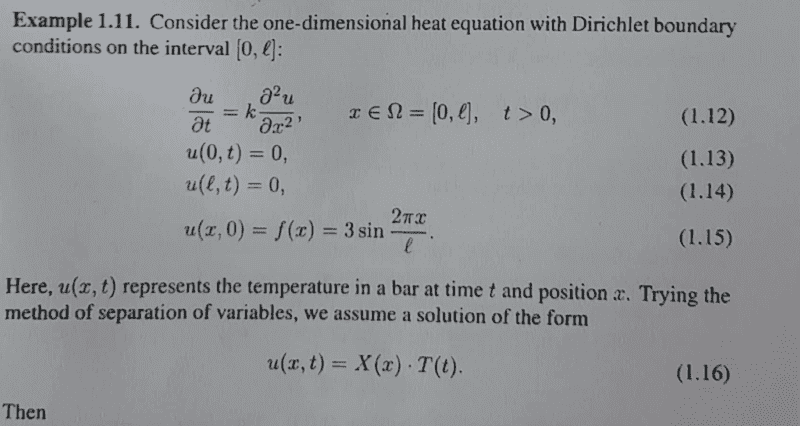
I am going through these notes...they are pretty easy to follow. I would like more insight on the initial condition. In this problem, (attachment below), i guess the choice of initial condition is convenient as its easier to plug in the values of ##n=2## and ##b=3## (highlighted on the attachment) to realize the required solution. My question is? supposing we had the initial condition being;
##f(x)=\dfrac{1}{2}\sin\left[\dfrac{4πx}{l}\right]## where then our ##n=4## and ##b_4=\dfrac{1}{2}##
then our solution would be;
##U(x,t)##= ##\dfrac{1}{2} e^{\left[\dfrac{-16π^2kt}{l^2}\right]} ×\sin\left[ \dfrac{4πx}{l}\right]## correct?
I think the other highlighted part is now clear to me i.e when the initial condition is not an eigen function, then we have to make use of the principle of superposition. (one thing that is clear to me here is that for us to have the eigen function then the initial condition has to be either a function of sine or cosine).
Can we have the initial condition being a non-trigonometric function which would give us an eigenfunction?
Cheers!
Find the problem and solution here from textbook;


##f(x)=\dfrac{1}{2}\sin\left[\dfrac{4πx}{l}\right]## where then our ##n=4## and ##b_4=\dfrac{1}{2}##
then our solution would be;
##U(x,t)##= ##\dfrac{1}{2} e^{\left[\dfrac{-16π^2kt}{l^2}\right]} ×\sin\left[ \dfrac{4πx}{l}\right]## correct?
I think the other highlighted part is now clear to me i.e when the initial condition is not an eigen function, then we have to make use of the principle of superposition. (one thing that is clear to me here is that for us to have the eigen function then the initial condition has to be either a function of sine or cosine).
Can we have the initial condition being a non-trigonometric function which would give us an eigenfunction?
Cheers!
Find the problem and solution here from textbook;
Last edited: