Solve the problem involving space curve

[chwala]

Homework Statement

see attached.

Relevant Equations

Vector differentiation

Refreshing... i'll start with part (a).

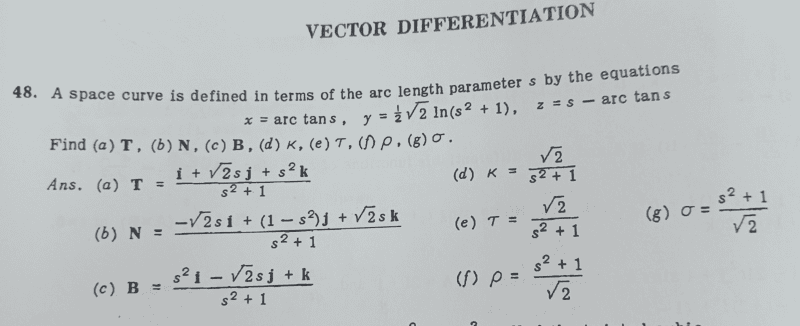
Just sharing in case there is more insight...

In my working i have,

$T = \dfrac{dr}{ds}=\dfrac{dx}{ds}i + \dfrac{dy}{ds}j + \dfrac{dz}{ds}k$

and

$x=\tan^{-1} s, y = \dfrac{\sqrt2}{2} \ln (s^2+1), z=\tan^{-1} s$

$\dfrac{ds}{dx} = \sec^2 x = 1 +\tan^2x$

$\dfrac{dx}{ds}= \dfrac{1}{1+s^2}$.

similarly,

$\dfrac{dy}{ds}=\dfrac{\sqrt 2}{2}⋅ \dfrac{1}{s^2+1}⋅2s = \dfrac{\sqrt 2}{s^2+1}s$

...
thus,

$T=\dfrac{1}{1+s^2} i + \dfrac{\sqrt 2}{s^2+1}sj + \left(1-\dfrac{1}{1+s^2}\right)$
$T=\dfrac{1}{1+s^2} i + \dfrac{\sqrt 2}{s^2+1}sj + \dfrac{s^2k}{1+s^2}$

For (d), curvature

My lines are

$\dfrac{dT}{ds} = \dfrac{-2s}{(1+s^2)^2} i + \dfrac{\sqrt 2(1-s^2)}{(1+s^2)^2}j +\dfrac{2s}{(1+s^2)^2}k$

$k=\dfrac{|dT|}{|ds|}= \dfrac{4s^2+2(1-s^2)^2 +4s^2}{(1+s^2)^4}$

$k=\sqrt{\dfrac{2s^4+4s^2+2}{(1+s^2)^4}}=\sqrt{\dfrac{2(s^2+1)^2}{(1+s^2)^4}}=\dfrac{\sqrt2⋅ (s^2+1)}{(1+s^2)^2}=\dfrac{\sqrt2}{1+s^2}$

...involves some bit of working...cheers ...rest of questions can be solved similarly as long as one knows the formula and how to differentiate...any insight is welcome. bye.

 

[jedishrfu]

You could continue and solve N and B and then show that T,N and B are all perpendicular.

https://en.wikipedia.org/wiki/Frenet–Serret_formulas

 

[chwala]

You could continue and solve N and B and then show that T,N and B are all perpendicular.

https://en.wikipedia.org/wiki/Frenet–Serret_formulas

Yes I'll do that later...

done already for $N$ and $B$... Not difficult ...had to use cross product... let me post my working later. Cheers man!