Solve the problem that involves Binomial distribution and hypothesis test

[chwala]

Homework Statement

see attached

Relevant Equations

Binomial distribution

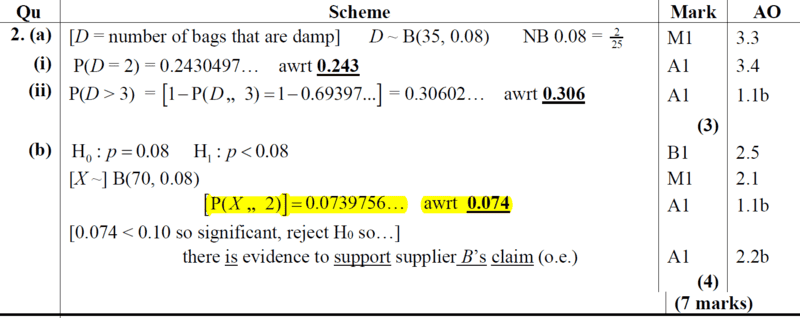
For this problem, the steps are quite clear. My question would be on the highlighted part, clearly we are told $n=2$ as claim from supplier then why did they consider other $n$ values? that is, $n=1$ and $n=0$ to give us,

$[P,X=2] = 0.002918+0.017764 + 0.053292 = 0.07397562$

why did they not use the value for $n=2$ as it is? Maybe, I am not getting the word problem clearly...

 

[Mark44]

why did they not use the value for n=2 as it is? Maybe, I am not getting the word problem clearly...

Because P(D = 2) (i.e., exactly 2 damp bags) is part (a), subpart (i). Subpart (ii) asks you to find P(D > 3).

 

[chwala]

Because P(D = 2) (i.e., exactly 2 damp bags) is part (a), subpart (i). Subpart (ii) asks you to find P(D > 3).

Sorry my question is on question 2(b) only. I have no problem with 2 (i) and 2(ii). This part is easy for me.

This part is fine,

For 2(i), i have

${_{35}C_2} (0.08)^2 (0.92)^{33} = 0.243$

For part (ii) i have,

$P(D>3) = 1 - [0.054+0.1644+0.2430+0.2324]$
$P(D>3) = 1 - 0.6938=0.3062$

My question is on 2(b)
They clearly used

$[P,X=2] = 0.002918+0.017764 + 0.053292 = 0.07397562$

This is where my problem is as the question states only 2 of the bags are damp.

Why not $[P,X=2] = 0.05329$

 

[Mark44]

I don't understand what their notation is saying, for example [P(X ,, 2)] or in the work for 2a(ii) where they have [1 - P(D ,, 3) = ...]

Also, it looks like the answer key has a typo in the second line of 2b:

[X~]B(70, 0.08)

I believe this should be [X ~ B(70, 0.08)], meaning that X is a random variable with a binomial distribution with n = 70 and p = 0.08.
,

 

[chwala]

I don't understand what their notation is saying, for example [P(X ,, 2)] or in the work for 2a(ii) where they have [1 - P(D ,, 3) = ...]

Also, it looks like the answer key has a typo in the second line of 2b:

I believe this should be [X ~ B(70, 0.08)}, meaning that X is a random variable with a binomial distribution with n = 70 and p = 0.08.
,

Correct, that is a typo.

 

[Mark44]

Do you understand what they mean by [P(X ,, 2)]? Do they explain this notation elsewhere in the textbook?

 

[chwala]

Do you understand what they mean by [P(X ,, 2)]? Do they explain this notation elsewhere in the textbook?

I do not understand.

This question is from an A-level past paper question.

 

[chwala]

Do you understand what they mean by [P(X ,, 2)]? Do they explain this notation elsewhere in the textbook?

... but going with the mark scheme guide, that should not be difficult to determine. It is simply a sum of combinations that is, P[X=0 ] + P[X=1 ] + P[X=2] = P[X ,,2].

 

[Mark44]

They clearly used
$[P,X=2] = 0.002918+0.017764 + 0.053292 = 0.07397562$

This is where my problem is as the question states only 2 of the bags are damp.

Why not $[P,X=2] = 0.05329$

I believe the idea here is that they're calculating the probability $P(X \le 2)$, which means they need to calculate the sum $P(X = 0) + P(X = 1) + P(X - 2)$. I get the same numbers you did and that are shown in the mark scheme.
Like I said, their notation leaves something to be desired. It would be clearer if they wrote $P(X \le 2)$ or maybe [P, X=0...2] with the understanding that X ~ B(70, .08). Keep in mind that the hypothesis test is whether p = 0.08 vs the alternate hypothesis that p < 0.08.

 

[chwala]

I don't think you understood my question. The way they worked the problem to solution is quite clear @Mark44

I just need to understand the highlighted part in post $3$.

 

[Mark44]

I don't think you understood my question.

I don't think you understood the question for 2b.
A candy manufacturer makes the claim that 8% of the bags of sugar that are received from the supplier are wet. The supplier disputes that claim, and instead asserts that the proportion of wet bags of sugar is less than 8%.
The supplier sets up a hypothesis test to determine a confidence interval with $\alpha = 0.10$ for the true value of p, the proportion of wet sugar bags.
$H_0 : p = 0.08$ -- The manufacturer's claim
$H_a : p < 0.08$ -- The supplier's claim

The null hypothesis is equivalent to $P(D = 2)$. The alternate hypothesis is equivalent to $P(D \le 2)$ which in turn is equal to $P(D = 0) + P(D = 1) + P(D = 2)$. If this probability turns out to be less than 0.10, we reject the null hypothesis because we're way into the left tail of the distribution. If that probability turns out to be 0.10 or larger, we accept the null hypothesis.

As you have already found, $P(D \le 2) \approx 0.074$, which implies that in actuality, fewer than 8% of the bags of sugar are wet.