Solving 2-Body Problem w/ Lagrangian: What Substitutions?

In summary, the speaker is asking for a demonstration for which substitutions can be made before taking partial derivatives in the classical two body problem with Lagrangian Principle. They believe that the answer may involve holonomic constraints.
  • #1
EduardoToledo
3
0
Hi, I was trying to solve the classical two body problem with Lagrangian Principle. I replaced the angular velocity before taking the partial derivatives (which respect to the distance to the virtual particle) and the result was completely different. I would like to ask, therefore, which substitutions can I do before taking these partial derivatives. I think the answer may be "the ones with holonomic constraints", but I really would like the demonstration for that
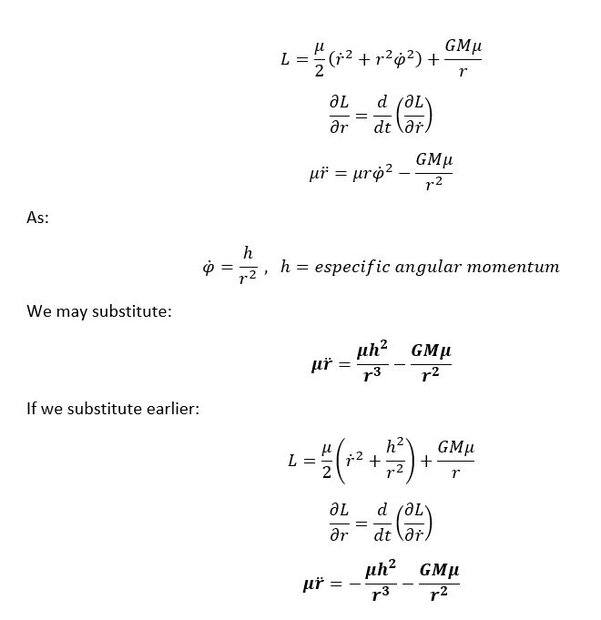substitution in euler lagrangian equation.JPG
.
 
Physics news on Phys.org
  • #2
EduardoToledo said:
I would like to ask, therefore, which substitutions can I do before taking these partial derivatives
$$q\mapsto f(t,Q),\quad \dot q\mapsto f_t+f_Q\dot Q$$
 
  • Like
Likes vanhees71

FAQ: Solving 2-Body Problem w/ Lagrangian: What Substitutions?

What is the 2-body problem?

The 2-body problem is a classical mechanics problem that involves finding the motion of two point masses under the influence of their mutual gravitational attraction. It is a simplified version of the n-body problem, which involves finding the motion of n point masses.

What is the Lagrangian method?

The Lagrangian method is a mathematical approach used to solve problems in classical mechanics. It involves defining a function called the Lagrangian, which represents the difference between the kinetic and potential energies of a system. By solving the equations of motion derived from the Lagrangian, the motion of a system can be determined.

Why are substitutions necessary in solving the 2-body problem with Lagrangian?

Substitutions are necessary in solving the 2-body problem with Lagrangian because the equations of motion derived from the Lagrangian are often difficult to solve directly. By making substitutions, the equations can be simplified and solved more easily.

What are some common substitutions used in solving the 2-body problem with Lagrangian?

Some common substitutions used in solving the 2-body problem with Lagrangian include transforming the coordinates into a rotating frame of reference, using polar coordinates, and using the reduced mass of the system. These substitutions can simplify the equations and make them easier to solve.

What are the limitations of using the Lagrangian method to solve the 2-body problem?

One limitation of using the Lagrangian method to solve the 2-body problem is that it assumes the system is in equilibrium, meaning that the forces acting on the bodies are balanced. It also assumes that the system is conservative, meaning that no external forces act on the system. Additionally, the Lagrangian method may not be applicable to all types of systems and may not provide an exact solution in some cases.

Similar threads

I Lagrangian density of a linearly elastic string under gravity
Replies
6
Views
1K
I Requirement of Holonomic Constraints for Deriving Lagrange Equations
Replies
1
Views
794
I Taylor expansion about lagrangian in noether
Replies
1
Views
963
I Lagrangian doesn't change when adding total time derivative
Replies
19
Views
2K
I Confused about applying the Euler–Lagrange equation
Replies
3
Views
963
I Why isn't the Lagrangian invariant under ##\theta## rotations?
Replies
5
Views
1K
A An ab initio Hilbert space formulation of Lagrangian mechanics
Replies
10
Views
1K
A Mass measurement in a Penning trap
Replies
0
Views
470
I Proving that the Lagrangian of a free particle is independent of q
Replies
1
Views
762
Lagrangian of two body problem with spin
Replies
4
Views
2K

Hot Threads

Recent Insights

Back
Top