bob012345
Gold Member
- 2,287
- 1,011
- TL;DR Summary
- Solving a 2D temperature problem but having an issue with the proper setup of the Galerkin method. All my terms are zero.
I want to solve the 2D heat equation
$$\frac{∂^2 {T}}{ ∂x^2} + \frac{∂^2 {T}}{ ∂y^2} = 0$$
The only boundary conditions is I will specify the edge temperatures but there are no heat sources.
So I create an average temperature function ##\tilde{T}## and weighting functions ##S_i## over a rectangular element with four nodes with i=1,2,3,4.
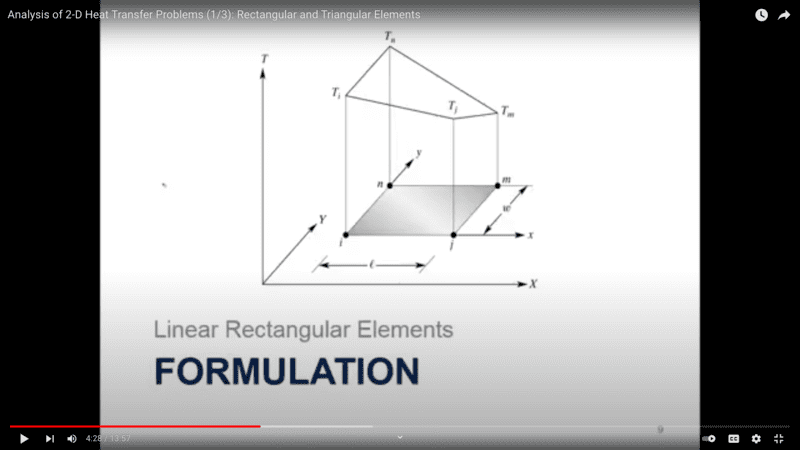
Using numbers instead of letters for clarity I wish to solve first for the weighting functions assuming a form for my average temperature function;
$$\tilde{T} = b_1 + b_2x +b_3y +b_4xy$$
I solve for the coefficients to get my weighting functions and my average temperature function;
$$S_1 = (1 - \frac{x}{l}) (1 - \frac{y}{w})$$
$$S_2 = \frac{x}{l}(1 - \frac{y}{w})$$
$$S_3 = \frac{xy}{lw}$$
$$S_4 = \frac{y}{w}(1 - \frac{x}{l})$$
Then;
$$\tilde{T} = T^e = S_1T_1 + S_2T_2 + S_3T_3 + S_4T_4$$
Following the Galerkin method I minimize the Residual
$$\iint_A S_i R \,dA = \iint_A S_i (\frac{∂^2 \tilde{T}}{ ∂x^2} + \frac{∂^2 \tilde{T}}{ ∂y^2} )\,dA = 0$$
Now I use integration by parts to eliminate the second order derivative. This is just for the x derivative part of one of the four equations;
$$\iint_A S_i \frac{∂^2 \tilde{T}}{ ∂x^2}\,dA = \int_Y \Big[ S_i \frac{∂ \tilde{T}}{ ∂x} \Big |_{x_1}^{x_2} - \int_X \frac{∂S_i}{∂x} \frac{∂ \tilde{T}}{ ∂x} \,dX \Big] dY$$ where the index goes to 4.
My problem is when I integrate this to get my node equations all the terms go to zero. I mean the two integrals exactly cancel for each node variable. My question is this, did I set up this correctly with the integration by parts over a double integral? I used the integration of parts for the x integral then when manipulating the x derivative terms. Thanks.
EDIT: I think I found the issue. It is how to evaluate the first term after integration by parts. I was substituting the full expression for ##\frac{∂ \tilde{T}}{ ∂x}## back in when it should be evaluated as the slope at the limits of integration. I found it by revisiting my 1D reference problem. Thanks.
$$\frac{∂^2 {T}}{ ∂x^2} + \frac{∂^2 {T}}{ ∂y^2} = 0$$
The only boundary conditions is I will specify the edge temperatures but there are no heat sources.
So I create an average temperature function ##\tilde{T}## and weighting functions ##S_i## over a rectangular element with four nodes with i=1,2,3,4.
Using numbers instead of letters for clarity I wish to solve first for the weighting functions assuming a form for my average temperature function;
$$\tilde{T} = b_1 + b_2x +b_3y +b_4xy$$
I solve for the coefficients to get my weighting functions and my average temperature function;
$$S_1 = (1 - \frac{x}{l}) (1 - \frac{y}{w})$$
$$S_2 = \frac{x}{l}(1 - \frac{y}{w})$$
$$S_3 = \frac{xy}{lw}$$
$$S_4 = \frac{y}{w}(1 - \frac{x}{l})$$
Then;
$$\tilde{T} = T^e = S_1T_1 + S_2T_2 + S_3T_3 + S_4T_4$$
Following the Galerkin method I minimize the Residual
$$\iint_A S_i R \,dA = \iint_A S_i (\frac{∂^2 \tilde{T}}{ ∂x^2} + \frac{∂^2 \tilde{T}}{ ∂y^2} )\,dA = 0$$
Now I use integration by parts to eliminate the second order derivative. This is just for the x derivative part of one of the four equations;
$$\iint_A S_i \frac{∂^2 \tilde{T}}{ ∂x^2}\,dA = \int_Y \Big[ S_i \frac{∂ \tilde{T}}{ ∂x} \Big |_{x_1}^{x_2} - \int_X \frac{∂S_i}{∂x} \frac{∂ \tilde{T}}{ ∂x} \,dX \Big] dY$$ where the index goes to 4.
My problem is when I integrate this to get my node equations all the terms go to zero. I mean the two integrals exactly cancel for each node variable. My question is this, did I set up this correctly with the integration by parts over a double integral? I used the integration of parts for the x integral then when manipulating the x derivative terms. Thanks.
EDIT: I think I found the issue. It is how to evaluate the first term after integration by parts. I was substituting the full expression for ##\frac{∂ \tilde{T}}{ ∂x}## back in when it should be evaluated as the slope at the limits of integration. I found it by revisiting my 1D reference problem. Thanks.
Last edited: