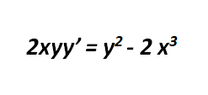Solving a First Order Linear Differential Equation with an Initial Condition

[Cadbury]

Hi! So I am solving this problem:

View attachment 3777

Find the solution that passes through (1,2)

First I tried substituting
x= u+1
y= v+2
dx = du
dy = dv to the equation but I cannot find the solution any help will be appreciated, thank you! :D

 

[Euge]

Note $2yy' = (y^2)'$, so by setting $u = y^2$, you may reduce your differential equation to the first order linear equation $xu' = u - 2x^3$, or

$$xu' - u = -2x^3.$$

Since you're looking for the solution that passes through $(1,2)$, you have an initial condition $y(1) = 2$. This translates to $u(1) = 4$, since $u = y^2$. Now solve the IVP

$$xu' - u = -2x^3,\quad u(1) = 4.$$

 

[Cadbury]

Note $2yy' = (y^2)'$, so by setting $u = y^2$, you may reduce your differential equation to the first order linear equation $xu' = u - 2x^3$, or

$$xu' - u = -2x^3.$$

Since you're looking for the solution that passes through $(1,2)$, you have an initial condition $y(1) = 2$. This translates to $u(1) = 4$, since $u = y^2$. Now solve the IVP

$$xu' - u = -2x^3,\quad u(1) = 4.$$

Thank you! :) :) :)