Solving a hard DE: R'(t)=k*4*pi*R(t)^2

[Mathman2013]

Homework Statement

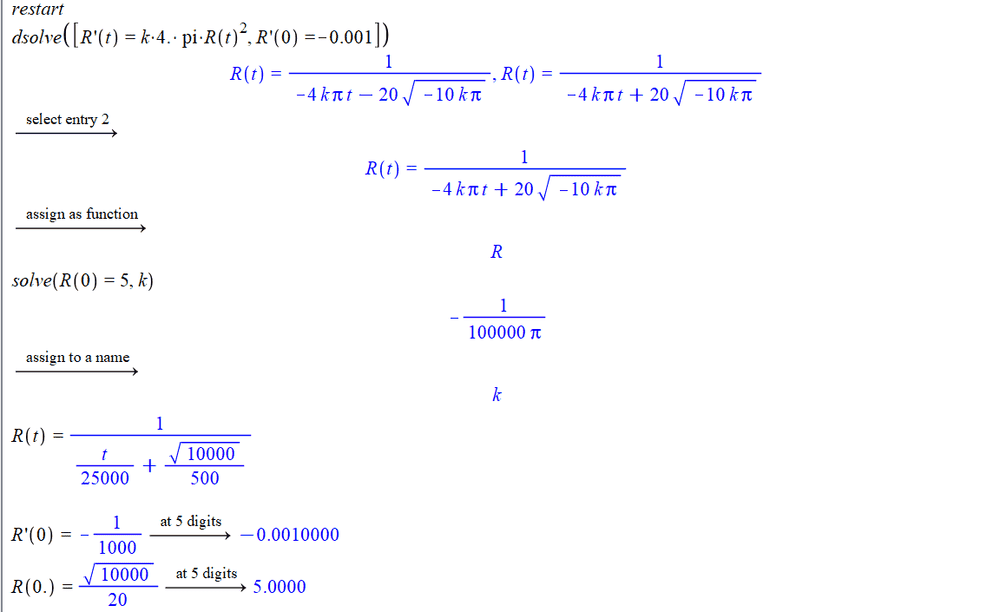
solving the following ODE (Hard)

Relevant Equations

R'(t)=k*4*pi*R(t)^2

I have the following DE, R'(t) = k*4*pi*R(t)^2, where R(0) = 5, and R'(0) =-0.001. I have attempted to solve it Maple, and would like to know if I have done it correctly?

 

[BvU]

would like to know if I have done it correctly

The way to check that you (Maple?) have done it correctly is to differentiate twice and see if it matches !
You don't need PF to do that for you, do you ?

[edit] and what about entry 1 ?
[edit2] Strike the 'twice' .

$\$

 

[Mathman2013]

The way to check that you (Maple?) have done it correctly is to differentiate twice and see if it matches !
You don't need PF to do that for you, do you ?

[edit] and what about entry 1 ?

$\$

I can't find k if I select entry 1.

 

[BvU]

How wold you go about if you had to solve this DE yourself ?

You mention 'hard' but it really isn't difficult ...

$\$

 

[Mark44]

The way to check that you (Maple?) have done it correctly is to differentiate twice and see if it matches !

The DE is first order, so the OP needs only to differentiate the solution function once.

 

[Frabjous]

This DE is solvable by hand. Notice that the original equation can be written as
dR/R^2 = Constant*dt

 

[BvU]

@mathman ?

 

[mathman]

@mathman ?

What are you asking?

 

[BvU]

How wold you go about if you had to solve this DE yourself ?

 

[Frabjous]

Wrong mathman. You want the 2013 edition.

 

[BvU]

Oops ! sorry.

 

[haushofer]

If this DE is hard, I wonder what this topic looks like when the variables are not separable :P

 

[BvU]

@Mathman2013 didn't reply to post #4