Solving an Equation: Overcoming the r's

[mhirschb]

I feel silly but I've been looking at this equation for a while and I don't fully understand the individual steps taken to go from the top line to the bottom line:

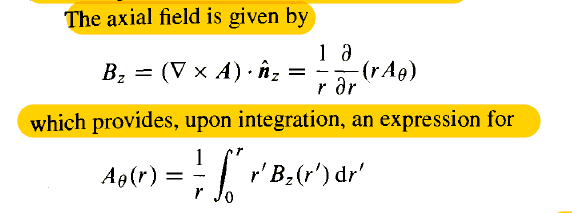
I think I am getting caught up with all the r's in the equation. I recognize that on the second line "r" describes the point at which we are evaluating the MVP, and r' is the domain of r that we're integrating over.
I'm confused because it looks like they've taken the 1/r and changed it to r' on the other side.

 

[pasmith]

The first equation obtains $B_z$ from $A_\theta$ bythe following steps:

• Multiply by $r$.
• Differentiate with respect to $r$.
• Divide by $r$.

Therefore $A_\theta$ is obtained from $B_z$ by reversing these steps:

• Multiply by $r$.
• Integrate with respect to $r$. This is done as a definite integral with lower limit 0, because any other choice of lower limit would make $A_\theta$ potentially infinite at $r = 0$. This definite integration requires the introduction of $r'$ as a dummy variable of integration, because it is bad practise to use $r$ as both a limit of the integral and the dummy variable of integration.
• Divide by $r$.

 

[mhirschb]

The first equation obtains $B_z$ from $A_\theta$ bythe following steps:

• Multiply by $r$.
• Differentiate with respect to $r$.
• Divide by $r$.

Therefore $A_\theta$ is obtained from $B_z$ by reversing these steps:

• Multiply by $r$.
• Integrate with respect to $r$. This is done as a definite integral with lower limit 0, because any other choice of lower limit would make $A_\theta$ potentially infinite at $r = 0$. This definite integration requires the introduction of $r'$ as a dummy variable of integration, because it is bad practise to use $r$ as both a limit of the integral and the dummy variable of integration.
• Divide by $r$.

Ah! It makes so much sense now I wanna facepalm!

Thank you for the explanation. It became clear when I needed to introduce the dummy variable r'.