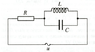
- #1
masterjoda
- 21
- 0
Homework Statement
Find the current in them in this circuit, if we know [itex]R=X_L, X_C[/itex] and [itex]u=5sin(314t)[/itex]
The Attempt at a Solution
First , [itex]5=U_0, 314=\omega[/itex] and voltage we can write as [itex]u=U_0cos(\omega t + \frac{\pi}{2})[/itex] and [itex]u=U_0 e^{i\frac{\pi}{2}}=iU_0[/itex]. [itex]U[/itex] is the voltage at the source [itex]U_1[/itex] in the branch and [itex]U_2[/itex] at the resistor. Now [itex]U=U_1+U_2[/itex] or [itex]U=IR+IZ[/itex] where [itex]\frac{1}{Z}=\frac{1}{iL\omega}-\frac{C\omega}{i}[/itex] or [itex]Z=\frac{iL\omega}{1-CL\omega^2}[/itex]
Now the current is [itex]I=\frac{U}{R+Z}[/itex] or when it is arranged [itex]I=\frac{iU_0(1-CL\omega^2)}{iL\omega (2-CL\omega^2)}[/itex]. Now I don't know how to complete the calculation to reduce it to the form like this [itex]I_0sin(\omega t + \theta)[/itex].