- #1
Svelte1
- 9
- 2
- Homework Statement
- Okay, I saw a derivation of work equaling a change in kinetic energy and it explicitly states that this is regarding the MAGNITUDE of the velocity vector, however in an example question the formula is used for the horizontal velocity component and I don't understand how this can be done, seems incorrect to me.
- Relevant Equations
- Work= change in potential energy=change in kinetic energy
https://ibb.co/jG6n0jZ
The 15 is fine as this is clearly his overall magnitude but then v2 is equated to the horizontal velocity rather than the magnitude.
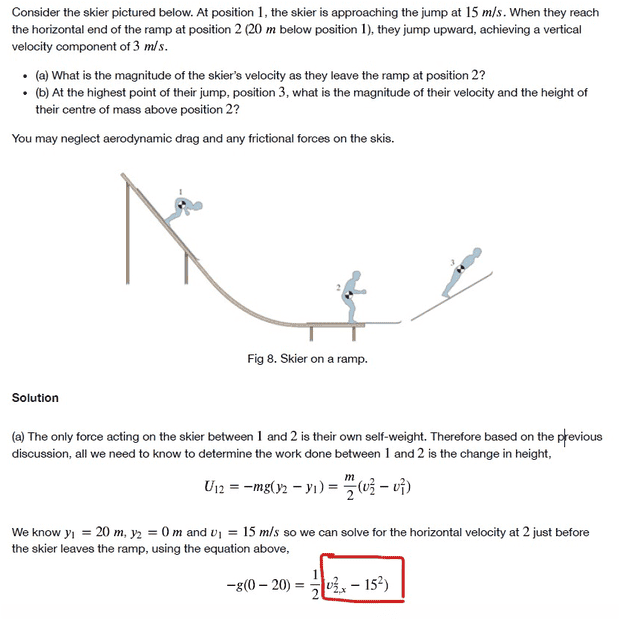
The 15 is fine as this is clearly his overall magnitude but then v2 is equated to the horizontal velocity rather than the magnitude.