Solving Implicit Function: Tangent Point & Level Curve Equation

In summary: View Full Size.In summary, the tangent point is at the first quarter and the equation of the level curve is y=8/x.
  • #1
Yankel
395
0
Hello all,

I need some help with this one, I do not have a clue how to even begin.

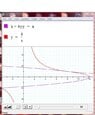
the level curve of

\[f(x,y)=x+4y^{2}\]

tangents the function

\[y=\frac{8}{x}\]

in a point at the first quarter. What is the tangent point, what is the equation of the level curve ?

This question need to involve implicit functions. I don't get it... :confused:

thanks !
 
Physics news on Phys.org
  • #2
Yankel said:
Hello all,

I need some help with this one, I do not have a clue how to even begin.

the level curve of

\[f(x,y)=x+4y^{2}\]

tangents the function

\[y=\frac{8}{x}\]

in a point at the first quarter. What is the tangent point, what is the equation of the level curve ?

This question need to involve implicit functions. I don't get it... :confused:

thanks !
A level curve of $f(x,y)$ is the set of points at which $f(x,y)$ takes a constant value $k$ say. So start with the equation $x+4y^2=k$, and differentiate it implicitly to get an expression for $y'.$ Next, differentiate $y=8/x$ to get another expression for $y'$. If the two curves are tangent to each other, then they must have the same value for $y'$ at that point. So put the two expressions for $y'$ equal to each other and you will get an equation for the point $(x,y)$. Use that together with the equation $y=8/x$ to find $x$ and $y$. Finally, use the equation $x+4y^2=k$ to find $k$.
 
  • #3
Thank you !

Did I do it correctly ?

the implicit derivative is:

\[\frac{dy}{dx}=-\frac{1}{8y}\]

The immediate derivative is:

\[y'=-\frac{8}{x^{2}}\]

leading to x=8, y=1 and k=12 ?

Thanks for your explanation, very helpful.
 
  • #4

Attachments

  • 23px-Yes_check.svg.png
    23px-Yes_check.svg.png
    309 bytes · Views: 69
  • #5

Attachments

  • Capture.JPG
    Capture.JPG
    38.6 KB · Views: 66
Last edited:

FAQ: Solving Implicit Function: Tangent Point & Level Curve Equation

What is an implicit function?

An implicit function is a mathematical representation of a relationship between two or more variables, where the dependent variable is not explicitly defined in terms of the independent variables. This means that the function cannot be easily solved for one of the variables, and instead, an equation is used to represent the relationship.

What is a tangent point?

A tangent point is a point on a curve where the slope of the curve is equal to the slope of a tangent line drawn at that point. In other words, the tangent line touches the curve at only one point and is parallel to the curve at that point.

How do you solve for the tangent point of an implicit function?

To solve for the tangent point, you must first find the derivative of the implicit function with respect to one of the variables. Then, set the derivative equal to the slope of the tangent line and solve for the variable. The resulting value will give you the x-coordinate of the tangent point. To find the y-coordinate, plug the x-coordinate into the original implicit function.

What is a level curve equation?

A level curve equation is a two-dimensional representation of a three-dimensional surface. It is an equation that describes all the points on a surface that have the same value for a given variable. These curves are often used to visualize and analyze functions with multiple variables.

How do you solve for the level curve equation of an implicit function?

To solve for the level curve equation, you must first set the implicit function equal to a constant value. Then, manipulate the equation to isolate one of the variables. This resulting equation will be the level curve equation for that specific constant value. To find other level curve equations, repeat this process with different constant values.

Similar threads

I How to evaluate the enclosed area of this implicit curve?
Replies
2
Views
2K
B Tangents to a curve as functions of different variables
Replies
5
Views
1K
B Confusion on Implicit Differentiation
Replies
16
Views
2K
I Gradient Vectors: Perpendicular to Level Curves
Replies
4
Views
2K
MHB Implicit Differentiation to find equation of a tangent line
Replies
4
Views
2K
MHB Another implicit function problem
Replies
2
Views
1K
I Approximation of a function of two variables
Replies
3
Views
1K
MHB Implicit function theorem for f(x,y) = x^2+y^2-1
Replies
1
Views
1K
MHB 205.2.6.1-2 another implicit problem with tangent line.
Replies
1
Views
1K
B Why does f = g in implicit equations?
Replies
8
Views
1K

Hot Threads

Recent Insights

Back
Top