musicgold
- 303
- 19
- Homework Statement
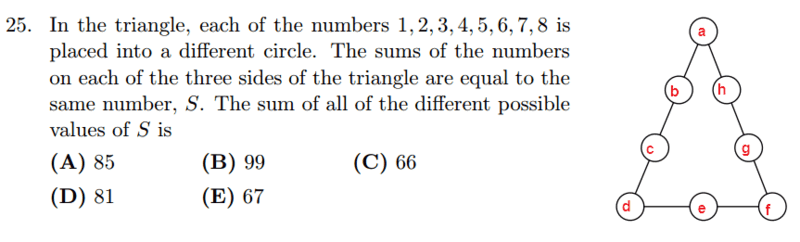
- I am trying to solve the puzzle shown below.
- Relevant Equations
- I could find the 3 equations shown below that appear to describe the situation.
First I tried to solve this with algebra, but there are not enough equations:
a+ b + c + d + e + f + g + h = 36
S = 12 + (d +f + a)/3 ........... ( d +f + a has to be a multiple of 3)
a + b + c = e + f
a + h + g = d + e
So I had to resort to the trial and error to find the solution below:
a, b, c, d : 6, 1, 5, 7
a, h, g, f : 6, 2, 3, 8
e : 4
Is there an elegant way / pattern to find other possible solutions?
Thanks
a+ b + c + d + e + f + g + h = 36
S = 12 + (d +f + a)/3 ........... ( d +f + a has to be a multiple of 3)
a + b + c = e + f
a + h + g = d + e
So I had to resort to the trial and error to find the solution below:
a, b, c, d : 6, 1, 5, 7
a, h, g, f : 6, 2, 3, 8
e : 4
Is there an elegant way / pattern to find other possible solutions?
Thanks
Last edited:

