phantomvommand
- 287
- 39
- Homework Statement
- Please refer to the picture below
- Relevant Equations
- Gauss's Law
Ampere's Law
I am only asking about part e. If you are short on time, you can read through parts a - d, to get an idea of what is happening, and then attempt part e directly.

I have solved parts a - d. If you would like to check your answers, the answer to part c is [rne^2 / 2e0] [ 1 - (v/c)^2], and the answer to part d is 0.
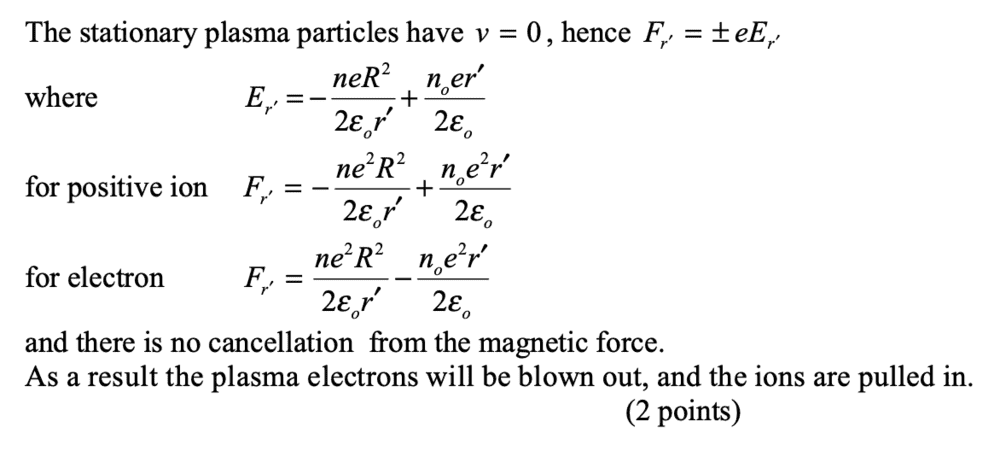
I do not understand the following solution to part e. The term on the left represents the E-field due to the electron beam, which I understand. I suppose the term on the right is the E-field due to the positively charged ions in the plasma. However, isn't the effect of the positively charged ions in the plasma negated by the effect of the negatively charged electrons in the plasma? Thus, the term on the right should not exist. What is wrong?
I have solved parts a - d. If you would like to check your answers, the answer to part c is [rne^2 / 2e0] [ 1 - (v/c)^2], and the answer to part d is 0.
I do not understand the following solution to part e. The term on the left represents the E-field due to the electron beam, which I understand. I suppose the term on the right is the E-field due to the positively charged ions in the plasma. However, isn't the effect of the positively charged ions in the plasma negated by the effect of the negatively charged electrons in the plasma? Thus, the term on the right should not exist. What is wrong?