qft-El
- 11
- 13
- TL;DR Summary
- I've run into this books claiming to solve the RG equation via the method of characteristics. There is a passage I don't quite understand and the method looks different from the usual method of characteristics.
I'm learning about the RG equation and Callan-Symanzik equation. In ref.1 they claim to solve the RG equation via the method of characteristics for PDE. Here's a picture of the relevant part:
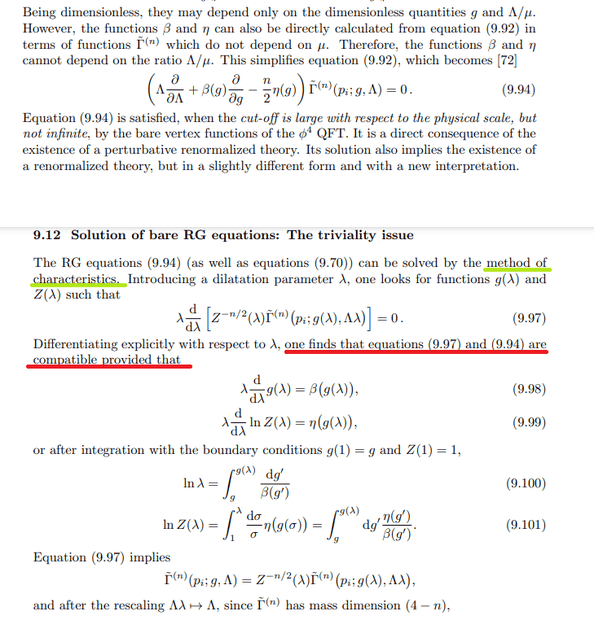
First, the part I don't understand - the one underlined in red. What does "compatible" mean here? Upon explicit differentiation one gets
$$Z_{\text{eff}}^{-n/2}(\lambda)\left[\Lambda\frac{\partial}{\partial\Lambda}+\lambda\frac{d}{d\lambda}g_\text{eff}(\lambda)\frac{\partial}{\partial g_\text{eff}}-n\cdot\frac{1}{2}\lambda\frac{d}{d\lambda}Z_\text{eff}(\lambda)\right]\Gamma_0(p_i;g_\text{eff}(\lambda),\lambda\Lambda)=0\tag{9.97a}.$$
Even if "compatible" just meant that they don't contradict each other, I don't see why ##(9.98-9.99)## specifically should hold. If anything, the choice doesn't seem unique, does it?
Secondly, I'm unsure about the method of characteristics here: it looks very different from the standard method I know (see this question on Physics.SE). I have never seen this dilatation parameter anywhere else, except other books having ref.1 as a reference, is there any source you can advise?
By the way, the independent variables should be ##g## and ##\Lambda## in eq. ##(9.94)##, so I guess that once one writes it down, we disregard that ##g=g(\Lambda)## - used to derive the equation and regard it as an independent variable, right?
See this question on Physics.SE for more context.
References
First, the part I don't understand - the one underlined in red. What does "compatible" mean here? Upon explicit differentiation one gets
$$Z_{\text{eff}}^{-n/2}(\lambda)\left[\Lambda\frac{\partial}{\partial\Lambda}+\lambda\frac{d}{d\lambda}g_\text{eff}(\lambda)\frac{\partial}{\partial g_\text{eff}}-n\cdot\frac{1}{2}\lambda\frac{d}{d\lambda}Z_\text{eff}(\lambda)\right]\Gamma_0(p_i;g_\text{eff}(\lambda),\lambda\Lambda)=0\tag{9.97a}.$$
Even if "compatible" just meant that they don't contradict each other, I don't see why ##(9.98-9.99)## specifically should hold. If anything, the choice doesn't seem unique, does it?
Secondly, I'm unsure about the method of characteristics here: it looks very different from the standard method I know (see this question on Physics.SE). I have never seen this dilatation parameter anywhere else, except other books having ref.1 as a reference, is there any source you can advise?
By the way, the independent variables should be ##g## and ##\Lambda## in eq. ##(9.94)##, so I guess that once one writes it down, we disregard that ##g=g(\Lambda)## - used to derive the equation and regard it as an independent variable, right?
See this question on Physics.SE for more context.
References
- Quantum Field Theory and Critical Phenomena, Jean Zinn-Justin, 2021, fifth edition. Section 9.11.2-9.12, eqs. ##(9.91-9.101)##.