- #1
DivGradCurl
- 372
- 0
Folks, I have a problem in my calculus textbook about the Wallis Product
[tex]\frac{\pi}{2} = \frac{2}{1} \cdot \frac{2}{3} \cdot \frac{4}{3} \cdot \frac{4}{5} \cdot \frac{6}{5} \cdot \frac{6}{7} \cdot \cdots[/tex]
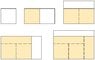
"We construct rectangles as follows. Start with a square of area 1 and attach rectangles of area 1 alternately beside or on top of the previous rectangle (see figure). Find the limit of the ratios of width to height of these rectangles."
Here's what I've got:
[tex]R_1 = \frac{W_1}{H_1} = \frac{1}{1} = 1[/tex]
[tex]R_2 = \frac{W_2}{H_2} = \frac{W_1 + \frac{1}{H_2}}{H_1} = \frac{2}{1} = 2[/tex]
[tex]R_3 = \frac{W_3}{H_3} = \frac{W_2}{H_2 + \frac{1}{W_3}} = \frac{2}{3/2} = \frac{4}{3}[/tex]
[tex]R_4 = \frac{W_4}{H_4} = \frac{W_3 + \frac{1}{H_4}}{H_3} = \frac{8/3}{3/2} = \frac{16}{9}[/tex]
[tex]R_5 = \frac{W_5}{H_5} = \frac{W_4}{H_4 + \frac{1}{W_5}} = \frac{8/3}{15/8} = \frac{64}{45}[/tex]
Since all previous parts of this problem are about the Wallis Product, it would not be surprising to find out that the limit of the ratios of width to height of those rectangles is [tex]\frac{\pi}{2}[/tex]. It doesn't seem to be appropriate to simply state it, though.
If [tex]n[/tex] is even, then
[tex]W_n = W_{n-1} + \frac{1}{H_n}[/tex] and [tex]H_n = H_{n-1}[/tex]
and so
[tex]R_n = \frac{W_n}{H_n} = \frac{W_{n-1} + \frac{1}{H_n}}{H_{n-1}}[/tex]
If [tex]n[/tex] is odd, then
[tex]W_n = W_{n-1}[/tex] and [tex]H_n = H_{n-1} + \frac{1}{W_n}[/tex]
and so
[tex]R_n = \frac{W_n}{H_n} = \frac{W_{n-1}}{H_{n-1} + \frac{1}{W_n}}[/tex]
Thus, we find
[tex]\lim _{n\to \infty} \frac{W_{n-1} + \frac{1}{H_n}}{H_{n-1}} = \lim _{n\to \infty} \frac{W_{n-1}}{H_{n-1} + \frac{1}{W_n}}[/tex]
However, I don't know how to proceed so that I find
[tex]\lim _{n\to \infty} R_n = \frac{\pi}{2}[/tex]
Any help is highly appreciated. Thanks.
PS: THE FIGURE IS ATTACHED.
[tex]\frac{\pi}{2} = \frac{2}{1} \cdot \frac{2}{3} \cdot \frac{4}{3} \cdot \frac{4}{5} \cdot \frac{6}{5} \cdot \frac{6}{7} \cdot \cdots[/tex]
"We construct rectangles as follows. Start with a square of area 1 and attach rectangles of area 1 alternately beside or on top of the previous rectangle (see figure). Find the limit of the ratios of width to height of these rectangles."
Here's what I've got:
[tex]R_1 = \frac{W_1}{H_1} = \frac{1}{1} = 1[/tex]
[tex]R_2 = \frac{W_2}{H_2} = \frac{W_1 + \frac{1}{H_2}}{H_1} = \frac{2}{1} = 2[/tex]
[tex]R_3 = \frac{W_3}{H_3} = \frac{W_2}{H_2 + \frac{1}{W_3}} = \frac{2}{3/2} = \frac{4}{3}[/tex]
[tex]R_4 = \frac{W_4}{H_4} = \frac{W_3 + \frac{1}{H_4}}{H_3} = \frac{8/3}{3/2} = \frac{16}{9}[/tex]
[tex]R_5 = \frac{W_5}{H_5} = \frac{W_4}{H_4 + \frac{1}{W_5}} = \frac{8/3}{15/8} = \frac{64}{45}[/tex]
Since all previous parts of this problem are about the Wallis Product, it would not be surprising to find out that the limit of the ratios of width to height of those rectangles is [tex]\frac{\pi}{2}[/tex]. It doesn't seem to be appropriate to simply state it, though.
If [tex]n[/tex] is even, then
[tex]W_n = W_{n-1} + \frac{1}{H_n}[/tex] and [tex]H_n = H_{n-1}[/tex]
and so
[tex]R_n = \frac{W_n}{H_n} = \frac{W_{n-1} + \frac{1}{H_n}}{H_{n-1}}[/tex]
If [tex]n[/tex] is odd, then
[tex]W_n = W_{n-1}[/tex] and [tex]H_n = H_{n-1} + \frac{1}{W_n}[/tex]
and so
[tex]R_n = \frac{W_n}{H_n} = \frac{W_{n-1}}{H_{n-1} + \frac{1}{W_n}}[/tex]
Thus, we find
[tex]\lim _{n\to \infty} \frac{W_{n-1} + \frac{1}{H_n}}{H_{n-1}} = \lim _{n\to \infty} \frac{W_{n-1}}{H_{n-1} + \frac{1}{W_n}}[/tex]
However, I don't know how to proceed so that I find
[tex]\lim _{n\to \infty} R_n = \frac{\pi}{2}[/tex]
Any help is highly appreciated. Thanks.
PS: THE FIGURE IS ATTACHED.