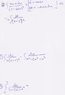- #1
Solving Tricky Integrals: What Technique to Use?
- Thread starter asdnator
- Start date
-
- Tags
- Integrals
In summary, the conversation discusses the best technique to use when solving integrals. The suggested technique is the Feynman technique, which involves writing down the problem, thinking critically, and writing down the solution. It is also advised to attempt the problem and consult notes and resources before seeking help. It is important to check the solution by taking the derivative.
- #2
vela
Staff Emeritus
Science Advisor
Homework Helper
Education Advisor
- 16,095
- 2,742
Use the Feynman technique:asdnator said:
- Write down the problem.
- Think really hard.
- Write down the solution.
- #3
vela
Staff Emeritus
Science Advisor
Homework Helper
Education Advisor
- 16,095
- 2,742
For #5, there's an obvious substitution to try.
For the remaining two, look in your notes and book for techniques to try and take a stab at them. You have to show a reasonable attempt before you can get help here.
For the remaining two, look in your notes and book for techniques to try and take a stab at them. You have to show a reasonable attempt before you can get help here.
- #4
- #5
Mark44
Mentor
- 37,788
- 10,180
You can check it yourself. Take the derivative of your answer. If it's correct, you should end up with the integrand. This is something you should make a habit of doing.asdnator said:
FAQ: Solving Tricky Integrals: What Technique to Use?
What are some common techniques for solving tricky integrals?
Some common techniques for solving tricky integrals include substitution, integration by parts, partial fractions, and trigonometric identities.
How do I know which technique to use for a specific integral?
The best way to determine which technique to use is by analyzing the integrand and looking for patterns or familiar forms. For example, if the integrand contains a trigonometric function, you may want to use a trigonometric identity. If the integrand is a product of two functions, integration by parts may be the best approach.
What should I do if none of the common techniques seem to work?
If none of the common techniques are successful, you can try manipulating the integrand by using algebraic properties or breaking it down into smaller parts. You can also consult a math textbook or seek help from a tutor or professor.
Are there any shortcuts for solving tricky integrals?
While there are no shortcuts for solving tricky integrals, practicing and becoming familiar with different techniques can make the process easier and quicker. Additionally, memorizing common integrals and their solutions can save time when encountering them in future problems.
How can I check if my integral solution is correct?
You can check your integral solution by differentiating it and seeing if the result matches the original integrand. You can also use online integral calculators or ask for feedback from a math tutor or professor.