member 731016
- Homework Statement
- Please see below
- Relevant Equations
- Please see below
For this problem,
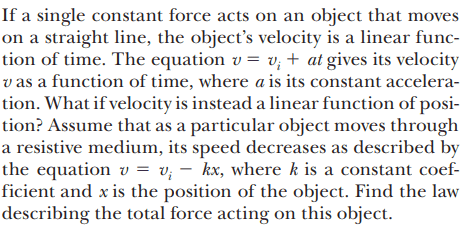
My working is
## \vec F = m\vec a##
## \vec F = \frac {m(v - v_i)}{\Delta t} ##
## \vec F = \frac {-mkx}{\Delta t} ##
However, the solution said ##\vec F = -mkv ##. I don't understand how this is possible since ## v ≠\frac {x}{\Delta t} ## since ##v =\frac {\Delta x}{\Delta t} ##?
Many thanks!
My working is
## \vec F = m\vec a##
## \vec F = \frac {m(v - v_i)}{\Delta t} ##
## \vec F = \frac {-mkx}{\Delta t} ##
However, the solution said ##\vec F = -mkv ##. I don't understand how this is possible since ## v ≠\frac {x}{\Delta t} ## since ##v =\frac {\Delta x}{\Delta t} ##?
Many thanks!