- #1
Wen
- 44
- 0
Here are some physics question i encounter in a book for physics olympiad. I couldn't work them out.
Questions:
1)
A particle moves along a straight line ABC with B
being the midpoint between A and C. The acceleration
of the particle from A to B is constant at a1, while
its acceleration from B to C is constant at a2. Given
that
vB = (vA + vC) / 2
where vA, vB and vC are the velocities of the particle
at points A, B and C respectively, compare the
magnitudes of a1 and a2.
2)
Two points A and B are a distance s apart along a
straight line. Distance s is divided into n equal
sections, such that each section is a distance s/n
long. A stationary particle starts traveling from A
such that its inital acceleration is a constant a
along the first section. At the end of each section,
the acceleration of the particle increases by a/n,
until the particle moves past B. Find the velocity vB
of the particle after it has moved past point B.
3)
Problem 1
A particle traveled half of a certain distance with a
velocity v0. The remaining part of the distance was
covered with velocity v1 for half the time, and with
velocity v2 for the other half of the time. Find the
mean velocity of the particle over the entire distance
travelled?
Problem 2
A uniform ladder of mass m1 and length 2l rests
against a smooth vertical wall with the foot of the
ladder placed on a rough horizontal floor some
distance away from the bottom of the wall. The
coefficient of static friction between the foot of the
ladder and the floor is ?, and a man of mass m2 climbs
a distance l1 up the ladder from the bottom.
(a) If the ladder makes an acute angle ? with the
floor, how far can the man climb without the ladder
slipping?
(b) If the man is to climb a distance l2 from the foot
of the ladder, what angle should the ladder make with
the horizontal floor?
4)
boat is rowed from A across the river. If the boat is
always pointing in the direction perpendicular to the
river, it will eventually reach C on the opposite bank
in 10 min. If the rower wants to reach point B
directly opposite A instead, he has to point his boat
in the direction towards D when he set off from A, and
maintain that direction as he rows across. In this
case he will take 12.5 min to cross the river.
Given that BC is 120 m, find
(a) the boat's speed v relative to the river;
(b) the river's width L;
(c) the speed u of river flow; and
(d) the angle ? between AD and AB.
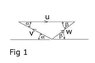
5)
Problem 1
A boat can travel at a speed of 3 m s-1 on still
water. A boatman wants to cross a river whilst
covering the shortest possible distance. In what
direction should he row with respect to the bank if
the speed of the water is
(i) 2 m s-1,
(ii) 4 m s-1?
Assume that the speed of the water is the same
everywhere.
Problem 2
The suspension springs of all four wheels of a car are
identical. By how much does the body of a car
(considered rigid) rise above each of the wheels when
its right front wheel is parked on an 8-cm-high
pavement? Does the result change when the car is
parked with both right wheels on the pavement? Does
the result depend on the number and positions of the
people sitting in the car?
Questions:
1)
A particle moves along a straight line ABC with B
being the midpoint between A and C. The acceleration
of the particle from A to B is constant at a1, while
its acceleration from B to C is constant at a2. Given
that
vB = (vA + vC) / 2
where vA, vB and vC are the velocities of the particle
at points A, B and C respectively, compare the
magnitudes of a1 and a2.
2)
Two points A and B are a distance s apart along a
straight line. Distance s is divided into n equal
sections, such that each section is a distance s/n
long. A stationary particle starts traveling from A
such that its inital acceleration is a constant a
along the first section. At the end of each section,
the acceleration of the particle increases by a/n,
until the particle moves past B. Find the velocity vB
of the particle after it has moved past point B.
3)
Problem 1
A particle traveled half of a certain distance with a
velocity v0. The remaining part of the distance was
covered with velocity v1 for half the time, and with
velocity v2 for the other half of the time. Find the
mean velocity of the particle over the entire distance
travelled?
Problem 2
A uniform ladder of mass m1 and length 2l rests
against a smooth vertical wall with the foot of the
ladder placed on a rough horizontal floor some
distance away from the bottom of the wall. The
coefficient of static friction between the foot of the
ladder and the floor is ?, and a man of mass m2 climbs
a distance l1 up the ladder from the bottom.
(a) If the ladder makes an acute angle ? with the
floor, how far can the man climb without the ladder
slipping?
(b) If the man is to climb a distance l2 from the foot
of the ladder, what angle should the ladder make with
the horizontal floor?
4)
boat is rowed from A across the river. If the boat is
always pointing in the direction perpendicular to the
river, it will eventually reach C on the opposite bank
in 10 min. If the rower wants to reach point B
directly opposite A instead, he has to point his boat
in the direction towards D when he set off from A, and
maintain that direction as he rows across. In this
case he will take 12.5 min to cross the river.
Given that BC is 120 m, find
(a) the boat's speed v relative to the river;
(b) the river's width L;
(c) the speed u of river flow; and
(d) the angle ? between AD and AB.
5)
Problem 1
A boat can travel at a speed of 3 m s-1 on still
water. A boatman wants to cross a river whilst
covering the shortest possible distance. In what
direction should he row with respect to the bank if
the speed of the water is
(i) 2 m s-1,
(ii) 4 m s-1?
Assume that the speed of the water is the same
everywhere.
Problem 2
The suspension springs of all four wheels of a car are
identical. By how much does the body of a car
(considered rigid) rise above each of the wheels when
its right front wheel is parked on an 8-cm-high
pavement? Does the result change when the car is
parked with both right wheels on the pavement? Does
the result depend on the number and positions of the
people sitting in the car?