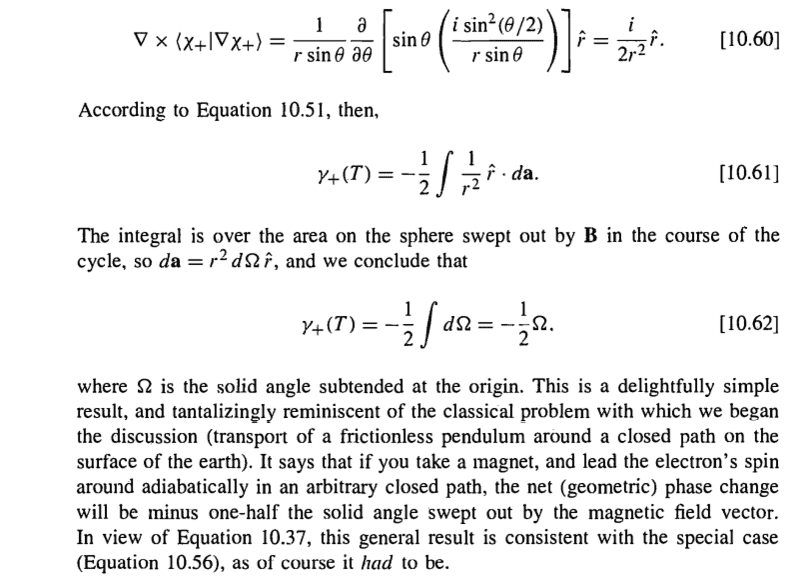
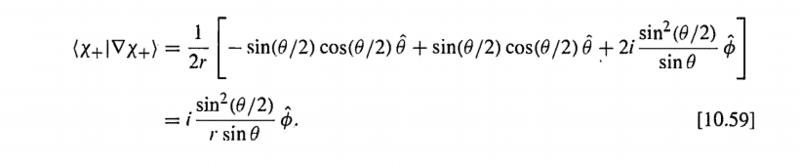- #1
heslaheim
- 1
- 0
- TL;DR Summary
- How to calculate the integral of a loop when the surface integrals of its curl are different in the area of different surface?
A certain field has a singularity at the origin, and the divergence of its curl is zero at any point outside the origin, but surface integral of the curl is not zero in the area of any closed surface containing the origin. So how should the Stokes theorem related to this field be expressed at this time?