Something I noticed about Doppler asymmetry

[JohnnyGui]

Good day,

I was playing a bit with a Doppler effect scenario and tried to combine it a bit with Special Relativity and I concluded something that I don’t quite understand, or that I at least don’t get the meaning of. I am aware that the scenario and my concluded formula don’t describe the full Relativistic Doppler effect but the result got me interested.

The scenario is the following:

Person A is moving away from Person B. Person B is holding a lasergun that emits a specific frequency of light pulses towards A.

Because of Special Relativity, Person A who is moving away would still measure the light pulses approaching him at c and he would think that Person B with the lasergun is moving away from him instead. From the Doppler asymmetry, Person A would therefore conclude that the time duration t between each light pulse that he’d receive is calculated by:

P x (1 + v/c) = t (Lightsource moving away)

In which P is de original time period between the light pulses (1 / f) that Person B is shooting.

However, Person B with the lasergun measures the light pulses going away from him at c and think that Person A is moving away from him. From the Doppler asymmetry, he’d think that person A would measure a time duration t’ between his light pulses which is calculated by:

P / (1- v/c) = t’ (Observer is moving away)

Out of curiosity, I then wanted to calculate the factor by which t and t’ are separated by calculating the ratio of these 2 formulas:

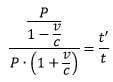
This simplifies to:

What got me surprised, is that this concluded ratio of t’ and t is equal to the Lorentz factor squared. However, I don’t get exactly why this is the case. Is this just coincidence or does it have a meaning and if so, what’s the meaning behind it? From what I understand, time dilation between 2 observers should be always separated merely by the Lorentz factor, not the square of it.

I’m very new to this so could someone please enlighten me on this in a simple way?

 

[Janus]

In neither of your scenarios did you account for time dilation. In the first scenario, you have to apply the time dilation along with applying the Doppler effect.
In other words, if P is the time between pulses as measured by B, Then
$$\frac{P}{\sqrt{1-\frac{v^2}{c^2}}}$$
will be the time between pulses emitted by B according to A. Applying the your Doppler shift expression to this will give the time between pulses measured by A, This will work out to.
$$P \sqrt{\frac{1+\frac{v}{c}}{1-\frac{v}{c}}}$$

In the second scenario, According to B, A's clock is running slow by the Lorentz factor, and so while B measures the pulses striking A by the time period your equation gave, he wouldn't expect A to measure that same time period due to A's slower running clock.

When you factor this in, you end up with the same answer as above as to the time period measured by A between received pulses. A and B must agree as to how much time A measures measures between pulses by his clock.
Time dilation and Doppler shift are two different effects and the reason you got the answer you got was due to neglecting one of them in both scenarios.

 

[JohnnyGui]

@Janus : Thanks for your explanation. Before delving into the Doppler factor, I have a question about the Lorentz factor alone:

. A and B must agree as to how much time A measures measures between pulses by his clock.

I find this weird. Because, if B is looking at the clock of A, he would say that A's clock is running slower and thus B's own P should get slowed down with the factor P / y (y being the Lorentz factor; 1 / √(1-v²/c²) to calculate the time period for A according to B. Thus, B says that A's time period is P / y.

At the same time, if A is looking at the clock of B, A would say that B's clock is running slower and thus the P that B measures, should be multiplied by the gamma factor to correct for the faster moving clock of A. Thus, A would say that his own time period between the lightpulses is P x y.

This means that B and A don't agree on A's time period (B says A's time period is P / y, A says his own time period is P x y). What am I doing wrong here?

Note that I'm putting the Doppler effect factor aside for now. Just want to understand this first.

 

[stevendaryl]

Yes, if you don't include time-dilation for the moving observer, then the Doppler shift measured by the moving observer for signals sent by the stationary observer is:

$f_1 = 1-\frac{v}{c}$

while the Doppler shift measured by the stationary observer for signals sent by the moving observer is:

$f_2 = \frac{1}{1+\frac{v}{c}}$

with the ratio being $\frac{f_2}{f_1} = \frac{1}{1-\frac{v^2}{c^2}}$

So without time dilation, you could tell who was "really" moving by looking at the Doppler shift.

Now, let's go through the same calculation including an unknown time dilation factor $g$.

The rate that the moving observer will measure signals will be greater because he's using a slowed clock. So instead of measuring $f_1$ above, he'll measure $f_1' = g f_1$

If the moving observer's clock is running slow, then the rate at which he will produce signals will be smaller by the factor of $g$. So the rate measured by the stationary observer will be, not $f_2$, but $f_2' = f_2/g$. Now, the ratio of the two modified rates will be:

$\frac{f_2'}{f_1'} = \frac{f_2/g}{g f_1} = \frac{1}{g^2} \frac{f_2}{f_1} = \frac{1}{g^2} \frac{1}{1-\frac{v^2}{c^2}}$

So if you choose $g = \frac{1}{\sqrt{1-\frac{v^2}{c^2}}}$, then the two modified frequencies will be the same, and there will be no way to tell who is moving.

So the principle of relativity (there is no absolute notion of "moving" versus "stationary") together with the classical Doppler shift implies the relativistic time dilation factor.

 

[JohnnyGui]

@stevendaryl : Thanks for your clear explanation. I've got a question:

So without time dilation, you could tell who was "really" moving by looking at the Doppler shift

Isn't this only true if either the observer (person A) or the laser shooter (person B) knows the other person's frequency in which he receives the light pulses? So, for example, if person B somehow knows the frequency that A receives his light pulses in, then person B can measure his own f_B and compare that with f_A that he heard from person A. If the ratio is 1 - v/c, then B knows that he's the one standing still. If B calculates a ratio of 1 / (1 + v/c), than B knows that he's the one moving.

Now, suppose neither A or B knows each other's frequency. Person B, as the laser shooter has one extra backup to decide who's moving; he has to measure the speed of the light pulses that he himself is shooting towards A. If he measures them being >c, then B knows that he's the one moving in the opposite direction.

The problem is however for person A if he doesn't know the frequency of B shooting his lasers according to B himself. That's because if A is standing still and B is moving away, A would see the light pulses coming at himself at c. But if A is moving away from B, A would still see the light pulses coming at him at c. So there's no way A would know who is moving other than knowing the frequency that B measures for his light pulses. But what if A doesn't know f_B?
Wouldn't A then think he's the one standing still and use the Doppler factor 1 / (1 + v/c) to calculate the frequency that B would measure?

In this case, if B is the one standing still, B would use 1 - v/c to calculate f_A and A would use 1 / (1 + v/c) to calculate f_B. Would this also cause a difference in time registration since A doesn't know who's moving? Or would this be merely an error coming from A?

 

[jartsa]

Yes, if you don't include time-dilation for the moving observer, then the Doppler shift measured by the moving observer for signals sent by the stationary observer is:

$f_1 = 1-\frac{v}{c}$

while the Doppler shift measured by the stationary observer for signals sent by the moving observer is:

$f_2 = \frac{1}{1+\frac{v}{c}}$

No no, the first formula is good for approaching observers, while the second formula is good for receding observers. (EDIT: There's an error, as shift happens to the wrong direction, but the basic idea is that at high speeds blueshifts get huge, while redshifts don't)

Here I made the error of using the first formula when the second one should have been used:
https://www.physicsforums.com/threads/relativistic-photonic-rocket.882298/#post-5547057

 

[stevendaryl]

No no, the first formula is good for approaching observers, while the second formula is good for receding observers

That's not correct. They are both for the receding case.

Suppose that Alice is stationary and Bob is moving at speed $v$. Then the only difference between the receding case and the approaching case is the sign of $v$. $v > 0$ means Bob is receding, $v < 0$ means that he is approaching. Suppose both Alice and Bob are sending signals to each other at the rate of 1 signal per second. Then let

$f_1 =$ the rate at which Bob receives signals from Alice.
$f_2 =$ the rate at which Alice receives signals from Bob.If Bob is receding from Alice, then

$f_1 = 1-\frac{v}{c}$
$f_2 = \frac{1}{1+\frac{v}{c}}$

Note: if Bob is receding at speed $c$, then the rate at which he receives signals from Alice goes to zero.

If Bob is approaching Alice, then

$f_1 = 1+\frac{v}{c}$
$f_2 = \frac{1}{1-\frac{v}{c}}$

In this case, if Bob is approaching at speed $c$, then the rate at which Alice receives signals from Bob goes to infinity.

Let's go through the math. Suppose that Alice is at rest and Bob is moving away from Alice, at speed $v$. Alice sends light signals toward Bob once per second. Let $t_{sn}$ be the time at which the nth signal is sent, and $t_{rn}$ be the time at which the nth signal is received. Let $x_{sn}$ be Bob's distance from Alice at time $t_{sn}$ and let $x_{rn}$ be Bob's distance at time $t_{rn}$. Then we have, since Bob is traveling at speed $v$:

$x_{rn} = x_{sn} + v (t_{rn} - t_{sn})$

Since the signal travels at speed $c$, we have:

$x_{rn} = c (t_{rn} - t_{sn})$

So $c(t_{rn} - t_{sn}) = x_{sn} + v(t_{rn} - t_{sn}) \Rightarrow t_{rn} = t_{sn} + \frac{x_{sn}}{c-v}$

So signal number $n$ arrives at time $t_{sn} + \frac{x_{sn}}{c-v}$. Similarly, signal number $n+1$ arrives at time $t_{s(n+1)} + \frac{x_{s(n+1)}}{c-v}$. The time between them is:

$\delta t = t_{s(n+1)} - t_{sn} + \frac{x_{s(n+1)} - x_{sn}}{c-v}$

Now, since the time between Alice's signals is 1 second, and Bob is traveling at speed $v$:

$t_{s(n+1)} - t_{sn} = 1$
$x_{s(n+1)} - x_{sn} = v (t_{s(n+1)} - t_{sn}) = v$

So
$\delta t = 1 + \frac{v}{c-v} = \frac{1}{1-\frac{v}{c}}$

The frequency is $f_1 = \frac{1}{\delta t} = 1 - \frac{v}{c}$

Now, consider signals sent by Bob to Alice. Bob sends the nth signal at time $t_{sn}$, when he is at location $x_{sn}$. It arrives at time $t_{rn} = t_{sn} + \frac{x_{sn}}{c}$. The next signal is sent at time $t_{s(n+1)}$ and arrives at time $t_{s(n+1)} + \frac{x_{s(n+1)}}{c}$. So the time between signals is:

$\delta t = t_{r(n+1)} - t_{rn} = (t_{s(n+1)} - t_{sn}) + \frac{x_{s(n+1)} - x_{sn}}{c}$

As with the case of Alice sending, $t_{s(n+1)} - t_{sn} = 1$, $x_{s(n+1)} - x_{sn} = v$. So

$\delta t = 1 + \frac{v}{c}$

The frequency is $f_2 = \frac{1}{\delta t} = \frac{1}{1+\frac{v}{c}}$

 

[jartsa]

Note: if Bob is receding at speed cc, then the rate at which he receives signals from Alice goes to zero.

I have not studied all that math, but let's say a light pulse hits a slightly reflecting panel every light second. Time between reflection blinks will be 2 seconds, when the pulse is moving away from an observer that is at rest relative to the panels.

Isn't that a thing moving at c, and sending signals?

 

[stevendaryl]

I have not studied all that math, but let's say a light pulse hits a slightly reflecting panel every light second. Time between reflection blinks will be 2 seconds, when the pulse is moving away from an observer that is at rest relative to the panels.

Isn't that a thing moving at c, and sending signals?

Sorry, I don't understand your scenario. But the important cases for the $v \rightarrow c$ limit:

• If Bob is moving away from Alice at speed $c$, then he will NEVER receive any signals from Alice. So the rate at which he receives messages is zero.
• If Bob is moving toward Alice at speed $c$, then that means that the signals he sends all arrive at the same time. (He keeps up with the signals he sends, so the second signal occurs right on top of the first signal. And similarly for the third, etc.) So the rate that Alice receives the messages is infinity.

 

[jartsa]

If Bob is moving away from Alice at speed cc, then he will NEVER receive any signals from Alice. So the rate at which he receives messages is zero.

It may sound odd, but I think Bob will receive signals in that case, if we ask Bob. Let me try to explain:

Waves In medium, speed means speed relative to the medium:

When Bob is moving away from Alice at speed of sound, Bob will never receive any words from Alice. "I move so fast that sound waves can't catch me", says Bob.

When Alice is moving away from Bob at speed of sound, then Bob will receive signals at half the rate that Alice sends them.

EM-waves In vacuum, we pretend time dilation does not exist:

When Alice is moving away from Bob at speed of light, then Bob will receive signals at half the rate that Alice sends them.

When Bob is moving away from Alice at speed of light, then Bob will receive signals at half the rate that Alice sends them - if we ask Bob. Surely Bob will not say: "I move so fast that light can't catch me". And as Bob is the observer here, Bob's views are the important ones.

 

[stevendaryl]

It may sound odd, but I think Bob will receive signals in that case, if we ask Bob. Let me try to explain:

Waves In medium, speed means speed relative to the medium:

When Bob is moving away from Alice at speed of sound, Bob will never receive any words from Alice. "I move so fast that sound waves can't catch me", says Bob.

It doesn't have anything necessarily to do with a medium. We're just assuming that as measured by Alice, both her signals and Bob's signals travel at the same speed $c$ in either direction. You could invoke a medium for the purpose of explaining WHY the signals travel at speed $c$, but for the purposes of computing the Doppler shift, why doesn't matter.

When Alice is moving away from Bob at speed of sound, then Bob will receive signals at half the rate that Alice sends them.

My calculations were all done in the frame in which Alice is at rest and (by assumption) the signals travel at speed $c$.

So to make everything explicit: We are assuming that there is a frame $F_A$ and an associated inertial coordinate system such that:

1. Alice is at rest.
2. Bob is traveling at speed $v$ (positive means away from Alice, negative means towards Alice)
3. Alice and Bob both send signals at rate one per second.
4. The signals travel at speed $c$ regardless of direction or who sent them.

So according to the coordinate system of frame $F_A$,

• Bob receives signals from Alice at the rate $f_1 = 1-\frac{v}{c}$
• Alice receives signals from Bob at the rate $f_2 = \frac{1}{1+\frac{v}{c}}$

This is not taking time dilation into account. To do that, we modify the assumptions as follows:

• According to frame $F_A$, Alice still sends at a rate of 1 signal per second, but Bob sends at a rate of $\frac{1}{g}$ signals per second.
• Bob's measured rate for signals received from Alice will be greater than the rate measured by frame $F_A$ by a factor of $g$

With these modifications,

• Bob's measured rate for receiving signals from Alice is: $f_1' = g(1-\frac{v}{c})$
• Alice's measured rate for receiving signals from Bob is: $f_2' = \frac{1}{g} \frac{1}{1+\frac{v}{c}}$

The choice of $g = \frac{1}{\sqrt{1-\frac{v^2}{c^2}}}$ makes these two rates identical: $f_1' = f_2' = \frac{\sqrt{1-\frac{v}{c}}}{\sqrt{1+\frac{v}{c}}}$

 

[jartsa]

OK. Maybe I got the idea now

 

[JohnnyGui]

@stevendaryl : Sorry for interrupting your explanation to jartsa but can you please asnwer my question in my post #5? Still not sure about it.

The choice of g=1√1−v2c2g=11−v2c2g = \frac{1}{\sqrt{1-\frac{v^2}{c^2}}} makes these two rates identical: f′1=f′2=√1−vc√1+vcf1′=f2′=1−vc1+vcf_1' = f_2' = \frac{\sqrt{1-\frac{v}{c}}}{\sqrt{1+\frac{v}{c}}}

Don't you mean that the ratio of f1 and f2 is that formula? The wiki says the following:

 

[stevendaryl]

@stevendaryl : Sorry for interrupting your explanation to jartsa but can you please asnwer my question in my post #5? Still not sure about it.
Don't you mean that the ratio of f1 and f2 is that formula? The wiki says the following:
View attachment 108371

I think you're getting the various frequencies mixed up. Let me enumerate them:

$f_{saa}$: the frequency at which Alice sends signals, as measured by Alice's clocks.
$f_{rba}$: the frequency at which Bob receives signals, as measured by Alice's clocks.
$f_{rbb}$: the frequency at which Bob receives signals, as measured by Bob's clocks.
$f_{sba}$: the frequency at which Bob sends signals, as measured by Alice's clocks.
$f_{raa}$: the frequency at which Alice receives signals, as measured by Alice's clocks.
$f_{rab}$: the frequency at which Alice receives signals, as measured by Bob's clocks.

Then if Bob is receding away from Alice,

$\frac{f_{rbb}}{f_{saa}} = \frac{f_{raa}}{f_{sbb}} = \frac{\sqrt{1-\frac{v}{c}}}{\sqrt{1+\frac{v}{c}}}$

In my derivation, $f_{saa} = f_{sbb} = 1$, and $f_{rbb} = f_1'$ and $f_{raa} = f_2'$, so this becomes:

$f_1' = f_2' = \frac{\sqrt{1-\frac{v}{c}}}{\sqrt{1+\frac{v}{c}}}$

 

[JohnnyGui]

I think you're getting the various frequencies mixed up. Let me enumerate them:

$f_{saa}$: the frequency at which Alice sends signals, as measured by Alice's clocks.
$f_{rba}$: the frequency at which Bob receives signals, as measured by Alice's clocks.
$f_{rbb}$: the frequency at which Bob receives signals, as measured by Bob's clocks.
$f_{sba}$: the frequency at which Bob sends signals, as measured by Alice's clocks.
$f_{raa}$: the frequency at which Alice receives signals, as measured by Alice's clocks.
$f_{rab}$: the frequency at which Alice receives signals, as measured by Bob's clocks.

Then if Bob is receding away from Alice,

$\frac{f_{rbb}}{f_{saa}} = \frac{f_{raa}}{f_{sbb}} = \frac{\sqrt{1-\frac{v}{c}}}{\sqrt{1+\frac{v}{c}}}$

In my derivation, $f_{saa} = f_{sbb} = 1$, and $f_{rbb} = f_1'$ and $f_{raa} = f_2'$, so this becomes:

$f_1' = f_2' = \frac{\sqrt{1-\frac{v}{c}}}{\sqrt{1+\frac{v}{c}}}$

Ah, ofcourse. I skipped the part that said the original frequencies are equal to 1.

After reading all this, I've drawn a conclusion for myself (I tend to do that a lot) about the scenario of Alice standing still while sending a frequency while Bob is moving away from Alice and receiving Alice's frequency.

I've concluded the following:

Could you please verify my conclusion being correct or not?

 

[stevendaryl]

Ah, ofcourse. I skipped the part that said the original frequencies are equal to 1.

After reading all this, I've drawn a conclusion for myself (I tend to do that a lot) about the scenario of Alice standing still while sending a frequency while Bob is moving away from Alice and receiving Alice's frequency.

I've concluded the following:
View attachment 108383Could you please verify my conclusion being correct or not?

I think so.

 

[JohnnyGui]

I think so.

Sorry for bumping up my old thread but after looking at the SR rule that is implemented in the Doppler shift, a question came up in me about Special Relativity.

As we've discussed, before SR is applied to the Doppler shift formula, Bob who is receding would say that:

While Alice would say:

De factor between the two f_B's is as concluded, y², which shows that:

Here's my question: Why isn't it possible that Bob would see Alice's clock go faster by a factor of y² instead? The formula clearly shows that, to make them both agree on f_B , Alice's clock could also get corrected by making it faster by a factor of y².

I understand that in SR, both would say that the other is moving. But why can't Bob for example agree that he's the one moving and thus see Alice's clock moving faster by a factor of y² to make them both agree on f_B?

 

[Nugatory]

SBut why can't Bob for example agree that he's the one moving and thus see Alice's clock moving faster?

The clock that is not moving relative to the observer is always the fastest according to that observer - and Bob's clock is not moving relative to Bob.

You may want to try working this out using the Lorentz transformations instead of the time dilation formula (this is often a good approach because the time dilation formula is derived from the Lorentz transformations, so by doing this you're going back to first principles).

Suppose that Alice's and Bob's clock are standing side by side; they both set their clocks to zero and then start moving apart at .6c.

1) Using the frame in which Bob is at rest, what does Alice's clock read at the same time that Bob's clock reads 30 seconds and Alice is 18 light-seconds distant? What does he conclude about the speed of her clock relative to his, given that his clock measured that 30 seconds passed since they separated?
2) Using the frame in which Alice is at rest, what does Bob's clock read at the same time that Alice's clock reads 30 seconds and Bob is 18 light-seconds distant? What does she conclude about the speed of his clock relative to hers, given that her clock measured that 30 seconds passed since they separated?
3) Using the frame in which Bob is at rest, what does Bob's clock read at the same time that Alice's clock reads 30 seconds and she is 18 light-seconds distant?

 

[JohnnyGui]

Post

Thanks for your answer. After reading your questions I come to realize that my problem is more why the Lorentz transformations should be based on both observers thinking that they’re standing still to come to an agreement, while my above mentioned formula shows that this agreement can be achieved by another way.

I really want to get this from the relativistic Doppler shift because I noticed something peculiar from it. I happen to calculate the same difference in Doppler asymmetry by saying that Bob is agreeing that he’s moving and not Alice. Please bear with me:

So Alice is shooting laser signals with a time period of P between them and she finds herself standing still. Imagine that Bob is agreeing that he’s moving away from Alice and that she’s standing still. Yet Bob still measures the lasersignals from Alice approaching him at c. This means that, the time period P’ that Bob would calculate for himself would be:

Since Bob is agreeing that he’s moving away from Alice and he’s measuring the light signals approaching him at c, Bob would have to conclude that Alice must be measuring her light signals having a speed of Bob’s own velocity plus c; so v + c.
So Bob would think that Alice must be calculating Bob’s P’ by using the formula:

So this formula is what Alice should use according to Bob. If you simplify this formula, you would see that this formula is the same as:

Which is the same formula for the case if Bob thinks that he’s the one who’s standing still and Alice is moving. I think that’s the reason why this whole SR rule is created about observers thinking they’re the ones standing still. But as you see, the same formula can be concluded if Bob is agreeing that he’s moving if he thinks that Alice is measuring her light signals are moving at (v + c).

However, for Alice herself, the speed of (v + c) is not true; she also measures her light signals leaving at c. So, she’s actually using the same initial formula that Bob is using for himself, to calculate P’. But this would lead to a contradiction because this would lead to a different P’, which can only be solved if time itself is different among the two.

This leads to my problematic step. Because Bob expects Alice to calculate his P’ way using the speed (v +c) for the light signals, that means Alice’s own P must be decreased by a factor. The factor being the difference/ratio between the formula with the (v + c) as signal speed and the formula with c as the signal speed, which is:

Multiplying this factor with Alice’s P would make her P smaller, thus making her clock go faster with the inverse of that factor. This is what Bob would see on Alice’s clock to make them both agree on Bob’s P’.

Why exactly can’t it be done like this? I have concluded the same formula according tot he SR rule that Bob is thinking that he’s standing still, but by saying that he’s agreeing that he’s moving.