- #1
Rasmus Nielsen
- 5
- 0
I collected this data by moving a sound level meter slowly through a total length of 0,75 meter between two speakers pointing towards each other. The wavelength of the sine waves was 0,343 meters and the frequency was 1000 Hz. The sound level meter measured the sound in dbA 20 times a second.
Can anyone explain why there's no constructive interference at all?
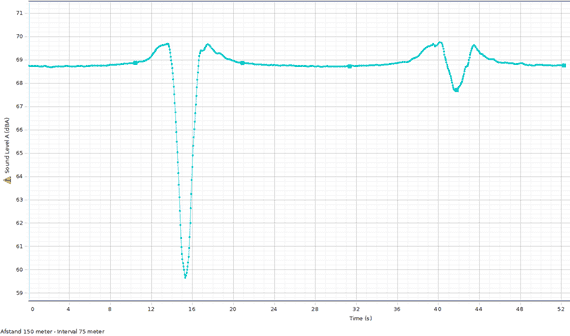
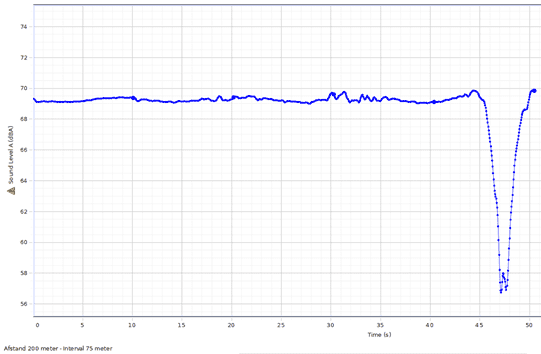
Can anyone explain why there's no constructive interference at all?
Attachments
Last edited: