benagastov
- 8
- 0
- Homework Statement
- It is known that a hypothetical atom emits light when it transitions from an excited state to a ground state with a frequency w0 = 8 x 10^14 Hz. It is known that the observer receives the frequency of these photons due to the Doppler effect.
Get the intensity of the photon spectrum!
- Relevant Equations
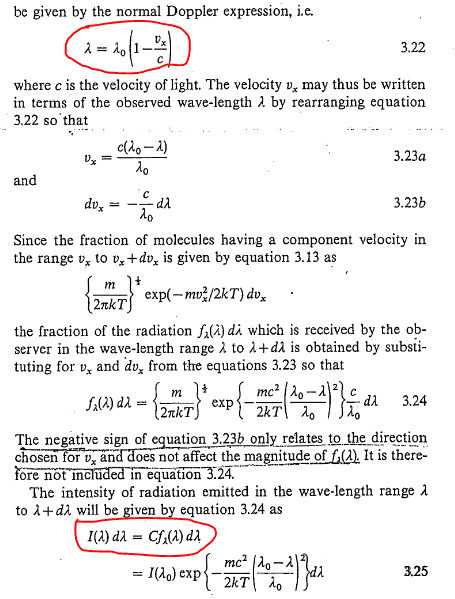
- \omega \:=\:\omega _0\cdot \left(1\:\pm \frac{v_x}{c}\right)
w = w0 (1 + vx/c)
I found there is kind of solution in Pointon's book: An Introduction to Statistical Physics for Students. But I don't know how to find intensity by using frequency.
Attachments
Last edited:


