Speed of a car rolling down a hill

[Thecla]

TL;DR Summary

Will a car make it to the bottom of a long hill?

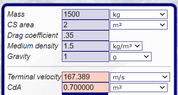
This looks like a physics question but it's not; it's an automotive question. Suppose you had a car at the top of a gently sloped hill, a 5% grade. You start it and put it in neutral or drive and it starts to roll down. You never place your foot on the brake or the accelerator and suppose the hill is 200 miles long.
Will the maximum speed be at the bottom of the hill or will it reach a maximum speed half way down or earlier after a few minutes of travel?
Are there mechanical features of the car that will prevent the car from gaining speed after a certain point?
Will there be a mechanical failure at some point that will terminate the trip like blown tires not designed for high speeds?

 

[jrmichler]

Start by searching car rolling resistance and car aerodynamic drag. Those searches should answer those of your questions related to maximum speed reached by the vehicle. Then make a free body diagram at the start, calculate acceleration vs speed, and calculate how far it travels until it reaches maximum speed. Compare to your 200 miles.

Something to think about if you are planning a car trip through the mountains. Most mountain grades are 5% to 7%, although a few are steeper.

 

[berkeman]

Summary:: Will a car make it to the bottom of a long hill?

You start it and put it in neutral

Why start it? Just put it in neutral and release the parking brake.

Summary:: Will a car make it to the bottom of a long hill?

Will the maximum speed be at the bottom of the hill or will it reach a maximum speed half way down or earlier after a few minutes of travel?
Are there mechanical features of the car that will prevent the car from gaining speed after a certain point?

This is a very underconstrained question. What tires and tire pressures? Which car and what are its wind drag characteristics? Are the windows rolled down? Do you have a roof rack? Are there any deer crossing the road?

This borders on a silly question, but let's give you one more chance to make this a good thread start...

 

[hutchphd]

Some of the OP questions can be answered immediately. OP proposes that the car is on a 5% 200 mile long grade (that's a 10 mile high mountain you realize...but OK). If it starts rolling it will continue all the way down so long as it doesn't fly apart. The details depend upon the rolling resistance, the air resistance, the mass of the car and its maximum viable speed. The calculation is not really very difficult after you specify these things..

Oh yeah and the deer and/or moose population as @berkeman mentioned

 

[Baluncore]

Summary:: Will a car make it to the bottom of a long hill?

This looks like a physics question but it's not; it's an automotive question.

But this is a physics question.
You start at the top of a hill with potential energy; PE = m·g·h
With time PE is converted to kinetic energy; KE = ½·m·v²

Terminal velocity will be reached when the force due to the 'down slope' is equal to the drag of wind resistance and mechanical friction.

 

[jbriggs444]

Terminal velocity will be reached when the force due to the 'down slope' is equal to the drag of wind resistance and mechanical friction.

Neither of which are constant over the course of the trip. Atmospheric pressure is increasing, tire gauge pressure is increasing at first and decreasing later.

Do we want to include the fact that gravity is likely stronger at the bottom of the hill than at the top?

 

[russ_watters]

Neither of which are constant over the course of the trip. Atmospheric pressure is increasing, tire gauge pressure is increasing at first and decreasing later.

Do we want to include the fact that gravity is likely stronger at the bottom of the hill than at the top?

If we're not using major simplifying assumptions then it becomes a pretty daunting civil/structural engineering problem too, given that it's a 52,000 foot "hill". And maybe mechanical/aerospace, because we have to figure out how to get the car to the top of a hill in the stratosphere. And build a car with a pressurized cabin. Economics of all of that?

To me, the OP reads as if it's an arbitrarily long hill for the purpose of making the length irrelevant. The answer (terminal velocity is reached) is the same for a 20 mile hill and maybe even a 2 mile hill. For that short of a hill, you can pick that atmospheric density and assume it's constant over that length.

We can make any problem really complicated if we want (we are really good at it here), but I'd rather push an OP toward simpler to start unless the problem truly demands complexity.

I'll note that the OP isn't back yet...

 

[Baluncore]

Do we want to include the fact that gravity is likely stronger at the bottom of the hill than at the top?

No, because when you are half way down the hill you are being pulled up by half the hill and not being pulled down by the valley. While at the bottom of the hill in the valley, you are being pulled up by the hills on either side.

 

[russ_watters]

Ok, let's try this as a data point for a model:
I've previously calculated that for a car traveling 60 mph (88 fps) and getting a pretty normal fuel economy of 30 mpg, its engine is putting out 17 horsepower. Arbitrarily I'm going to guess 25% rolling resistance, 25% drivetrain loss and 50% aerodynamic drag/actual propulsive force. That's 53 lb of drag/propulsive force.

If the car weighs 4,000 lb, sitting on a 5% grade is 200 lb of force along the incline, minus 50 for rolling resistance (in neutral, so drivetrain loss is assumed zero), so 150 lb of force accelerating the car, for an initial acceleration of 1.21 fps/s. Vs our 53 lb of force at 60 mph, that's a top/terminal speed of 101 mph.

Throwing that into a spreadsheet, I get 13.1 miles (along the slope) to terminal velocity (at 99% of terminal velocity). That's more than I expected, but still a much more manageable 3,500 ft hill.

Certainly these numbers can be refined and will vary based on weight/drag.

[Edit]
That top speed seems high. Back-checking, gravity is providing 54 horsepower at that speed. Using the 25% drivetrain loss assumption, that's 72 horsepower at the engine. I had an Eagle Talon with a ridiculous 92 hp engine that still did about 115 mph, so that's actually right in the ballpark.

...I did have a little error, calculating 48 lb instead of 53 lb of force at 60mph for the example case. Corrected.

 

[anorlunda]

Throwing that into a spreadsheet

Good job Russ.

In my day we used to say "back of the envelope estimate" but I just realized how much that dates me. Nowadays. people have spreadsheets at hand but they don't have used envelopes from the waste paper basket, because they don't use snail mail. They probably don't even say "waste paper basket" any more. They probably don't even use real baskets for trash.

It's not easy getting old.

 

[russ_watters]

In my day we used to say "back of the envelope estimate" but I just realized how much that dates me. Nowadays. people have spreadsheets at hand but they don't have used envelopes from the waste paper basket, because they don't use snail mail.

There were also two post-it notes involved.

 

[bob012345]

[Edit]
That top speed seems high. Back-checking, gravity is providing 54 horsepower at that speed. Using the 25% drivetrain loss assumption, that's 72 horsepower at the engine. I had an Eagle Talon with a ridiculous 92 hp engine that still did about 115 mph, so that's actually right in the ballpark.

...I did have a little error, calculating 48 lb instead of 53 lb of force at 60mph for the example case. Corrected.

I would be curious to see what the acceleration vs. time curve looked like and if the velocity vs. time curve looks like a damped oscillation?

 

[russ_watters]

What was the terminal velocity under your assumptions and what does the curve of acceleration vs. time look like?

Note the slight correction. What you quoted says terminal velocity of 106 mph but I re-checked, found a little error and corrected to 101. Graph (note, the acceleration is x10 for visibility):

 

[Baluncore]

It's not easy getting old.

Which is why I did the experiment when I was young.
I initially accelerated down the hill to what I thought would be terminal velocity, then put it in neutral to see if it would speed up or slow down. For that hill, at 2 AM on a Sunday morning, 120 mph was about right. I then realized that avoiding animals crossing the road was more difficult at higher speeds. I drove on home more sedately and wiser. Next day I measured the panel positions on the left and right hand sides of the car. The panels on the left were all moved backwards by 15 mm. I assume the kangaroo died instantly. They certainly don't make cars like that anymore.

 

[hutchphd]

would be curious to see what the acceleration vs. time curve looked like and if the velocity vs. time curve looks like a damped oscillation?

I see nothing on my envelope that would cause oscillation. Hopefully there are none in @russ_watters spreadsheet result.

 

[bob012345]

I see nothing on my envelope that would cause oscillation. Hopefully there are none in @russ_watters spreadsheet result.

Can it be generally shown that you would need an oscillatory driving force to get any oscillations no matter how non-linear the other forces were? I was just thinking a spring force is ~$-k x^2$ and the air resistance here is ~$-kv^2$.

 

[hutchphd]

I will leave to more knowledgeable folks the general question for arbitrary nonlinear systems.
The case of $v^2$ drag is soluble directly and gives no oscillations

 

[jack action]

The governing equation (inertia = gravity - rolling resistance - drag):
$$ma = mg\sin\theta - f_r mg\cos\theta -\frac{1}{2}\rho C_d Av^2$$
Terminal velocity is (at $a=0$):
$$v = \sqrt{\frac{2mg(\sin\theta - f_r \cos\theta)}{\rho C_d A}}$$
We already notice that the coefficient of rolling resistance $f_r$ must be smaller than $\tan\theta$ (or the hill grade) for the terminal velocity to be greater than zero. For typical values of $m = 1800 kg$ ,$fr=0.013$ and $\rho C_d A = 0.9 kg/m$, we get a terminal velocity of 44 m/s or 159 km/h.

As for the distance $x$, assuming the average acceleration $\bar{a}$ is half^* the maximum acceleration (at the top of the hill, when $v=0$), we can estimate it with:
$$x = \frac{v^2}{2\bar{a}}$$
or:
$$x = \frac{\frac{2mg(\sin\theta - f_r \cos\theta)}{\rho C_d A}}{2\frac{g(\sin\theta - f_r \cos\theta)}{2}}$$
$$x = \frac{2m}{\rho C_d A}$$
So funny thing, our car will take a few clicks over 2 km to reach its terminal velocity, no matter the grade of the hill, no matter the rolling resistance.

---

* Actually it will be a little bit smaller than half, probably around 40% of the maximum acceleration.

 

[hutchphd]

I think the exact result about terminal velocity distance represents mostly the assumption you make about the average acceleration. Two things come to mind:

1. the car never actually reaches terminal velocity, so one needs caution
2. for a fixed angle hill, the effect of $f_r$ is simply to lessen the effective g (look at original eqn.) so it should not be a surprise that it falls out

 

[jbriggs444]

No, because when you are half way down the hill you are being pulled up by half the hill and not being pulled down by the valley. While at the bottom of the hill in the valley, you are being pulled up by the hills on either side.

That's not correct. https://en.wikipedia.org/wiki/Gravity_of_Earth#/media/File:EarthGravityPREM.svg

The dominant effect is increasing proximity to the dense core.

 

[hutchphd]

The dominant effect is increasing proximity to the dense core.

Can you be a little more specific as to where in the reference this argument obtains? It is not obvious to me which part wins here.

 

[jbriggs444]

Can you be a little more specific as to where in the reference his argument obtains? It is not obvious to me which part wins here.

The section on depth in the wikipedia article considers a much more extreme case. A test object subject to gravity within an ideal near-spherical Earth, the "Preliminary Reference Earth Model" or PREM for short. Instead of mere hillsides overhead, one has solid rock overhead. And yet gravity still gets stronger as you go deeper.

So the proximity to the core wins -- at depths near the surface.

Though I will concede that with Osmium hillsides the local result could deviate from the average.

 

[Baluncore]

Instead of mere hillsides overhead, one has solid rock overhead. And yet gravity gets stronger as you go deeper.

The solid rock overhead is part of a perfect spherical shell, that is exactly canceled by that same spherical shell of material over the rest of the sphere beneath your feet.
That does not hold when there are local hills and valleys, where g is reduced because the Earth is not a perfect sphere and the hills are not complete spherical shells.

 

[jbriggs444]

The solid rock overhead is part of a perfect spherical shell, that is exactly canceled by that same spherical shell of material over the rest of the sphere beneath your feet.
That does not hold when there are local hills and valleys, where g is reduced because the Earth is not a perfect sphere and the hills are not complete spherical shells.

Edit: possibly you have it correct. I am no longer sure of my reasoning in the case of a local hill sides to right and left which win on proximity.

 

[Dullard]

"I see nothing on my envelope that would cause oscillation. Hopefully there are none in @russ_watters spreadsheet result."

You are obviously ignoring the dog. All dogs have a preferred head-out-the-window speed range.

 

[hutchphd]

Neither argument here is definitive IMHO.
I believe in the Cavendish balance and the presence of a nearby isolated tall mountain can certainly reduce the local g. The devil is of course in the detail of the Osmium hills and nearby Tungsten cliffs.

 

[russ_watters]

You are obviously ignoring the dog. All dogs have a preferred head-out-the-window speed range.

I smell a research opportunity: "Canis Familiaris Glazing Retracted Pulse-Width Modulated Automobile Velocity Control"

 

[Baluncore]

Neither argument here is definitive IMHO.

I agree.

One of the corrections done during regional gravity surveys is to allow for the reduction in g observed due to the local topography.

 

[bob012345]

Neither argument here is definitive IMHO.
I believe in the Cavendish balance and the presence of a nearby isolated tall mountain can certainly reduce the local g. The devil is of course in the detail of the Osmium hills and nearby Tungsten cliffs.

The OP concerned a hill rising above the earth. We should assume a perfectly spherical Earth with only that hill on it breaking the symmetry but need we to agree on what the hill density, size and shape is. A cone with a 5% grade, a 200 mile long edge and the average density of the Earth would be 'simple'.

 

[Dullard]

I smell a research opportunity: "Canis Familiaris Glazing Retracted Pulse-Width Modulated Automobile Velocity Control"

It has "NASA SBIR" written all over it.

 

[Thecla]

I wanted the hill to be long enough to reach a terminal velocity not at the bottom of a hill. If a 20 mile hill is long enough that will be fine. The car by the way is a normal sedan with standard tires

 

[bob012345]

I wanted the hill to be long enough to reach a terminal velocity not at the bottom of a hill. If a 20 mile hill is long enough that will be fine. The car by the way is a normal sedan with standard tires

Great. That puts it in the range of a real mountain in principle.

 

[Thecla]

By the way would you feel safe in a car free rolling down a 30 degree slope that is 20 miles long? Would you worry the car will fall apart?

 

[jrmichler]

This physics problem can benefit from some real data. A coastdown test on my truck resulted in the drag equation: Drag = 54 lbs + 0.0143 X (ft/sec)^2. The test procedure and calculations are here: https://ecomodder.com/forum/showthread.php/coastdown-test-06-gmc-canyon-20405.html. That coastdown test was done at 17 deg F outside air temperature. The drag would be a lot less at warmer temperatures.

At terminal velocity on a 5% grade, the total drag will be 0.05 X 4650 = 233 lbs. Solving for the velocity at which total drag = 233 lbs, the terminal velocity would be 112 ft/sec = 76 MPH.

Calculating the terminal velocity for a 30 degree slope is left as an exercise for others.

 

[bob012345]

By the way would you feel safe in a car free rolling down a 30 degree slope that is 20 miles long? Would you worry the car will fall apart?

No, I'd worry about me falling apart first.

Also, you asked;

Are there mechanical features of the car that will prevent the car from gaining speed after a certain point?

The engine will act like a brake and limit the speed to some extent if you downshift or with an automatic, shift to Low depending on the circumstances. I could envision a situation where the acceleration due to gravity is matched by the engine braking and rolling friction even without aerodynamic drag for a gentle slope.