Splitting Fields: Anderson and Feil, Theorem 45.4 ....

[Math Amateur]

I am reading Anderson and Feil - A First Course in Abstract Algebra.

I am currently focused on Ch. 45: The Splitting Field ... ...

I need some help with some aspects of the proof of Theorem 45.4 ...

Theorem 45.4 and its proof read as follows:

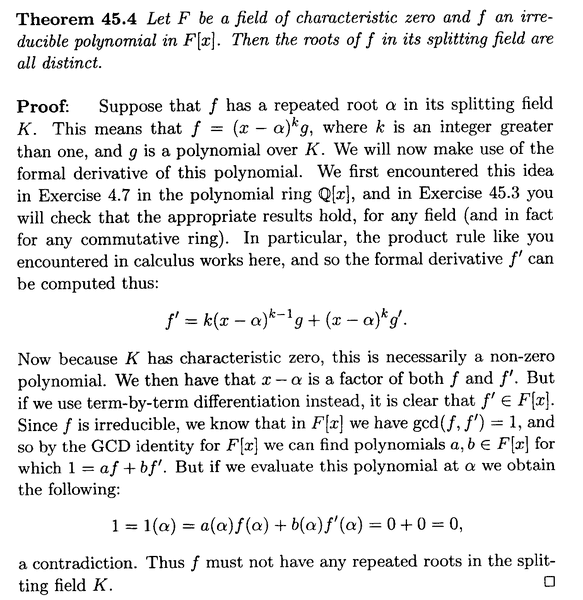
My questions on the above proof are as follows:Question 1In the above text from Anderson and Feil we read the following:"... ... This means that $f = ( x - \alpha)^k g$, where $k$ is an integer greater than $1$ and $g$ is a polynomial over $K$ ... ... Since $f$ is in $F[x]$ ... that is $f$ is over $F$ ... shouldn't $g$ be over $F$ not $K$?

(I am assuming that $f$ being "over $F$" means the coefficients of $f$ are in $F$ ... )

Question 2In the above text from Anderson and Feil we read the following:"... ... We then have that $x - \alpha$ is a factor of both $f$ and $f'$. But if we use term-by-term differentiation instead, it is clear that $f'\in F[x]$. ... ... "What do Anderson and Feil mean by term-by-term differentiation in this context ... ... and if they do use term-by-term differentiation (what ever they mean) how does this show that $f'\in F[x]$ ... ... ?
Hope someone can help ...

Help will be much appreciated ... ...

Peter

 

[andrewkirk]

If $\alpha\in K-F$ then $g$ will not necessarily be in $F$ as its coefficients will be functions of $\alpha$.

Consider $F=\mathbb R,\ K=\mathbb C$ and $f(x)=(x^2+1)^2=(x-i)^2(x+i)^2$, which has roots $i$ and $-i$, both of multiplicity 2. Taking $\alpha=i$ we get $g(x)=(x+i)^2$, which is not in $\mathbb R[x]$.

 

[andrewkirk]

"... ... We then have that $x - \alpha$ is a factor of both $f$ and $f'$. But if we use term-by-term differentiation instead, it is clear that $f'\in F[x]$. ... ... "

I was going off to have dinner, thinking I didn't have time to work out the second one. Then I realized it's actually the easier of the two.

By 'term-by-term' they mean writing the polynomial in expanded, unfactorised form as $f(x)=\sum_{k=0}^n c_kx^k$ and then differentiating to get $f'(x)=\sum_{k=1}^n kc_kx^{k-1}$. The coefficients are of the form $kc_k$ which, since $k$ is in $\mathbb N$ rather than $F$, means $\sum_{j=1}^k c_k$, which must be in $F$ since $c_k$ is. Hence $f'\in F[x]$.

 

[Math Amateur]

If $\alpha\in K-F$ then $g$ will not necessarily be in $F$ as its coefficients will be functions of $\alpha$.

Consider $F=\mathbb R,\ K=\mathbb C$ and $f(x)=(x^2+1)^2=(x-i)^2(x+i)^2$, which has roots $i$ and $-i$, both of multiplicity 2. Taking $\alpha=i$ we get $g(x)=(x+i)^2$, which is not in $\mathbb R[x]$.

Thanks Andrew ... good example ... makes it pretty clear ...

Peter