member 731016
- Homework Statement
- I have successfully solved the problem below by assuming that momentum is conserved and that there is an inelastic collision occurring between the masses. However, I am wondering whether the momentum being conserved is a valid assumption that I have made.
- Relevant Equations
- ##E_i = E_f##
##\vec p_i = \vec p_f##
For this problem,
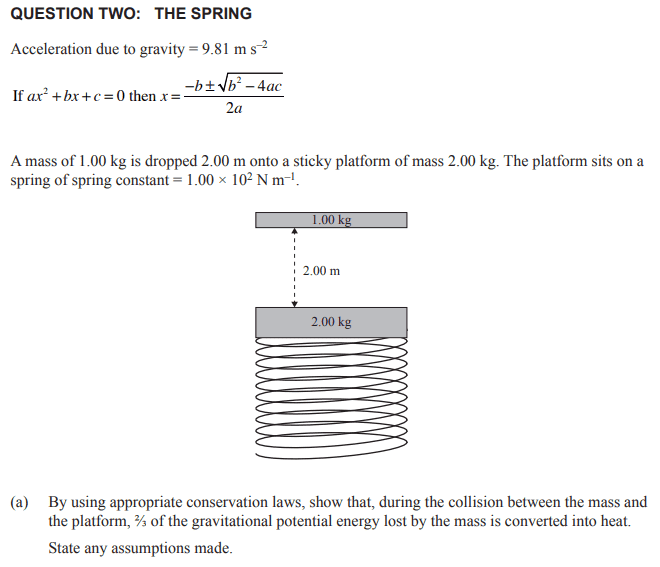
The reason why I am not sure whether it is a valid assumption whether momentum is conserved because during the collision if we consider the two masses to be the system, then there will be a uniform gravitational field acting on both masses, and a spring force that is acting upwards. Therefore, there will be two external forces acting on the system. The only reason I can think of for momentum being conserved in this case is if the forces acting on the both the masses acted over such a short time interval that there was no change in the momentum due to the forces.
However, if we define the system as everything, the two masses, the spring, and the source of the g-field, then I believe everything is internal force pairs so momentum is conserved.
If someone please knows whether momentum is conserved is a valid assumption and why, that would be greatly appreciated!
Many thanks!
The reason why I am not sure whether it is a valid assumption whether momentum is conserved because during the collision if we consider the two masses to be the system, then there will be a uniform gravitational field acting on both masses, and a spring force that is acting upwards. Therefore, there will be two external forces acting on the system. The only reason I can think of for momentum being conserved in this case is if the forces acting on the both the masses acted over such a short time interval that there was no change in the momentum due to the forces.
However, if we define the system as everything, the two masses, the spring, and the source of the g-field, then I believe everything is internal force pairs so momentum is conserved.
If someone please knows whether momentum is conserved is a valid assumption and why, that would be greatly appreciated!
Many thanks!