Standard designation for generalization of Euler-Lagrange?

[nomadreid]

TL;DR Summary

In Wiki's article on the Euler-Lagrange equation , under "Generalizations">'Single function of single variable...', there is an equation (stated in main text). Is there a standard name for it? Some Russian authors call it the Euler-Poisson equation.

In English, does the equation

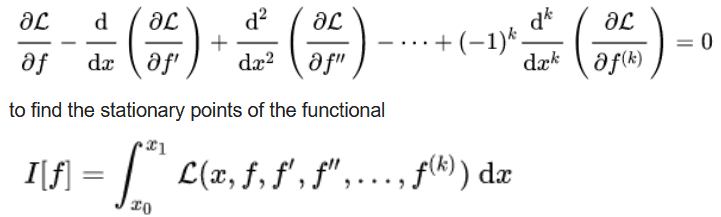
have any standard name besides (generalization of) the Euler-Lagrange Theorem? I have seen the designation "Euler-Poisson Equation" used by the Russian mathematician Lev Elsholtz way back in 1956 repeated in recent Russian webpages, but am not sure whether this would be recognizable (perhaps with a footnote?) by English-speaking mathematicians. (Not to get mixed up with either the Euler-Poisson-Darboux Equation or the Euler-Poisson Integral.)

I was unsure whether to post this in the mathematics or the physics section, as it is strictly speaking mathematics but mainly used in physics. If a moderator wishes to move it, then my thanks in advance to that moderator.

 

[robphy]

In Schouten's Ricci Calculus (Springer) and his Tensor Analysis for Physicists (Dover),
he refers to the "Lagrange Derivative".

previews from Google Books... search for "lagrange derivative"

From Ricci Calculus,

From Tensor Analysis for Physicists,

 

[nomadreid]

Thanks very much, robphy. (Sorry for the delayed response.)

(You attached the same excerpt twice.)

Unfortunately, when I googled "Lagrange derivative", I came up empty (i.e., nothing under that title, and, google sending me what was closest, everything was about the Euler-Lagrange Equation).

I am working through the text you sent. Given that I am close to nil in differential equations, perhaps you can answer a couple of questions on it. First,

Wouldn't that make the Script-L a functional?

I am attempting to see whether this text would help me interpret the so-called Euler-Poisson equation in where the partial derivatives such as

in the Euler-Lagrange equation are replaced by the "total partial derivative", or "complete partial derivative",

which are defined as follows (Elgots, Calculus of Variations)

Is the "complete partial derivative" here the same as the "total derivative"?

For which situation(s) is the replacement of partial derivatives by "total partial derivatives" in the Euler-Lagrange equation useful/valid? It appears that when one applies this new equation to a Lagrangian, one is likely to get a different answer than the application of the usual Euler-Lagrange equation, so the circumstances must be different, no?

Sorry if the question is obvious, but my level of "diffy-Q" is rather basic. Thanks for any help.

​

 

[robphy]

Thanks very much, robphy. (Sorry for the delayed response.)

(You attached the same excerpt twice.)

Hmmm... that's odd. I see 4 distinct images, 2 each from the two works (p.111, 112 from Ricci Calculus, p 78-79, 79-80).

with quotes

• https://www.google.com/search?q="lagrange+derivative"+schouten
  https://scholar.google.com/scholar?&q="lagrange derivative"+schouten
• "Euler-Lagrange operator"
  https://scholar.google.com/scholar?q="Euler-Lagrange+operator"
  e.g. https://de.maplesoft.com/support/he...ifferentialGeometry/JetCalculus/EulerLagrange
  https://encyclopediaofmath.org/wiki/Euler_operator
  
  Natural Variational Principles on Reimannian Manifolds
  Ian M. Anderson
  https://www.jstor.org/stable/2006945?origin=crossref&seq=1#metadata_info_tab_contents
• possibly useful?
  Lagrange geometry of second order
  R.Miron G.Atanasiu
  https://www.sciencedirect.com/science/article/pii/0895717794901554

I've been interested in Schouten's work for a while...
so I recall seeing the "Lagrange derivative"
... and, thus, my response.
Unfortunately, I don't know any more details, except for these terms that may be related to what you seek.

 

[vanhees71]

I know it as the functional derivative: You define
$$S[q]=\int_{t_1}^{t_2} \mathrm{d} t L(q,\dot{q},\ddot{q},\ldots)$$
as a functional on the space of trajectories $q(t)$ with fixed initial and final point. Then via variation and integration by parts you get
$$\delta S=\int_{t_1}^{t_2} \mathrm{d} t (\partial_q L - \mathrm{d}_t \partial_{\dot{q}} L + \mathrm{d}_t^2 \partial_{\ddot{q}} L+\cdots).$$
This defines the functional derivative as
$$\frac{\delta S}{\delta q(t)} = \partial_q L - \mathrm{d}_t \partial_{\dot{q}} L + \mathrm{d}_t^2 \partial_{\ddot{q}} L+\cdots$$

 

[nomadreid]

Thanks very much, robphy and vanhees71. Very helpful!