Static friction and a rotating object

[Biker]

Homework Statement

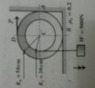
Sorry for the bad resolution on the image but I will clear the variables out.

The friction act on points A and B, Us = 0.2, W = 900 and there is a force P to the right
The question is:
Find the force P that makes the weight move downward with a constant velocity

Homework Equations

Torque around a point.
net F = 0

The Attempt at a Solution

I don't care much about the numbers what bugs me that in the solution, He assumed that the friction force at both A and B is static friction and its magnitude is u N_A and u N_B respectively.

How is it spinning with constant velocity or this is at least what I gathered from the question but have static friction instead of kinetic one?

I can see why it is static friction at point B if it is moving without slipping then the relative velocity of point B to the ground is zero so it is static but that doesn't explain point A

My professor said that it is equivalent to the statement that the object is about to rotate, But clearly it is different.

 

[kuruman]

But clearly it is different.

If the wheel is spinning at constant velocity, you have to use kinetic friction. Having said that, you must use μ_kN for friction. Note the subscript. Can you post the full statement of the problem instead of just the question?

 

[Biker]

If the wheel is spinning at constant velocity, you have to use kinetic friction. Having said that, you must use μ_kN for friction. Note the subscript. Can you post the full statement of the problem instead of just the question?

I translated the question, The full statement is just describing the question so I can draw it.
But The actual question is just asking what P has to be so the weight can move downward with constant velocity

So it is kinetic friction then?

 

[kuruman]

I was asking specifically to see what the meaning of "Us = 0.2" is. Is that exactly what is given in the original question?

 

[Biker]

I was asking specifically to see what the meaning of "Us = 0.2" is. Is that exactly what is given in the original question?

Us is the coefficient of static friction. Yep, I listed everything it said.

 

[kuruman]

So it is kinetic friction then?

Yes, it is kinetic friction. The problem clearly states that the wheel is moving at constant velocity so the surfaces are sliding relative to each other. If Us is the only coefficient that is given, then I suggest that you use it pretending it is the coefficient of kinetic friction.

 

[kuruman]

By the way, is the wheel supposed to be massless?

 

[Biker]

By the way, is the wheel supposed to be massless?

How does that matter? They might have forgotten to mention that.

 

[kuruman]

It matters because it affects the normal force N that the floor exerts on the wheel and hence the kinetic friction μ_kN at that point of contact. If there is no mention of the wheel's mass, then you can only assume that it is massless. Also, can you post the numbers for the two radii? I cannot read them off the figure.

 

[Biker]

It matters because it affects the normal force N that the floor exerts on the wheel and hence the kinetic friction μ_kN at that point of contact. If there is no mention of the wheel's mass, then you can only assume that it is massless. Also, can you post the numbers for the two radii? I cannot read them off the figure.

R2 = 50 cm and R1 = 30 cm

Yes, There is no mention of its mass so it is massless. The problem was with the static friction, Other than that it is just solving equilibrium equations.

 

[kuruman]

Other than that it is just solving equilibrium equations.

Yes, the sum of all the forces is zero and the sum of all the torques is zero because the linear and angular acceleration of the system is zero.
I calculated an answer which you can compare with yours when you get it.

 

[Biker]

I have the answer assuming it is kinetic friction (Book's answer). I was wondering can't it be that it is rolling to the left side with constant angular velocity without slipping? That would mean that we have static friction at the bottom ( not maximum though) and kinetic friction on the side (momentarily). However that makes it 4 unknowns in 3 equation, So we do have an infinite amount of solutions that means that we don't have specific value of P.

Another question with the same idea, These questions bother me a lot. Hopefully you can help me figure why they solve it this way.
https://image.prntscr.com/image/aRnAD33ES2mML5JBwQBQvQ.png

it said in the question that U = 0.6 without saying if it is static or kinetic, However It is static because we are in a static course XD.
It wants P that makes the cylinder about to slip.

Now my explanation for the diagram was, That we could have rolling without slipping ( rotates about B and have maximum static friction at A) but that again makes it 4 unknowns. But he said that he wants the cylinder to be about to slip. So B must have static friction that is maximum and A must have maximum static friction because If for it to move it must overcome that friction. Is this right?

 

[kuruman]

have the answer assuming it is kinetic friction (Book's answer). I was wondering can't it be that it is rolling to the left side with constant angular velocity without slipping? That would mean that we have static friction at the bottom ( not maximum though) and kinetic friction on the side (momentarily).

It can be rolling to the left without slipping at constant velocity for an appropriate value of force P. However, the proper way of solving this is not when there is "kinetic friction on the side (momentarily)" because "constant velocity" is not momentary but extends over a long period of time.

Another question with the same idea, These questions bother me a lot. Hopefully you can help me figure why they solve it this way.

This is a new problem, so please post it on a separate thread and I or someone else should be able to help you.

 

[Biker]

It can be rolling to the left without slipping at constant velocity for an appropriate value of force P. However, the proper way of solving this is not when there is "kinetic friction on the side (momentarily)" because "constant velocity" is not momentary but extends over a long period of time.

This is a new problem, so please post it on a separate thread and I or someone else should be able to help you.

Wouldn't it first have friction on the wall until it moves away from it?

All right I will post it in a different thread.

 

[kuruman]

Wouldn't it first have friction on the wall until it moves away from it?

It would, but as you said that friction is momentary. It can't roll away at constant velocity and maintain the friction on the wall. You want a solution for force P when it is at 1 cm away from the wall or 2 cm away from the wall or any distance away from the wall because that's what constant velocity means. The velocity cannot be instantaneously constant; you don't now that it is constant unless you look at it at two different points in time and ascertain that it is the same. When the wheel is in contact with the wall, its velocity is zero because it hasn't started moving yet.