Statics: A pulley system in equilibrium

[Violagirl]

Homework Statement

Hi,

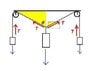
I am confused by this physics question and I was wondering if someone could help me out. I have a system with 2 pulleys and 3 weights. They are all held together by one string, the two weights on the outside of the two pulleys, both weighing the same, hold up the weight in the center.
The system has a central object B with mass (M) which is suspended half way between two pulleys by a string. The whole system is in equilibrium. The objects
A and C, which are on the outside of the pulleys, have the same mass of (m).
What I'm trying to figure out, what is an equation for the vertical displacement of the central object B in terms of the horizontal distance between the two pulleys (L), the mass of object B (M), and the mass (m) of objects A and C?

 

[ehild]

Make a drawing first. Show the data given. Then draw the forces acting on each weight. The system is in equilibrium so the vector sum of forces at each weight has to be zero.

ehild

 

[Violagirl]

In drawing it out, if the masses on A and C are kept constant and the string is all one length, then I assume we'd show distance to be equivalent to L/2, right? When it comes to determining how the angle changes, that's what I find to be difficult in putting it together. If it the mass b (M) is changing, I want to say that L/2 Tan theta = d but I'm not sure where to go from there.

 

[ehild]

Why does anything change when the system is in equilibrium? Nothing moves. Which distance is L/2?

Can you show your picture, or should I show it?

ehild

 

[Violagirl]

Oh yes, that is true, so then the angle would remain consistent. And L/2 corresponds to the string lengths of masses a and c and since the mass will be consistent on both, L can be divided by two to represent both. Attached is my picture for it along with the forces that are significant.

 

[ehild]

Do you need to find how d is related to the masses and to L? Draw the forces at each weight. The tension in the rope is the same everywhere. Do you know its magnitude in terms of m?

ehild

 

[ehild]

Some more hint: See attachment. The yellow triangles are similar, so you can relate the vertical and horizontal components of the tension at P with the angle θ.


ehild

 

[Violagirl]

Thanks for your drawing! So if T is the same everywhere and corresponds to L/2 whereas D is a measure of how much it moves vertically, could we say that D = (L/2) cos θ M/m_a+m_c?

 

[ehild]

Thanks for your drawing! So if T is the same everywhere and corresponds to L/2 whereas D is a measure of how much it moves vertically, could we say that D = (L/2) cos θ M/m_a+m_c?

No. How did you get that equation?
Do not forget that ma=mc=m. You need to express D with M, m and L. cos(theta) can be expressed with the masses.

ehild

 

[Violagirl]

Oh shoot, you're right, nevermind!

In going through my notes, I did find an equation relating to a sample problem like this for what we did in my lab for class. The equation was given as L/2 tan θ = D.

Next, a picture is shown with an inclined plane with 2m on the hypotenuse, M on the side (y axis of triangle), and √(2m)²-m².

I have no idea if it's supposed to correlate in conjunction to this problem or not however...Thank you for your input again!

 

[ehild]

The forces have to cancel at point P. The tension in the rope attached to B is Mg.
The tension of the long rope is the same everywhere. To keep the objects A and C in equilibrium, it must be T=mg. So you have tree forces, Mg vertically down, and two upward forces of magnitude T, at angle theta to the vertical. The resultant of the two forces of magnitude T=mg is the blue vector and it has to cancel the downward force Mg.

Spoiler

_{The magnitude of the blue vector is 2mg cos(θ)}

ehild

 

[Violagirl]

Oh! Ok, got it, that makes sense now. So since we have a point, P, in our system and the pulley attached to mass B can be considered a lever, we are looking at an overall equation of T (torque) = F * D sin θ.

Our forces in this situation are T and mg. Because the masses of A and C are equivalent to one another, we have a total force of 2T which equals 2 mg as they are opposite and the system is in equilibrium. The angle at which mass B is lifted is at an angle of cos theta. And our distance in this situation is equivalent to 2L from what it had looked like in drawing it out. Would the overall equation then be:τ = 2mg*2L cos θ?

Otherwise, my professor had also mentioned to talk about the derivation of the formula in hinting that it be important for our next exam. If I were to take the integral for it, wouldn't it be:

τ= mg²*L²sin θ?

Thanks again for your input, it has really helped me understand this better!

 

[ehild]

NO, I did not mean that! You have three forces acting at the point P and their vector sum must be zero. You can add forces by adding their components. The horizontal components of the tensions along the upper rope are Tsin(θ) and -Tsin(θ): they cancel, so the vector sum of the tensions in the pieces of the upper rope has only vertical component, the sum of the vertical components of the tensions in both upward forces. The vertical components of both tension forces are Tcos(θ), they add, so the resultant is 2Tcos(θ) (the blue force). That is opposite and equal to the downward force Mg, So 2Tcos(θ)-Mg=0. You know that T=mg, so 2mgcos(θ)=Mg, cos(θ)=M/(2m). You also know that tan(θ)=L/(2d). Use the relation between tangent and cosine: \tan(\theta)=\sqrt{\frac{1}{1+\cos^2(\theta)}}

ehild

 

[Violagirl]

Echild, thank you so much! I really appreciate your help, it does make sense now. My final question, in relating tan θ to √1/1+cos²(θ), did you use the Trig identity,

tan²θ+1 = sec²(θ)?

 

[ehild]

Echild, thank you so much! I really appreciate your help, it does make sense now. My final question, in relating tan θ to √1/1+cos²(θ), did you use the Trig identity,

tan²θ+1 = sec²(θ)?

Yes, it is the correct relation, I mistakenly exchanged cosine and tangent. It should have been \cos(\theta)=\sqrt{\frac{1}{1+\tan^2(\theta)}}

ehild