Stimulated emission/absorption - which equations act on external targets?

[JD23]

TL;DR Summary

Both equations act on target inside laser - which ones act on external targets?
Could population inversion be achieved by shooting with (ring) laser?

Stimulated emission-absorption have two symmetric equations for population (N_1 - number of ground state atoms, N_2 - of excited) written below, at heart of lasers: https://en.wikipedia.org/wiki/Stimulated_emission#Mathematical_model
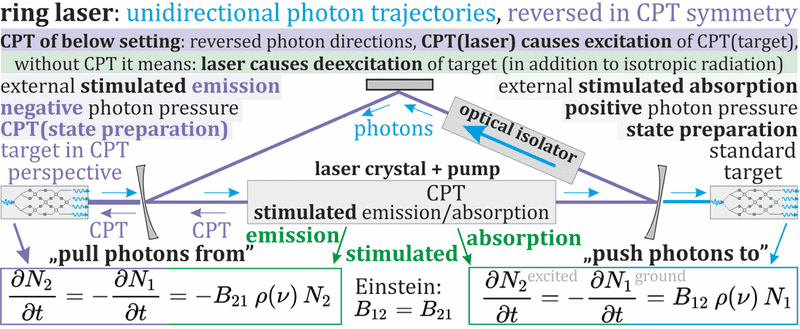
We assume that both equations act on the central target inside laser - but what about external laser's targets? (with frequencies in agreement)

Standard laser's target absorbs produced photons, increasing the number of its excited atoms (N_2) - like in the equation on the right.
But what about the second equation (on the left)? - if it also acts, the maximal achievable excitation level by shooting with laser should be N_1 = N_2, is it?

Then there are ring lasers - using optical isolator to enforce unidirectional photon trajectories - thanks to e.g. Faraday effect: difference in propagation speed for two circular polarizations (they would switch after T transform - this material violates T symmetry).
For them it seems equation on the right acts only on target on the right?
If so, they would allow for very high excitation levels for external target: N_2 > N_1 for target (population inversion, if overcoming spontaneous emission)?
And what about target on the left? Looking from perspective after T or CPT symmetry, it would become the standard target - for equation on the right, so in standard perspective shouldn't equation on the left act on target on the left?

Could population inversion be achieved by shooting with (ring) laser?

 

[JD23]

I have another argument suggesting the stimulated emission equation should act on external target on the left - adding mirrors to enclose a detour, it becomes internal target, hence both equations should act on it:

"Which of 2 equations act on external targets?" seems extremely important fundamental question ... looks like nobody knows answer to (?) - for months I have searched literature, asked on forums, through private communication ...

Here are some experimental test examples - maybe some of you could perform (for important article, I would gladly collaborate):

1) For ring laser (with optical isolator), the question is if stimulated emission acts on external target on the left. To test it, this target needs to be continuously excited e.g. by external pump (the same frequency). Monitoring its population level, opening the shutter toward laser, the question is if it would increase deexcitation rate?

2) For standard laser (no optical isolator) both equations should act on external targets - allowing to reach at most N_2 = N_1 population level for two-state systems (for ring laser it could be higher) ... and increasing deexcitation rate in case of target's population inversion N_2 > N_1.

 

[JD23]

Thinking about stimulated emission-absorption as CPT analogs: negative-positive radiation pressure, below is looking similar hydrodynamical situation with pump - can we think this way?

It brings another test proposal - for pump negative radiation pressure should reduce flow down the shown split - it would be great testing if light intensity down the beam splitter also changes by opening/closing shutter toward left?