Stress, strain and deflections due to temperature change

[Dell]

in the following problem

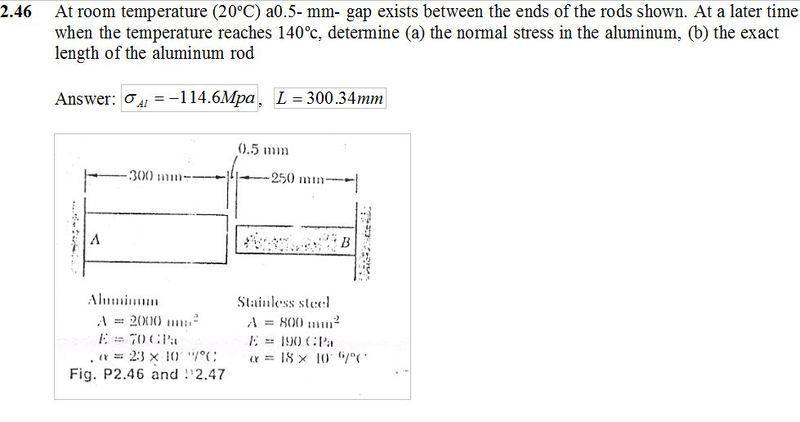
i know that ΔT=120

now the maximum deflection is 0.5mm so i looked for the total deflection had there been no restrictions

ΔL(aluminium)=300*(23e-6*120)= 0.8280
ΔL(st steel)=250*(18e-6*120)= 0.5400

this is clearly more than the maximum deflection of 0.5- there are 0.868 "extra" which cause the stress.

now putting this all together is where i get stumped.


F=ε*E*A
and ΔL=ε*L

F(aluminium)=ε(al)*(70e9)*(2000)
F(steel)=ε(s)*(190e9)*(800)
ε(al)*300+ε(s)*250=0.5

now the force in the aluminium and in the steel must be equal so i have 3 equation system to solve, after solving i get

F= 1.3201e+011
ε(al)= 0.942928e-3
ε(s)=0.868486e-3

now simply using

σ=ε*E or σ=F/A

σ=ε*E
=0.942928e-3*70e9
=66004960

but the correct answer is -114.6MPa

i can see where this might be wrong, nowhere in my stress calculations do i take into account the amount that each material expands. but i have no idea how to fix it

 

[Dell]

think i got it,

F(aluminium)=ε(al)*(70e9)*(2000)
F(steel)=ε(s)*(190e9)*(800)
ε(al)*300+ε(s)*250=0.868

 

[Mene]

I would like to first clarify that stress, strain, and deflection are all related but different concepts. Stress is the force per unit area that a material experiences, while strain is the deformation or change in shape of a material due to the applied stress. Deflection, on the other hand, is the displacement or movement of a material due to an external force or load.

In this problem, we are dealing with stress, strain, and deflection due to temperature change. When a material is subjected to a change in temperature, it expands or contracts, causing stress and strain. The amount of deflection that occurs depends on the material's properties, such as its coefficient of thermal expansion.

In order to solve this problem, we need to consider the thermal expansion of both materials, as well as the force and area of each material. We also need to consider the relationship between stress and strain, which is given by the material's modulus of elasticity (E).

To begin, we can calculate the total thermal expansion of each material using the given temperature change (ΔT=120) and the material's coefficient of thermal expansion. This will give us the total change in length (ΔL) for each material.

ΔL(aluminium) = 300 * (23e-6 * 120) = 0.828 mm
ΔL(stainless steel) = 250 * (18e-6 * 120) = 0.54 mm

Next, we need to calculate the strain for each material using the formula ΔL/L, where L is the original length of the material. This will give us the amount of deformation for each material.

ε(aluminium) = 0.828 mm / 300 mm = 2.76e-3
ε(stainless steel) = 0.54 mm / 250 mm = 2.16e-3

Now, we can use the relationship between stress and strain to calculate the stress in each material. Recall that stress is equal to E * strain, where E is the modulus of elasticity for the material.

σ(aluminium) = 2.76e-3 * 70e9 = 193.2 MPa
σ(stainless steel) = 2.16e-3 * 190e9 = 410.4 MPa

We can see that the stress in the aluminium is lower than the maximum allowable stress of 114.